Matematika SMA - Vektor (5) - Vektor Dimensi 3, Operasi Vektor Dimensi 3 (Y)

Summary

In this educational video, the host explains vector operations in three-dimensional space. The lesson covers key concepts such as unit vectors, position vectors, and calculating vector magnitudes using the Pythagorean theorem. The video also dives into vector addition, subtraction, scalar multiplication, and provides clear, step-by-step examples to help viewers understand these operations. Practical exercises and problem-solving examples are included, making it an excellent resource for anyone learning vector algebra and its application in 3D space.

Takeaways

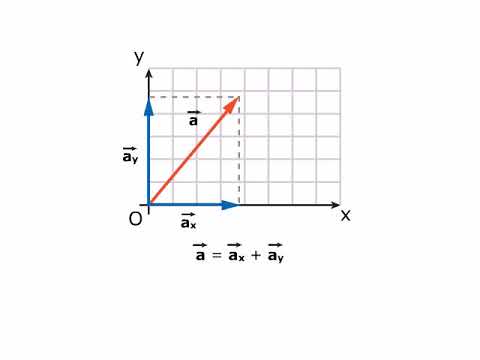
• 😀 The video explains vectors in 3D space, starting with a review of Cartesian coordinates and unit vectors (i, j, k) in 3D.
• 😀 In 3D, vectors are represented by three components: along the x, y, and z axes. Unit vectors in these directions are i (100), j (010), and k (001).
• 😀 The length (magnitude) of a vector in 3D is calculated using the formula √(a1² + a2² + a3²), where a1, a2, and a3 are the vector components.
• 😀 The video demonstrates how to calculate the distance (or length) between two points (P and Q) in 3D using the same magnitude formula.
• 😀 The script provides an example where the coordinates of points P and Q are used to calculate the length of vector PQ.
• 😀 The vector subtraction operation is explained by finding the difference between the coordinates of two points, P and Q.
• 😀 For the vector subtraction of two points P and Q, the new vector is computed by subtracting corresponding components of the points' coordinates.
• 😀 The video also discusses how to convert a vector from column form to its component form (i, j, k).
• 😀 Operations on vectors, such as addition, subtraction, and scalar multiplication, are performed by directly operating on the vector components.
• 😀 The script concludes by introducing a more complex example involving the combination of vectors A, B, and C, showcasing how to compute the result of scalar multiplication and addition of vectors in 3D space.

Q & A

What is the main topic of the video?

The main topic of the video is the concept of vectors in 3-dimensional space, including how to represent and calculate vector operations such as position, magnitude, and algebraic operations in 3D space.

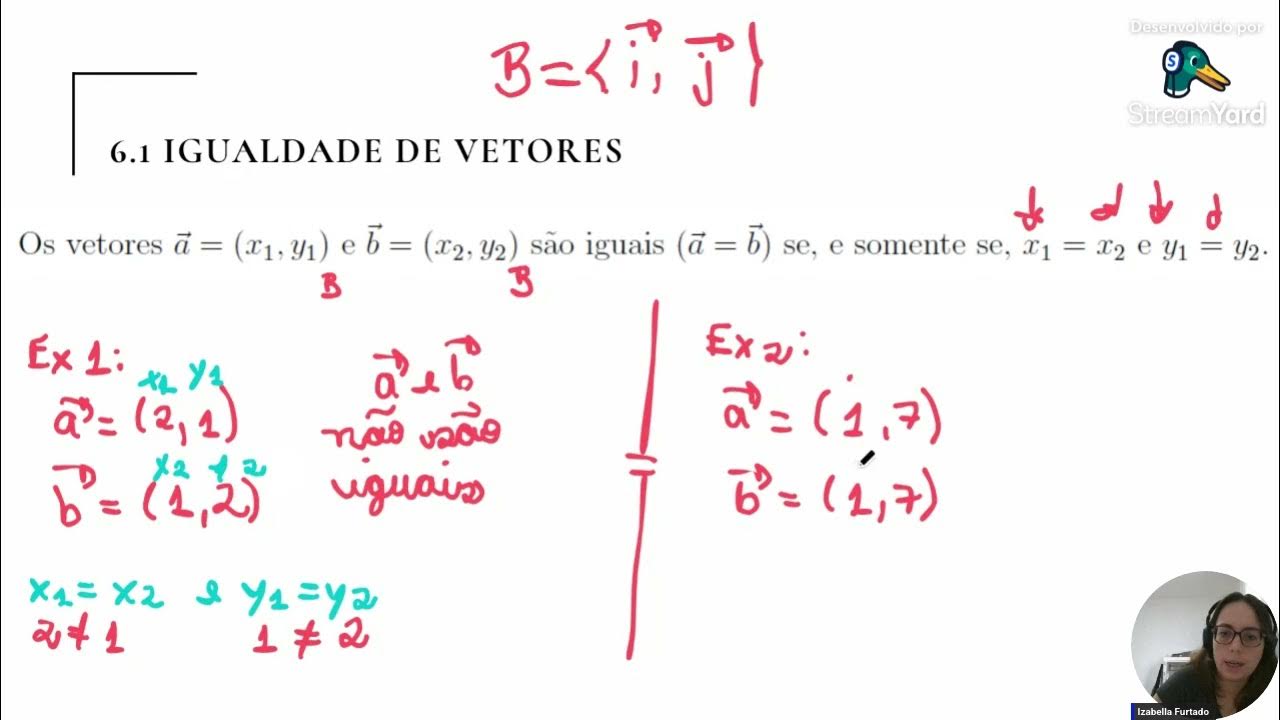
How are unit vectors in 3D space different from those in 2D space?

In 3D space, unit vectors are represented as i, j, and k, corresponding to the x, y, and z axes respectively. In 2D space, only i and j are used, corresponding to the x and y axes.

What is the formula for the length (magnitude) of a vector in 3D space?

The length of a vector in 3D space is calculated using the formula: √(A1² + A2² + A3²), where A1, A2, and A3 are the components of the vector.

How do you calculate the displacement vector between two points P and Q in 3D?

The displacement vector PQ can be calculated by subtracting the coordinates of point P from the coordinates of point Q. The formula is: (B1 - A1, B2 - A2, B3 - A3), where (A1, A2, A3) are the coordinates of P and (B1, B2, B3) are the coordinates of Q.

What is the method for finding the magnitude of the displacement vector?

The magnitude of the displacement vector is found by taking the square root of the sum of the squares of its components. For example, for a displacement vector (x, y, z), the magnitude is √(x² + y² + z²).

How do you express a vector in unit vector form using i, j, k notation?

To express a vector in unit vector form, multiply each component of the vector by its corresponding unit vector i, j, or k. For example, a vector (A1, A2, A3) can be written as A1i + A2j + A3k.

What operation is used to add or subtract vectors in 3D space?

To add or subtract vectors in 3D space, you simply add or subtract their corresponding components. For example, if vector A = (A1, A2, A3) and vector B = (B1, B2, B3), then A + B = (A1 + B1, A2 + B2, A3 + B3), and A - B = (A1 - B1, A2 - B2, A3 - B3).

How do you calculate the result of multiplying a vector by a scalar?

To multiply a vector by a scalar, multiply each component of the vector by the scalar. For example, if vector A = (A1, A2, A3) and the scalar is k, the result of kA is (k * A1, k * A2, k * A3).

What is the result of the operation 3A + 2B - C, given specific vector values?

To solve 3A + 2B - C, multiply each component of vector A by 3, vector B by 2, and subtract vector C. For example, if A = (1, 2, 3), B = (-3, -2, -1), and C = (1, -2, 3), then 3A + 2B - C = (3, 6, 9) + (-6, -4, -2) - (1, -2, 3), resulting in (-4, -3, 5).

What is the difference between the vector operations discussed in the video and basic arithmetic operations?

Vector operations, such as addition, subtraction, and scalar multiplication, work on the components of vectors in multi-dimensional space, while basic arithmetic operations are performed on individual numbers. The key difference is that vector operations are done component-wise, maintaining the direction and magnitude of the vectors involved.