Adding & Subtracting Radical Expressions
Summary
TLDRThis video explains the process of adding and subtracting radical expressions, drawing parallels to combining like terms in algebra. It covers how to combine radicals with the same radicand (e.g., 2√5 + 6√5 = 8√5), and emphasizes that radicals must be identical to combine. The video demonstrates simplifying radicals (like √12 into 2√3) to make them easier to combine, and discusses when simplification isn't enough. Key takeaway: working with radicals requires matching radicands, and simplification may be necessary for effective addition and subtraction.
Takeaways
- 😀 Radical expressions can be added or subtracted similarly to combining like terms in algebra.
- 😀 To combine radical expressions, the radicals must have the same square root.
- 😀 Example 1: 2 * √5 + 6 * √5 can be combined to 8 * √5 because the square roots are the same.
- 😀 Only terms with the exact same radicand can be combined, similar to how you can't combine terms with different variables.
- 😀 Example 2: √B + 6 * √2B - 5 * √B shows that you can combine √B terms, but not √2B.
- 😀 When simplifying, break down perfect square factors (e.g., √12 can be rewritten as 2 * √3).
- 😀 Example 3: 2 * √12 + 6 * √3 involves simplifying √12 to 2 * √3, resulting in 4 * √3 + 6 * √3, which can be combined to 10 * √3.
- 😀 Always check for perfect square factors to simplify the square root before attempting to combine terms.
- 😀 Example 4: √200 and √75 can be simplified to 10 * √2 and 5 * √3, respectively, but they cannot be combined due to different radicands.
- 😀 The key to adding and subtracting radicals is recognizing when simplification can lead to combining terms with the same square root.
Q & A
What is the main idea behind adding and subtracting radical expressions?
-Adding and subtracting radical expressions is similar to combining like terms in algebra. The key is that the square roots must have the same radicand in order to combine them.
What do we need to have in common to combine radical expressions?
-To combine radical expressions, they must have the same square root, or the same radicand, inside the radical.
How is the process of combining radical expressions similar to combining like terms in algebra?
-Just like combining like terms in algebra (e.g., 6X + 2X = 8X), combining radical expressions involves adding or subtracting terms with the same square root, such as 2√5 + 6√5 = 8√5.
In the expression 2√5 + 6√5, why can these terms be combined?
-The terms can be combined because both terms have the same square root, √5, making them like terms.
Why can't we combine the terms 6√b and 5√2b?
-We cannot combine these terms because they have different radicands, √b and √2b, so they are not like terms.
What is the significance of simplifying square roots before combining terms?
-Simplifying square roots is important because it might make the radicands the same, which allows for the terms to be combined. For example, simplifying √12 into 2√3 makes it possible to combine like terms.
In the expression 2√12 + 6√3, how can we simplify the square root of 12?
-We simplify √12 by factoring it into √(4 * 3), and then simplifying it to 2√3, allowing the terms to become 4√3 and 6√3, which can be combined.
How do perfect square factors help in simplifying radicals?
-Perfect square factors, like 4, 9, 16, etc., help simplify radicals by allowing us to pull out the square root of those factors. For example, √200 can be simplified by factoring it into √(100 * 2), which simplifies to 10√2.
What should we do if we cannot find a common radicand between terms?
-If we cannot find a common radicand, we simply leave the terms as they are and do not combine them. For example, 10√2 + 5√3 cannot be simplified further because their radicands are different.
What role does the 'radicand' play in simplifying and combining radical expressions?
-The radicand is the number or expression inside the square root. For terms to be combined, their radicands must be identical. If they aren't, the terms cannot be combined, even if the coefficients are the same.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

An Intro to Combining Like Terms | Simplifying Expressions by Combining Like Terms | Math with Mr. J
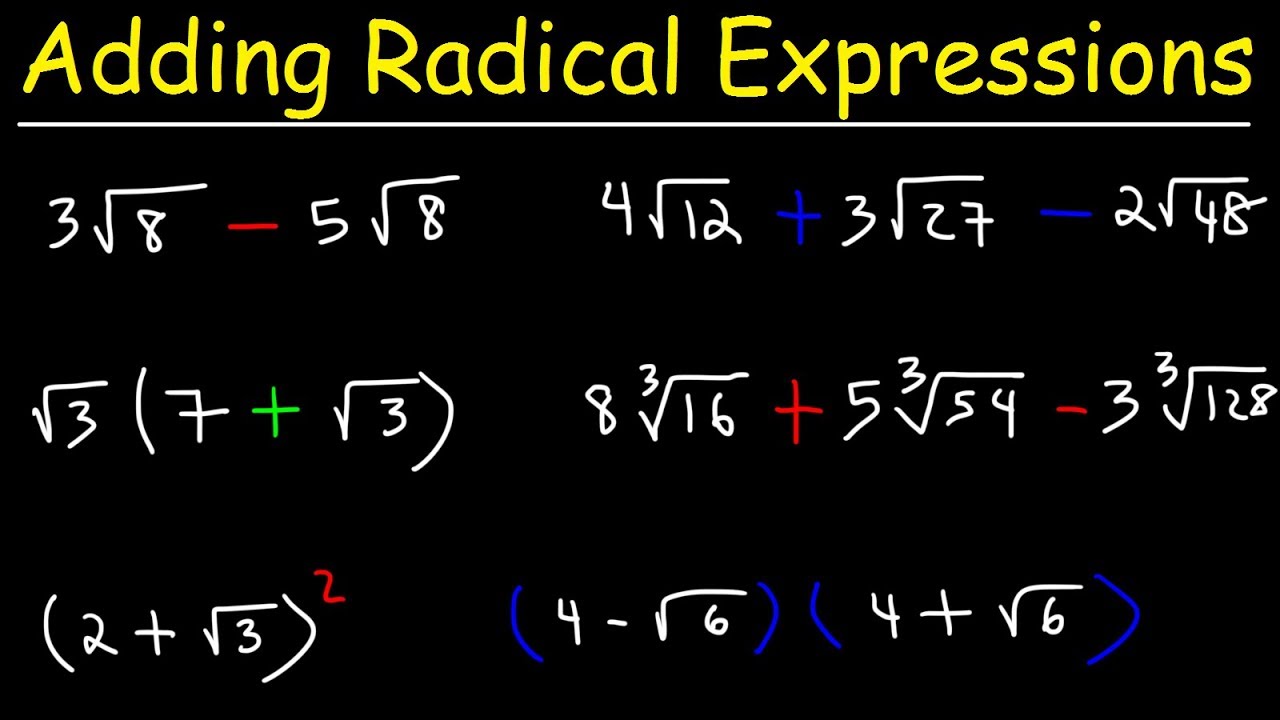
Adding and Subtracting Radical Expressions With Square Roots and Cube Roots

1 PENGERTIAN ALJABAR - ALJABAR - KELAS 7 SMP
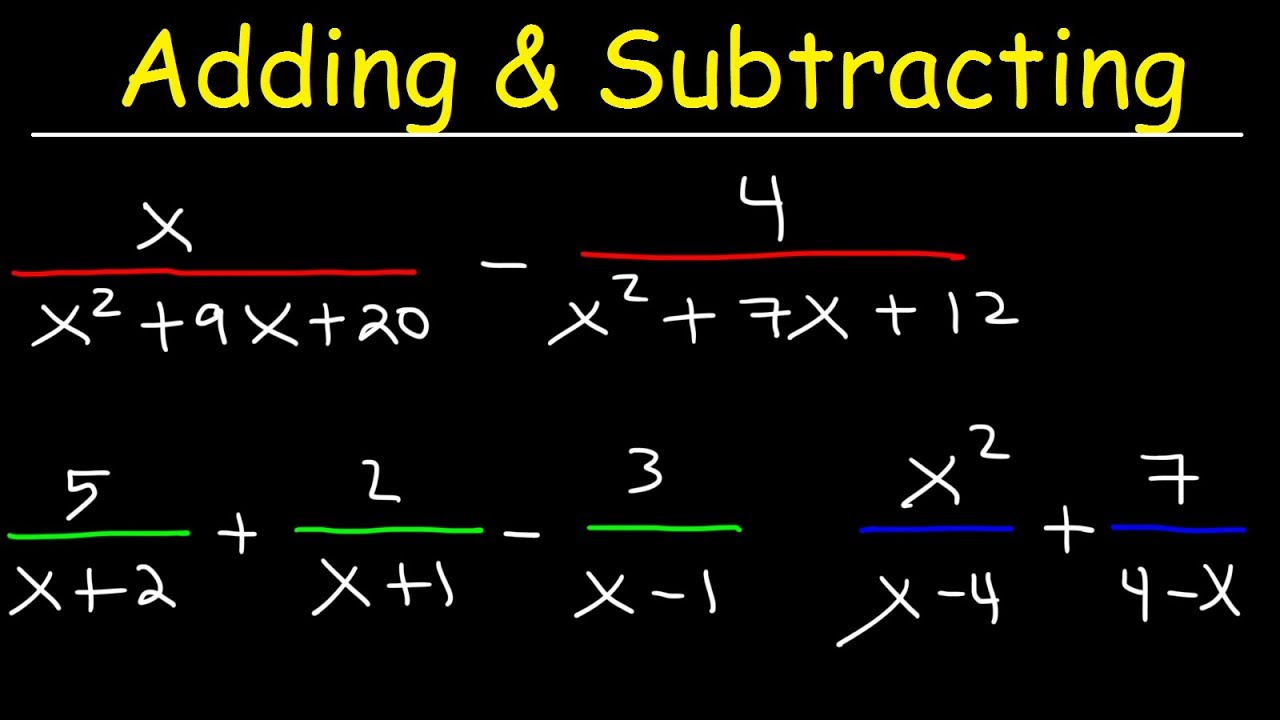
Adding and Subtracting Rational Expressions With Unlike Denominators

Kurikulum Merdeka Matematika Kelas 7 Bab 4 Bentuk Aljabar

2 PENJUMLAHAN DAN PENGURANGAN ALJABAR - ALJABAR - KELAS 7 SMP
5.0 / 5 (0 votes)
