Lec 38 - Graphs of Polynomials: Multiplicities
Summary
TLDRThis educational video delves into the intricacies of polynomial functions, focusing on identifying their properties, roots, and behaviors through graphical analysis. Viewers learn to discern whether a function is polynomial, find x-intercepts, and understand the impact of root multiplicities on graph shape. The video highlights critical distinctions between odd and even degree polynomials, explaining how these affect the graph's interaction with the x-axis—whether it crosses or bounces off. By mastering these concepts, learners gain a foundational skill set for further exploration in polynomial mathematics.
Takeaways
- 😀 Mastering skills to identify polynomial functions and their roots is essential for understanding polynomial behavior.
- 🔍 Knowledge of x-intercepts and y-intercepts helps visualize the overall shape of polynomial graphs.
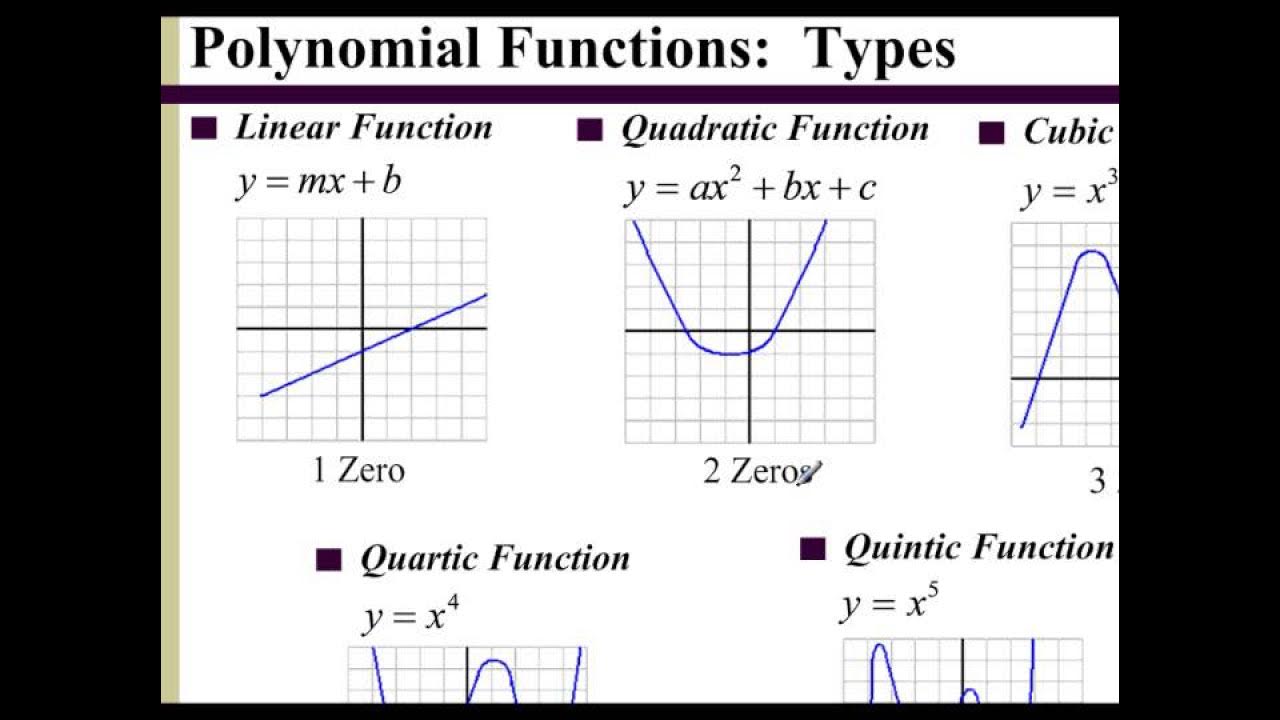
- 📊 The graph of a polynomial function is smooth and can have specific turning points depending on its degree.
- ✏️ Finding x-intercepts can be done through plotting function values at key points when the polynomial is not in factored form.
- 📈 The multiplicity of a root determines how the graph behaves at the x-axis: even multiplicities cause the graph to bounce off, while odd multiplicities allow it to cross.
- 🔗 Polynomial long division can be used to find additional roots once some roots are known.
- 🧩 Understanding the behavior of polynomial graphs around x-intercepts requires knowledge of their multiplicities and degrees.
- 🎨 Odd degree polynomials cross the x-axis, while even degree polynomials touch and bounce off the x-axis.
- ⚖️ The steeper the polynomial's degree, the more pronounced the curve's behavior near its roots.
- 📚 Graphing tools can enhance understanding of polynomial functions by visually representing their characteristics and behaviors.
Q & A
What are the two skills that have been mastered regarding polynomial functions?
-The two skills mastered are identifying whether a given function is a polynomial function and using algebraic expressions to find the roots or zeros of the polynomial.
How can the x-intercepts of a polynomial function be identified?
-X-intercepts can be identified by plotting values of the polynomial function at standard points and observing where the function equals zero.
What is the importance of understanding the multiplicity of a root?
-Understanding the multiplicity of a root helps in determining how the graph behaves at that root, specifically whether it crosses the x-axis or bounces off it.
What is the difference in graph behavior at x-intercepts for even and odd multiplicities?
-Graphs with even multiplicities touch and bounce off the x-axis at the intercept, while graphs with odd multiplicities cross the x-axis.
What is the significance of identifying the roots of a polynomial?
-Identifying the roots allows for constructing the polynomial in factored form, which aids in understanding its graph and behavior.
How does the degree of a polynomial influence its graph?
-The degree of a polynomial affects the number of times it can turn (maximum of degree - 1) and influences whether the graph will cross or bounce at x-intercepts.
What methods can be used to find the roots of a cubic polynomial if it is not in factored form?
-One method is to use polynomial long division after identifying some roots through plotting points and constructing a table of values.
What happens to the graph of a polynomial with higher even degrees near its x-intercepts?
-As the degree increases, the graph appears flatter and broader near the x-intercepts, meaning it will still touch the x-axis but will do so more gently.
Why is it important to use both analytical and graphical tools in understanding polynomial functions?
-Using both tools provides a comprehensive understanding of polynomial behavior, enabling accurate graphing and identification of key features like intercepts and turning points.
Can a cubic polynomial have more than three roots?
-No, a cubic polynomial can have at most three roots, as dictated by its degree.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

Graphs of Polynomials: Identification and Characterization

Grade 10 - Illustrating and graphing polynomial functions | SirABC
Lesson 6.3 - Identifying a Polynomial Function from the Graph

Lec 52 - Logarithmic Functions

CBSE Class 11 Maths Project-Relations & Functions

Polinomial (Bagian 5) - Cara Menentukan Akar-akar Persamaan Polinomial
5.0 / 5 (0 votes)
