ALL OF GRADE 11 MATH IN 1 HOUR! (exam review part 1) | jensenmath.ca
Summary
TLDRThis script is a comprehensive review for a grade 11 functions math course, covering key topics like identifying functions using the vertical line test, stating domains and ranges, and function notation. It delves into quadratic functions, explaining how to find vertices through completing the square and partial factoring, and solving quadratics by factoring, using the quadratic formula, and solving linear-quadratic systems. The video also touches on simplifying and subtracting rational expressions, emphasizing the importance of factoring and stating restrictions to avoid undefined expressions.
Takeaways
- 📚 The video is a review for grade 11 functions math, covering main topics and exam expectations.
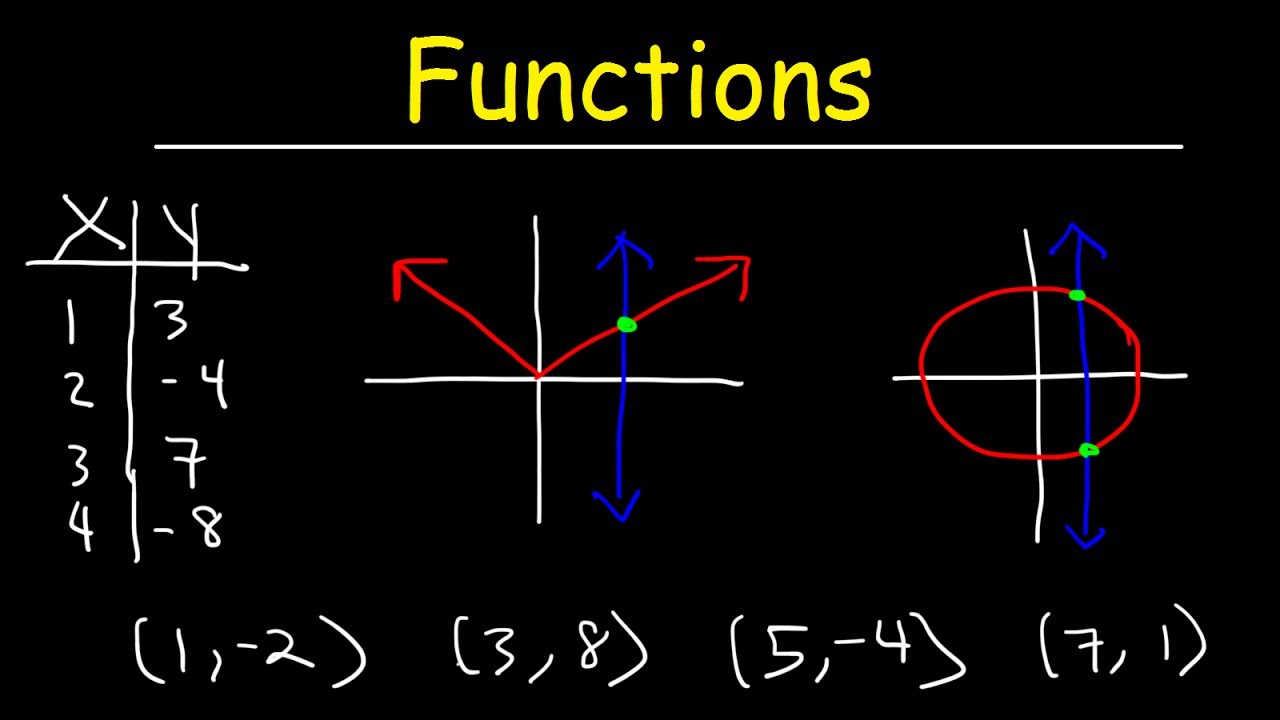
- 🔍 It discusses how to determine if a relation is a function using the vertical line test.
- 📈 The script explains how to define the domain and range of a relation, with examples.
- 📝 Function notation is introduced, replacing 'y' with 'f(x)' to represent the y-value of a function at a given x.
- 🔢 Techniques for finding the vertex of a quadratic function are reviewed, including completing the square and partial factoring.
- 📉 Methods for solving quadratic equations are detailed, such as factoring and using the quadratic formula.
- 🤝 The script covers how to solve systems of linear and quadratic equations, including substitution and factoring.
- ✅ It emphasizes the importance of stating restrictions when simplifying rational expressions to avoid undefined values.
- 🧮 Tips for subtracting rational expressions are provided, highlighting the need for common denominators and fully factored expressions.
- 📊 The video concludes with a quick overview of rational expressions, including simplification and stating restrictions.
Q & A
What is the primary condition for a relation to be considered a function?
-A relation is considered a function if each value in the domain corresponds to exactly one value in the range, passing the vertical line test.
How do you perform the vertical line test to determine if a relation is a function?
-You draw a vertical line anywhere on the graph of the relation and check if it intersects the graph at more than one point. If it does, the relation fails the test and is not a function.
Can you explain how to determine the domain and range of a relation?
-The domain is the set of all possible x-values the relation can take, while the range is the set of all possible y-values. You determine the domain by looking at the horizontal extent of the relation and the range by looking at the vertical extent.
What is function notation and how is it used?
-Function notation, such as f(x), is a way to represent a function where 'f' is the function name and 'x' is the input. It replaces y in the equation y = ... and is used to find the y-value (output) for a given x-value (input).
Describe the process of completing the square to find the vertex of a quadratic function.
-Completing the square involves rewriting the quadratic equation in vertex form by factoring a perfect square trinomial and adjusting the constant term to maintain equality, which reveals the vertex of the parabola.
How do you find the vertex of a quadratic function using partial factoring?
-Partial factoring involves setting the quadratic equation equal to a known y-intercept and solving for x-values that yield this y-intercept. The vertex's x-coordinate is the average of these x-values.
What are the different methods to solve quadratic equations discussed in the script?
-The script discusses completing the square, partial factoring, factoring by grouping, and using the quadratic formula as methods to solve quadratic equations.
How does the discriminant affect the number of solutions when solving a quadratic equation?
-The discriminant (b^2 - 4ac) determines the nature of the solutions: a positive discriminant results in two distinct real solutions, zero gives one real solution (a repeated root), and a negative discriminant results in no real solutions (two complex solutions).
What is the significance of the discriminant when solving a linear-quadratic system?
-The discriminant's value dictates the number of intersection points (solutions) between the line and the parabola: two points for a positive discriminant, one point for a zero discriminant (tangent line), and no intersection points for a negative discriminant.
How do you simplify rational expressions, and what are the key steps involved?
-To simplify rational expressions, you factor the numerator and denominator, then cancel out common factors. Key steps include changing division to multiplication, factoring, and reducing by canceling common factors across the numerator and denominator.
When subtracting rational expressions, what is the first step you should take?
-The first step when subtracting rational expressions is to ensure both expressions have a common denominator, which may involve factoring each expression fully.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео
5.0 / 5 (0 votes)