Menemukan Konsep Dilatasi Materi SMA Kelas XI Wajib

Summary

This video lesson explores the concept of dilation, focusing on scaling objects and figures in mathematics. The instructor uses various examples, like miniature models, wayang puppets, and balloons, to illustrate how dilation can enlarge or shrink shapes without changing their form. The lesson delves into Cartesian coordinates, explaining dilation from different centers, like point O (0,0) or other points, and discusses scale factors that either enlarge or reduce objects. Several mathematical examples are provided, guiding students through solving dilation-related problems step by step.

Takeaways

• 📏 Dilatation is the process of enlarging or shrinking a shape while maintaining its proportions.
• 🖼️ A miniature object retains the same shape as its original, but with a different scale.
• 🎭 Dilatation is commonly seen in traditional puppet shows where the shadow is enlarged or reduced.
• 🎈 A simple illustration of dilatation can be seen when blowing up a balloon, changing its size while keeping the shape intact.
• 🔢 In a Cartesian plane, dilatation can occur with respect to a point of origin (0,0) or another fixed point.
• ⚖️ The scale factor (k) determines whether the shape will be enlarged (k > 1) or reduced (0 < k < 1).
• 🔄 If the scale factor is negative, the image is reflected as well as scaled.
• 🧮 Dilatation formulas help in determining the new coordinates of a point after scaling with respect to the origin or another point.
• 📐 Congruency is maintained during dilatation, meaning the shape remains the same even if the size changes.
• ✏️ Practical examples of dilatation include applying it to different shapes like triangles, rectangles, and circles on the Cartesian plane.

Q & A

What is the concept of dilation in mathematics?

Dilation is a transformation that changes the size of a figure, either enlarging or reducing it, without altering its shape. This transformation is characterized by a scale factor, which determines the degree of size change, and a center point, which is the origin of the dilation.

Why is learning about dilation important?

Learning about dilation is important because it helps us understand how objects can be resized proportionally in real-world situations, such as in art, design, and engineering. It provides insight into how scale factors affect the dimensions of objects while maintaining their original shape.

What is the difference between dilation with scale factors greater than 1 and less than 1?

A scale factor greater than 1 results in an enlargement of the object, making it bigger than the original. A scale factor less than 1 results in a reduction, making the object smaller than the original. Both transformations maintain the shape of the object.

How does dilation affect the coordinates of a shape on a Cartesian plane?

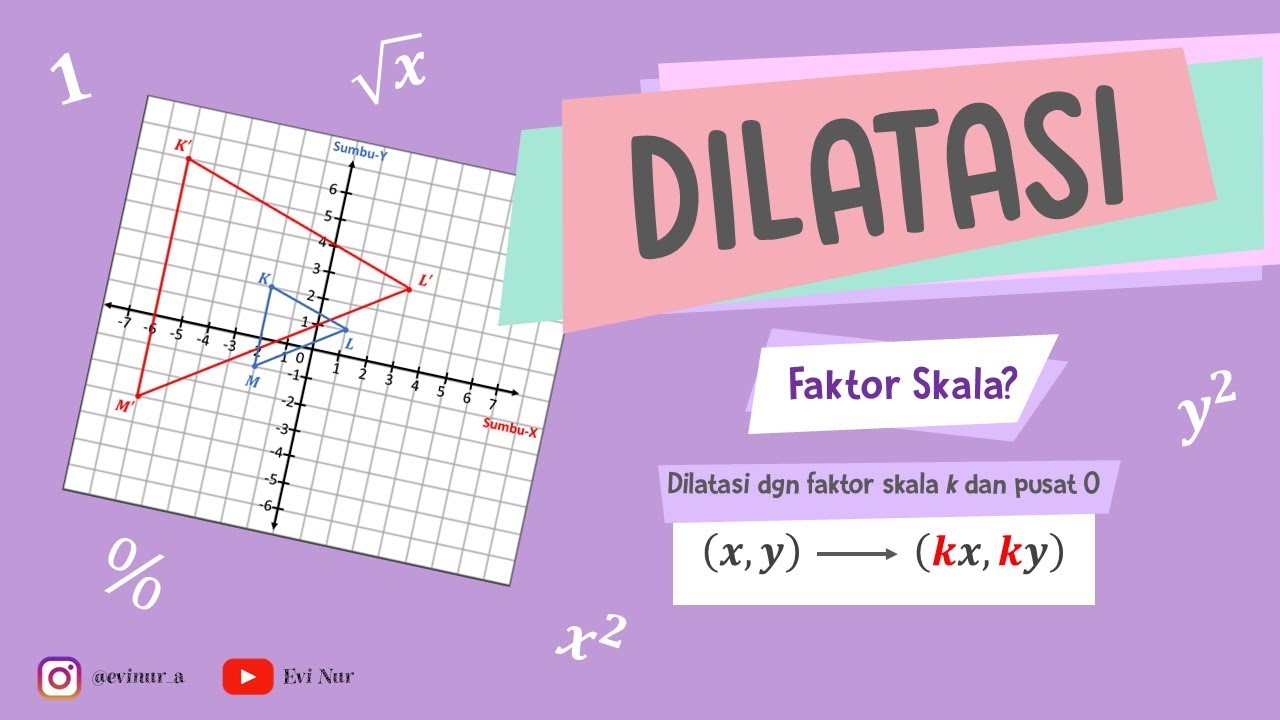
Dilation affects the coordinates of a shape on a Cartesian plane by multiplying each coordinate by the scale factor. If the dilation is centered at the origin (0,0), the new coordinates are simply the original coordinates multiplied by the scale factor.

What happens during dilation when the scale factor is negative?

When the scale factor is negative, the object is not only resized but also flipped across the dilation center. The negative scale factor results in an image that is oriented in the opposite direction from the original figure.

What is the role of the dilation center in the transformation?

The dilation center is the fixed point from which the dilation is performed. The distances from this point to all other points in the shape are scaled by the factor of dilation, dictating how far the transformed points will be from the center compared to the original points.

How do you perform dilation on a point not centered at the origin?

To perform dilation on a point not centered at the origin, adjust the coordinates by subtracting the center coordinates, apply the scale factor, and then add the center coordinates back. This ensures the dilation is accurately performed relative to the specified center point.

What are the properties of dilation when the scale factor is 1?

When the scale factor is 1, the figure does not change in size; it remains congruent to the original. This means that the transformation essentially has no effect on the object’s dimensions or orientation.

How can dilation be represented using matrices?

Dilation can be represented using matrices by multiplying the coordinate vector of a point by a dilation matrix that contains the scale factor. For dilation with respect to the origin, the matrix form is simply a diagonal matrix with the scale factor on the diagonal.

How does dilation relate to real-world examples like blowing up a balloon or a shadow puppet performance?

In real-world examples, dilation can be seen when a balloon is inflated, increasing its size without changing its overall shape. Similarly, in shadow puppet performances, figures appear to grow when moved closer to the light source, effectively demonstrating dilation with a larger scale factor.