Imaginary Numbers Are Real [Part 1: Introduction]
Summary
TLDRThe video script 'Imaginary Numbers Are Real [Part 1: Introduction]' explores the concept of imaginary numbers, which are often misunderstood. It starts by examining the function f(x) = x^2 + 1, which has no real roots, contrary to the Fundamental Theorem of Algebra that states every polynomial equation has roots equal to its degree. The script suggests that our traditional number system is incomplete, lacking a dimension to account for the square root of negative one. It introduces the idea that these 'missing' numbers exist in a new dimension and are wrongly named 'imaginary,' with Gauss proposing 'lateral' as a more fitting term. The video promises to delve into the history and significance of these numbers in future parts, questioning their real-world relevance and their role in solving mathematical problems.
Takeaways
- 📚 The script introduces the concept of imaginary numbers, starting with the function f(x) = x^2 + 1, which has no real roots but is supposed to have two according to the Fundamental Theorem of Algebra.
- 🌐 It explains that the traditional one-dimensional number line is incomplete and that imaginary numbers exist in a new dimension, related to the square root of negative one.
- 🔍 The script suggests that the term 'imaginary' is misleading and Gauss proposed the term 'lateral' to better represent these numbers.
- 🤔 It raises questions about why students are taught about numbers that were not understood or accepted for thousands of years, such as negative and imaginary numbers.
- 📈 The development of mathematics has been incremental, with natural numbers being the first to be used, followed by the introduction of fractions, zero, and negative numbers.
- 🧩 The acceptance of zero and negative numbers was not immediate and was met with skepticism due to their abstract nature and lack of tangible connection to the real world.
- 🔧 Negative numbers became essential for solving algebraic problems and expressing concepts like debt, eventually becoming an indispensable part of mathematics.
- 👨🏫 The script mentions that even renowned mathematicians like Euler struggled with the concept of negative numbers, indicating the complexity of these ideas.
- 🌟 Gauss is highlighted as a significant figure in the understanding of polynomial equations and the roots they possess, including those in the 'lateral' or imaginary dimension.
- 📉 The script implies that the journey to understand and accept imaginary numbers is part of a larger narrative of mathematical progress and overcoming conceptual barriers.
- 🔮 The next part of the series promises to delve into the historical discovery of imaginary numbers and address the questions raised in this introduction.
Q & A
What is the function f(x) discussed in the script?
-The function discussed in the script is f(x) = x^2 + 1, which is a quadratic function resulting in a parabolic graph.
Why does the parabola of f(x) = x^2 + 1 not cross the x-axis?
-The parabola does not cross the x-axis because the equation x^2 + 1 = 0 has no real solutions, as the square of a real number cannot be negative.
What is the Fundamental Theorem of Algebra?
-The Fundamental Theorem of Algebra states that every polynomial equation of degree n has exactly n roots, which can be real or complex.
Why does the script suggest that our number system is incomplete?
-The script suggests that our number system is incomplete because it does not account for the roots of equations like x^2 + 1 = 0, which exist in a new dimension beyond the real numbers.
What is the significance of the square root of negative one in this context?
-The square root of negative one, denoted as 'i', is significant as it represents the introduction of imaginary numbers, which, along with real numbers, form the complex numbers that can solve equations with no real roots.
What does Gauss suggest as an alternative name for imaginary numbers?
-Gauss suggested the term 'lateral' as an alternative to 'imaginary' to better reflect the fact that these numbers exist in a different dimension rather than being unreal.
Why were early humans only concerned with natural numbers?
-Early humans were only concerned with natural numbers because they were sufficient for counting and basic arithmetic, which were the primary uses of numbers at that time.
How did the introduction of fractions change the number system?
-The introduction of fractions filled in the gaps between whole numbers on the number line, allowing for a more precise representation of quantities and advancements in mathematical understanding.
What was the initial skepticism towards zero and negative numbers?
-The initial skepticism towards zero and negative numbers was due to their abstract nature and the difficulty in relating them to tangible realities, leading to their avoidance or rejection in some cultures.
Why are negative numbers essential in mathematics?
-Negative numbers are essential in mathematics because they allow for the expression of concepts like debt, temperature below zero, and are necessary for solving a wide range of algebraic problems.
What questions do negative and imaginary numbers raise about the nature of mathematics?
-Negative and imaginary numbers raise questions about why we study numbers that were historically difficult to understand and accept, and how these numbers, seemingly disconnected from the real world, are crucial for solving mathematical problems.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示
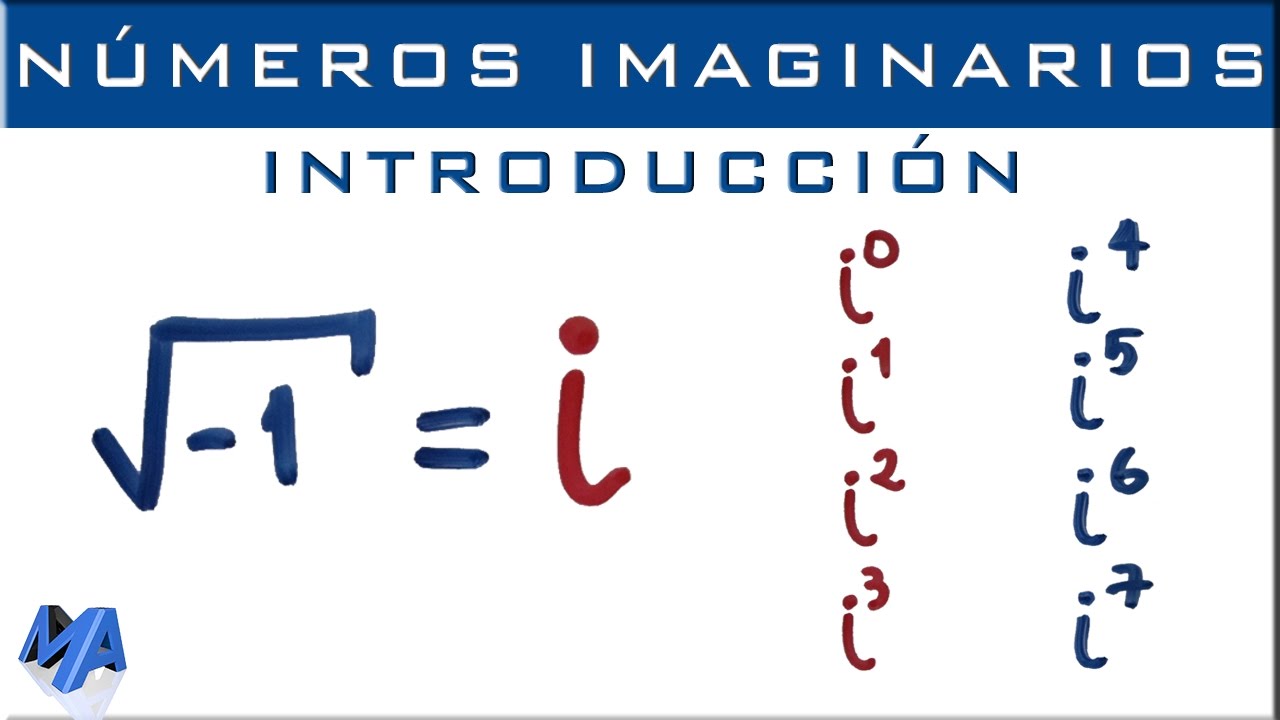
Números imaginarios | Introducción y potencias de "i"

kompleks 01 pendahuluan

Complex Numbers 01 | Introduction to Complex Numbers | Class 11 | JEE

APA ITU BILANGAN KOMPLEKS ? (Materi Kurikulum Merdeka)

LATIHAN A NO 1 2 3 BILANGAN KOMPLEKS MATEMATIKA TINGKAT LANJUT SMA KELAS 11 #kurikulummerdeka
BAB 1 Sistem Bilangan | Matematika Dasar | Alternatifa
5.0 / 5 (0 votes)
