FACTORING PERFECT SQUARE TRINOMIALS || GRADE 8 MATHEMATICS Q1

Summary

This educational video script explains how to identify and factor perfect square trinomials. It clarifies that a perfect square trinomial must have a positive leading term that is a perfect square, a middle term twice the product of the square roots of the first and last terms, and a last term that is also a perfect square and positive. The script provides examples to illustrate the process, including incorrect cases, and demonstrates the factoring technique for perfect square trinomials, emphasizing the importance of checking each term's square status and the middle term's relationship to the others.

Takeaways

• 📚 The video explains how to identify and factor perfect square trinomials, which are algebraic expressions that can be written as the square of a binomial.
• 🔍 A perfect square trinomial must have a first term that is a perfect square and always positive, a middle term that is twice the product of the square roots of the first and last terms, and a last term that is also a perfect square and positive.
• 📉 The script provides examples of expressions that are and are not perfect square trinomials, helping to clarify the concept.
• 📝 To factor a perfect square trinomial, one should express it as the square of the sum or difference of two terms, depending on the sign of the middle term.
• 🤔 The video emphasizes the importance of checking if both the first and last terms are perfect squares and if the middle term is twice their product.
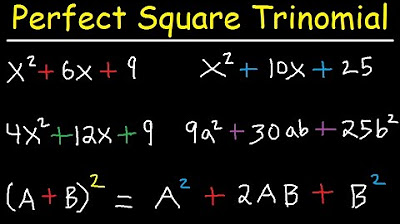
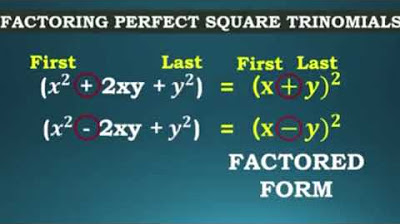
• 📐 It demonstrates the process of factoring perfect square trinomials by providing step-by-step examples, such as \(x^2 + 2xy + y^2\) which factors to \((x + y)^2\).
• 🚫 The script clarifies that not all trinomials are perfect squares, and it's crucial to verify the conditions before attempting to factor.
• ✅ The video includes a method to factor expressions that are not perfect square trinomials by first factoring out the greatest common factor (GCF), if applicable.
• 📝 The script gives a clear example of how to handle negative middle terms in perfect square trinomials, such as \(x^2 - 22x + 121\) which factors to \((x - 11)^2\).
• 🔢 The importance of recognizing perfect squares, such as \(4x^2\) being \((2x)^2\) and \(25\) being \(5^2\), is highlighted for successful factoring.
• 👍 The video concludes with an encouragement to like, subscribe, and follow the channel for more educational content.

Q & A

What is the main topic of the video?

The main topic of the video is identifying and factoring perfect square trinomials.

What is a perfect square trinomial?

A perfect square trinomial is an algebraic expression that can be written as the square of a binomial, meaning it has the form (ax + by)^2.

What are the characteristics of the first term in a perfect square trinomial?

The first term in a perfect square trinomial must be a perfect square and it is always positive.

What is the condition for the middle term of a perfect square trinomial?

The middle term must be twice the product of the square roots of the first and last terms.

What should the last term of a perfect square trinomial be?

The last term must also be a perfect square and it is always positive.

How can you determine if the expression 4x^2 + 20x + 25 is a perfect square trinomial?

You can determine it's a perfect square trinomial because the first term (4x^2) and the last term (25) are perfect squares, and the middle term (20x) is twice the product of the square roots of the first and last terms (2x * 5).

Why is the expression x^2 + 5x + 6 not a perfect square trinomial?

The expression x^2 + 5x + 6 is not a perfect square trinomial because the last term (6) is not a perfect square.

What is the factored form of a perfect square trinomial x^2 + 2xy + y^2?

The factored form of the perfect square trinomial x^2 + 2xy + y^2 is (x + y)^2.

How do you factor a perfect square trinomial with a negative middle term?

If the middle term is negative, the factored form is the square of the binomial with a negative sign, such as (x - y)^2.

What is the process for factoring the expression 16x^2 + 72x + 81?

First, confirm that the first and last terms are perfect squares (16x^2 and 81) and that the middle term (72x) is twice the product of their square roots (4x * 9). Then, factor it as (4x + 9)^2.

Can the expression 27a^2 + 72ab + 48b^2 be a perfect square trinomial?

No, the expression 27a^2 + 72ab + 48b^2 cannot be a perfect square trinomial because 27 and 48 are not perfect squares.

What is the factored form of the expression 4x^3 - 24x^2 + x, given it is a perfect square trinomial?

The factored form of the expression 4x^3 - 24x^2 + x is x(x - 6)^2, after factoring out the greatest common factor x.