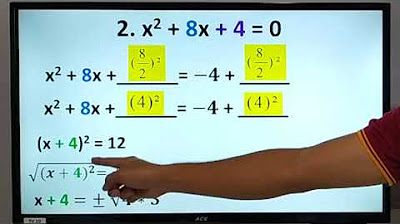Persamaan Kuadrat Kelas 10 Kurikulum Merdeka

Summary

This educational video provides a comprehensive guide to solving quadratic equations, focusing on three primary methods: factoring, completing the square, and using the quadratic formula. It begins with an explanation of the general form of a quadratic equation (ax² + bx + c = 0) and proceeds to demonstrate each solving technique through detailed examples. The video covers how to factor equations, complete the square to form perfect square trinomials, and apply the quadratic formula (abc formula) to find solutions. This lesson is designed to help students understand and apply these methods to find the solutions of quadratic equations.

Takeaways

• 😀 Quadratic equations are of the form ax² + bx + c = 0, where a, b, and c are constants, and a cannot be zero.
• 😀 To identify a quadratic equation, check if the highest power of x is 2 and ensure that a ≠ 0.
• 😀 The coefficient 'a' must not be zero in a quadratic equation; otherwise, the equation is no longer quadratic.
• 😀 The three main methods for solving quadratic equations are: factorization, completing the square, and using the quadratic formula (ABC formula).
• 😀 Factorization involves finding two numbers that multiply to give ac and add up to give b, then solving each factor set equal to zero.
• 😀 Completing the square involves rearranging the equation and adding a term to make it a perfect square trinomial, then solving for x.
• 😀 The quadratic formula is used to find the roots of a quadratic equation using the formula x = [-b ± √(b² - 4ac)] / 2a.
• 😀 The discriminant (b² - 4ac) in the quadratic formula determines the nature of the roots: if it's positive, there are two real roots; if it's zero, there’s one real root; if it's negative, the roots are complex.
• 😀 In factorization, after finding the factors, solutions are found by setting each factor equal to zero.
• 😀 For completing the square, the equation is manipulated so that the left side becomes a perfect square, and then solutions are derived by solving for x.
• 😀 The video emphasizes practicing each method to gain a deeper understanding of solving quadratic equations and their real-world applications.

Q & A

What is the general form of a quadratic equation?

The general form of a quadratic equation is ax² + bx + c = 0, where 'a' is the coefficient of x², 'b' is the coefficient of x, and 'c' is the constant. The key condition is that 'a' cannot be zero.

Why can't the coefficient of x² (a) be zero in a quadratic equation?

If 'a' equals zero, the term ax² becomes zero, and the equation becomes linear, not quadratic. This means the equation no longer contains a squared term, which disqualifies it from being classified as a quadratic equation.

In the equation 2x² + 5x - 3 = 0, what are the values of 'a', 'b', and 'c'?

In the equation 2x² + 5x - 3 = 0, 'a' = 2, 'b' = 5, and 'c' = -3.

What are the three main methods for solving a quadratic equation?

The three main methods for solving a quadratic equation are factorization, completing the square, and using the quadratic formula.

How do you solve a quadratic equation using factorization?

To solve a quadratic equation by factorization, you factor the quadratic into two binomials, set each factor equal to zero, and then solve for 'x'. For example, if the equation is x² + 10x + 21 = 0, the factors are (x + 3) and (x + 7), giving the solutions x = -3 and x = -7.

What is the method of completing the square?

Completing the square involves converting a quadratic equation into a perfect square trinomial, making it easier to solve. This is done by adding the square of half the coefficient of 'x' to both sides of the equation. For example, to solve x² + 6x + 2 = 0, you would move the constant to the other side, add (6/2)² = 9 to both sides, and then solve for 'x'.

What happens if the constant term 'c' in a quadratic equation is negative while completing the square?

If the constant term 'c' is negative, you move it to the other side of the equation and then add the square of half the coefficient of 'x' to both sides to form a perfect square trinomial. This makes it possible to solve the equation by taking the square root of both sides.

What is the quadratic formula, and when is it used?

The quadratic formula is given by x = (-b ± √(b² - 4ac)) / 2a. It is used to solve any quadratic equation, especially when factorization and completing the square are difficult or not possible.

How do you apply the quadratic formula to the equation 5x² + 2x - 7 = 0?

To apply the quadratic formula to 5x² + 2x - 7 = 0, identify 'a' = 5, 'b' = 2, and 'c' = -7. Then substitute these values into the formula: x = (-2 ± √(2² - 4(5)(-7))) / 2(5). This simplifies to x = (-2 ± √144) / 10, giving solutions x = 1 and x = -7/5.

What is the discriminant, and how does it affect the solutions of a quadratic equation?

The discriminant is the part of the quadratic formula under the square root: b² - 4ac. It determines the nature of the solutions of a quadratic equation. If the discriminant is positive, there are two real solutions. If it is zero, there is one real solution. If it is negative, the solutions are complex (non-real).