Probability of Independent and Dependent Events (6.2)
Summary
TLDRThis video script explores the concepts of independent and dependent events in probability. Independent events, such as rolling a die and flipping a coin, have outcomes that don't affect each other's probabilities. The script explains how to calculate the probability of both occurring with a simple formula. Dependent events, on the other hand, like drawing marbles without replacement, change the probability of subsequent events. The video provides examples and calculations for both scenarios, emphasizing the importance of understanding event dependence in probability.
Takeaways
- 🎲 Independent events are those where the occurrence of one event does not influence the probability of another event. For example, rolling a die and flipping a coin are independent because the result of one does not affect the other.
- 📐 The formula for calculating the probability of two independent events occurring together is the product of their individual probabilities: P(A and B) = P(A) * P(B).
- 🧩 To determine the probability of an event, you divide the number of favorable outcomes by the total number of possible outcomes.
- 🔢 For the example given, the probability of rolling a 5 on a 6-sided die is 1/6, and the probability of getting heads on a coin flip is 1/2.
- 🤔 Dependent events are the opposite of independent events; the occurrence of one event affects the probability of another. An example is drawing marbles from a box without replacement.
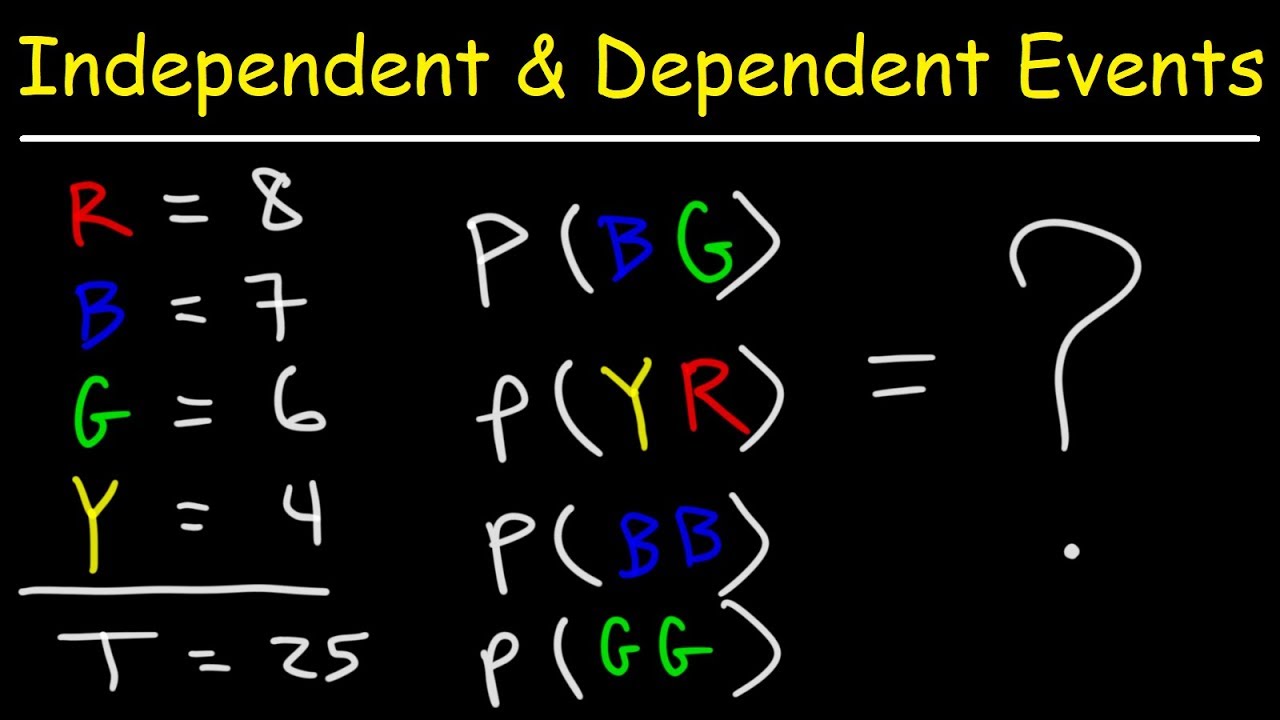
- 💚 The probability of drawing a green marble first and then a blue marble from a box of 10 marbles (7 green, 3 blue) changes after the first draw due to the reduction in total marbles and the specific color count.
- 📉 The probability of drawing a blue marble after drawing a green one without replacement changes from 3/10 to 3/9, reflecting the dependency of the events.
- 💙 To calculate the probability of two dependent events, you multiply the probability of the first event by the probability of the second event after the first has occurred.
- 🌐 The probability of drawing two green marbles without replacement is calculated by considering the change in the total number of marbles and the number of green marbles after the first draw.
- 📚 Understanding the difference between independent and dependent events is crucial for accurately calculating probabilities in various scenarios.
- 📈 The video provides a clear explanation of how to calculate probabilities for both independent and dependent events, using examples to illustrate the concepts.
Q & A
What are independent events?
-Independent events are those where the occurrence of one event does not affect the probability of another event. For example, rolling a die and flipping a coin are independent events because the outcome of the die roll does not influence the coin flip.
How do you calculate the probability of two independent events happening together?
-To calculate the probability of two independent events happening together, you multiply the probability of the first event by the probability of the second event. The formula is P(A and B) = P(A) * P(B).
What is the probability of rolling a 5 on a die and getting heads on a coin flip, given that both are independent events?
-The probability of rolling a 5 on a 6-sided die is 1/6, and the probability of getting heads on a coin flip is 1/2. To find the combined probability, multiply these two probabilities: (1/6) * (1/2) = 1/12 or 0.0833.
What are dependent events?
-Dependent events are those where the occurrence of one event affects the probability of another event. An example would be drawing two marbles from a box without replacement, where the outcome of the first draw affects the probability of the second.
How does the probability change when drawing two marbles without replacement from a box containing green and blue marbles?
-The probability changes because the total number of marbles and the number of marbles of each color decrease after the first draw. For instance, if you draw a green marble first, the probability of drawing a blue marble second is now 3/9 instead of 3/10.
What is the correct formula to use when calculating the probability of dependent events?
-For dependent events, the correct formula is P(A and B) = P(A) * P(B after A). This means you multiply the probability of the first event by the probability of the second event occurring after the first event has already occurred.
What is the probability of drawing two green marbles without replacement from a box of 10 marbles, with 7 green and 3 blue marbles?
-The probability of drawing the first green marble is 7/10. If the first marble drawn is green, there are now 6 green marbles left out of 9 total marbles. The probability of drawing a second green marble is 6/9. The combined probability is (7/10) * (6/9) = 7/15 or 0.4667.
Why is it incorrect to use the formula for independent events when dealing with dependent events?
-It is incorrect because the formula for independent events assumes that the outcome of one event does not affect the outcome of the other. In dependent events, the outcome of the first event changes the conditions for the second event, thus altering its probability.
What is the difference between drawing with replacement and drawing without replacement?
-Drawing with replacement means that after an item is drawn, it is put back into the set before the next draw, keeping the total number of items constant. Drawing without replacement means the item is not put back, thus reducing the total number of items available for subsequent draws.
How can you determine if events are independent or dependent?
-You can determine if events are independent by checking if the outcome of one event has no effect on the probability of the other event. If the outcome of one event changes the probability of the other, then the events are dependent.
What is the probability of drawing a green marble and then a blue marble without replacement from a box with 10 marbles, 7 green and 3 blue?
-The probability of the first event, drawing a green marble, is 7/10. After drawing a green marble without replacement, the probability of the second event, drawing a blue marble, is 3/9. The combined probability is (7/10) * (3/9) = 7/30 or 0.2333.
What is the significance of the number of favorable outcomes and total possible outcomes in calculating probabilities?
-The probability of an event is calculated by dividing the number of favorable outcomes by the total number of possible outcomes. This gives the likelihood of the event occurring in a single trial.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Probability, Meaning and basic concepts of Probability
Probability - Independent and Dependent Events

Peluang Kejadian Saling Bebas dan Bersyarat (Tidak Saling Bebas)

LEC_3 | PROBABILITY| CONDITIONAL Prob.|BAYE'S THEOREM| UNIT-4 (ST-2) #aktu #math4 @monikamittalmm

Peluang (Part 2) | Kejadian Majemuk | Saling Lepas, Tidak Saling Lepas, Saling Bebas, dan Bersyarat

Probability Concepts for Data Analysis and Data Science | Statistics for Data Science
5.0 / 5 (0 votes)
