Basic trigonometry II | Basic trigonometry | Trigonometry | Khan Academy

Summary

This educational script delves into the fundamentals of trigonometry, focusing on right triangles. It introduces the Pythagorean theorem to determine the hypotenuse and employs the 'soh cah toa' mnemonic to explain sine, cosine, and tangent. The script provides step-by-step calculations for various trigonometric ratios, including rationalizing denominators and demonstrates the process using 30-60-90 triangles. It highlights the relationships between the trigonometric functions of complementary angles, offering a clear and practical understanding of trigonometric principles.

Takeaways

• 📐 The script focuses on understanding trigonometric functions within the context of right triangles, using the Pythagorean theorem to find the hypotenuse.
• 🔢 The mnemonic 'soh cah toa' is introduced to remember the trigonometric ratios: sine (opposite/hypotenuse), cosine (adjacent/hypotenuse), and tangent (opposite/adjacent).
• 📚 The script provides a step-by-step example of calculating the trigonometric functions for a right triangle with sides of length 7 and 4, resulting in a hypotenuse of √65.
• 📉 The concept of rationalizing the denominator is explained, which involves multiplying by the conjugate to eliminate irrational numbers from the denominator.
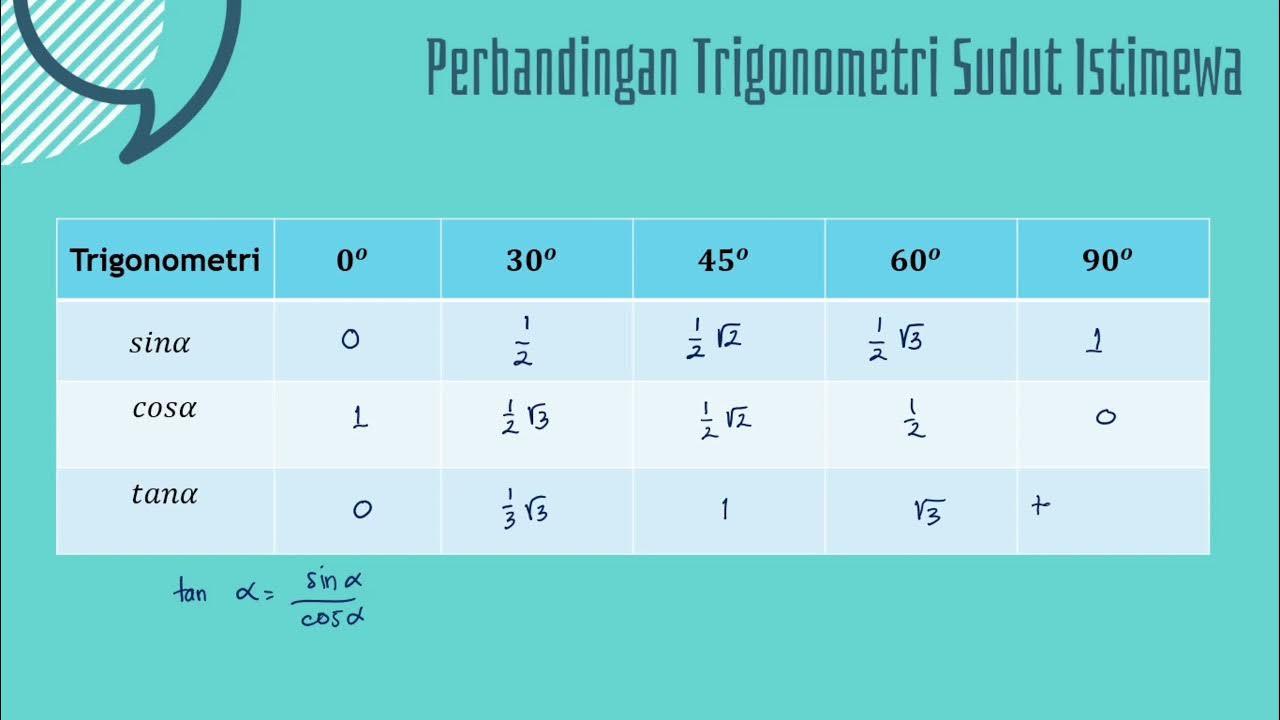
• 📈 The script demonstrates how to calculate the sine, cosine, and tangent for a 30-degree angle in a 30-60-90 triangle, highlighting the relationship between the sides and angles.
• 🔍 The sine of 30 degrees is shown to be 1/2, and the cosine is √3/2, with the tangent being √3/3, illustrating the basic trigonometric values for a 30-degree angle.
• 📐 For a 60-degree angle in the same triangle, the sine is √3/2, the cosine is 1/2, and the tangent is √3, showing the inverse relationship between the sine and cosine for complementary angles.
• 🧩 The script connects the trigonometric ratios for the 30-degree and 60-degree angles, emphasizing the complementary nature of these angles in a right triangle.
• 📝 The importance of understanding the positions of sides relative to the angles (opposite, adjacent, hypotenuse) is stressed for correctly applying trigonometric functions.
• 🔑 The script encourages practice to solidify the understanding of trigonometric functions and their relationships in right triangles.

Q & A

What is the purpose of constructing right triangles in the context of this script?

The purpose of constructing right triangles is to demonstrate and practice the application of trigonometric functions, which are initially defined for right triangles.

What is the Pythagorean theorem and how is it used in the script?

The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. In the script, it is used to calculate the length of the hypotenuse given the lengths of the other two sides.

How is the hypotenuse of a right triangle with sides of length 7 and 4 calculated in the script?

The hypotenuse is calculated using the Pythagorean theorem: h^2 = 7^2 + 4^2, which simplifies to h^2 = 49 + 16 = 65. Then, taking the square root of both sides gives h = √65.

What is the mnemonic 'soh cah toa' used for in trigonometry?

The mnemonic 'soh cah toa' is used to remember the definitions of the trigonometric functions sine, cosine, and tangent. Each letter represents a part of the definition: 'soh' for sine (opposite/hypotenuse), 'cah' for cosine (adjacent/hypotenuse), and 'toa' for tangent (opposite/adjacent).

How is the cosine of an angle in a right triangle defined in the script?

The cosine of an angle in a right triangle is defined as the length of the side adjacent to the angle divided by the length of the hypotenuse.

What is the process of rationalizing the denominator in the context of trigonometric ratios?

Rationalizing the denominator involves eliminating the irrational number from the denominator of a fraction. This is done by multiplying both the numerator and the denominator by the conjugate of the denominator, which in the case of a square root is the square root itself.

What is the sine of a 30-degree angle in a right triangle?

The sine of a 30-degree angle in a right triangle is equal to 1/2, as the opposite side is half the length of the hypotenuse.

How is the cosine of a 30-degree angle calculated in the script?

The cosine of a 30-degree angle is calculated as the length of the adjacent side (which is 2√3) divided by the hypotenuse (which is 4), simplifying to √3/2.

What is the tangent of a 30-degree angle in a right triangle?

The tangent of a 30-degree angle is the ratio of the opposite side (which is 2) to the adjacent side (which is 2√3), simplifying to √3/3 after rationalizing the denominator.

How are the trigonometric ratios for a 60-degree angle related to those of a 30-degree angle in the script?

The sine of a 60-degree angle is equal to the cosine of a 30-degree angle, and vice versa. The tangent of a 60-degree angle is the square root of 3, which is the reciprocal of the tangent of a 30-degree angle.