Identitas Trigonometri: Identitas Kebalikan, Perbandingan dan Pythagoras - SMA Kelas 10
Summary
TLDRThis educational video teaches key trigonometric identities, including reciprocal, ratio, and Pythagorean identities. The presenter explains the relationships between sine, cosine, tangent, and their reciprocals (cosecant, secant, cotangent) using both formulas and visual aids like a right-angled triangle. Key concepts such as the Pythagorean theorem and trigonometric functions are demonstrated with examples and practice problems. Additionally, the video includes practical steps for solving problems involving trigonometric ratios, such as finding cosine and tangent from known sine values. The session concludes with a few problem-solving exercises to reinforce the concepts.
Takeaways
- 😀 Reciprocal identities are key to trigonometry: sine, cosine, and tangent have corresponding reciprocal identities (cosecant, secant, and cotangent).
- 😀 Tangent is the ratio of sine to cosine, while cotangent is the reciprocal of tangent, illustrating how ratios work in trigonometry.
- 😀 Pythagorean identities are derived from the Pythagorean theorem and include important equations like sin²(α) + cos²(α) = 1.
- 😀 The reciprocal identity for cosecant is sin(α) = 1/cos(α), for secant is cos(α) = 1/sin(α), and for cotangent is tan(α) = 1/cot(α).
- 😀 By understanding these identities, one can simplify complex trigonometric expressions and solve equations more effectively.
- 😀 The sin²(α) + cos²(α) = 1 identity forms the foundation for many other trigonometric proofs and calculations.
- 😀 Trigonometric identities are used to simplify expressions like sin(α) * cos(α) or to prove complex equalities in trigonometry.
- 😀 Understanding the relation between tangent and cotangent is essential for simplifying trigonometric expressions.
- 😀 The script also explores how the Pythagorean theorem relates to trigonometric functions, providing a geometric basis for identities.
- 😀 Practice problems demonstrate how to apply these identities to calculate unknown values such as sine, cosine, and tangent, reinforcing learning.
Q & A
What are the three types of trigonometric identities discussed in the video?
-The three types of trigonometric identities discussed are reciprocal identities, ratio identities, and Pythagorean identities.
What is the reciprocal identity for sine (sin) in the video?
-The reciprocal identity for sine is: sin(α) = 1 / cos(α).
How is cosecant (csc) related to sine according to the video?
-Cosecant (csc) is the reciprocal of sine, so: csc(α) = 1 / sin(α).
What is the reciprocal identity for cosine (cos) as described in the video?
-The reciprocal identity for cosine is: cos(α) = 1 / sec(α), and sec(α) = 1 / cos(α).
What is the reciprocal identity for tangent (tan) in the video?
-The reciprocal identity for tangent is: tan(α) = 1 / cot(α), and cot(α) = 1 / tan(α).
What is the Pythagorean identity for sine and cosine?
-The Pythagorean identity for sine and cosine is: sin²(α) + cos²(α) = 1.
What happens when we divide the Pythagorean identity by cos²(α)?
-When dividing the Pythagorean identity by cos²(α), we get the identity: tan²(α) + 1 = sec²(α).
What happens when we divide the Pythagorean identity by sin²(α)?
-When dividing the Pythagorean identity by sin²(α), we get the identity: cot²(α) + 1 = csc²(α).
How do you calculate cos(α) and tan(α) if sin(α) = 12/13?
-To calculate cos(α), use the identity sin²(α) + cos²(α) = 1. Substituting sin(α) = 12/13 gives cos²(α) = 1 - (144/169), so cos(α) = 5/13. To calculate tan(α), divide sin(α) by cos(α): tan(α) = (12/13) / (5/13) = 12/5.
How do you simplify the expression x * tan(x) * cos(x)?
-The expression simplifies by recognizing that sec(x) = 1 / cos(x), so the expression becomes tan(x) * sec(x), which simplifies to sin(x) / cos(x) * 1 / cos(x) = sin(x) / cos(x).
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Identitas Trigonometri | Matematika Wajib Kelas X

Simplifying Trigonometric Expressions
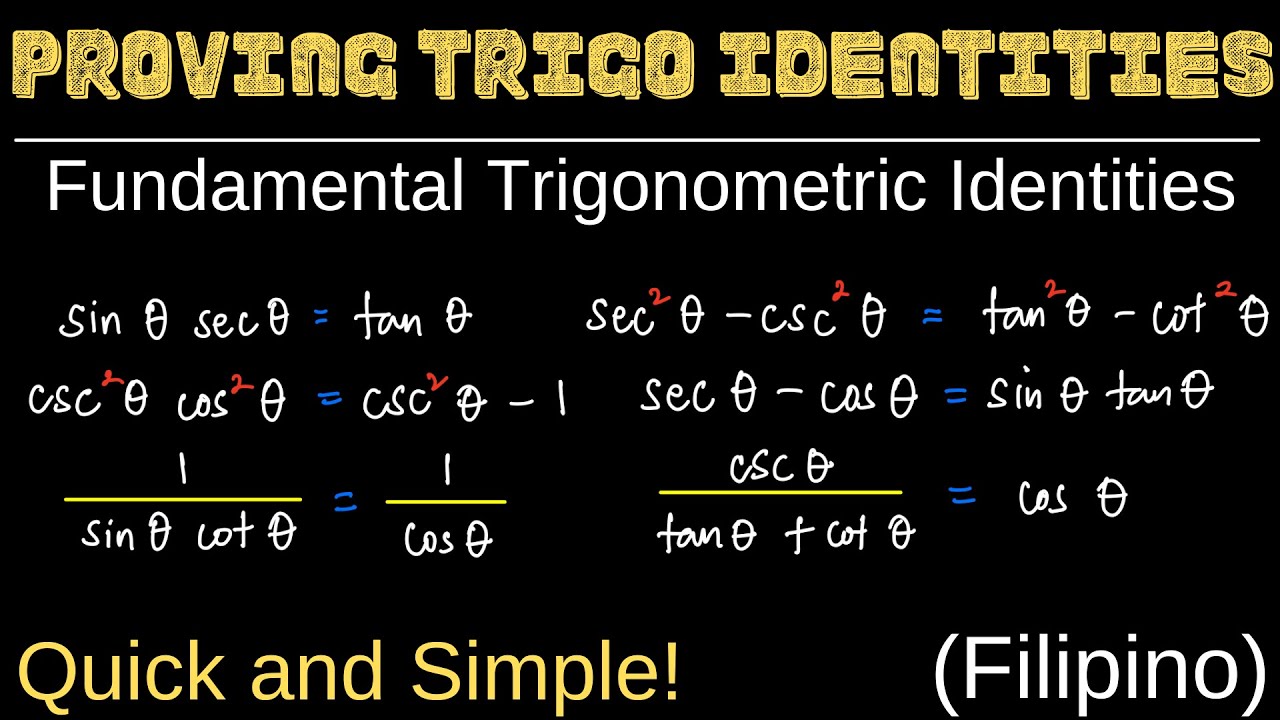
Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Sample Problems

[Math 20] Lec 3.4 Fundamental Identities (1 of 2)
Proving Trigonometric Identities (Tagalog/Flipino Math)
Super Hexagon for Trigonometric Identities | Trigonometry | Infinity Learn
5.0 / 5 (0 votes)
