Simplifying Trigonometric Expressions
Summary
TLDRIn this video, the process of simplifying trigonometric expressions using key identities is explained in detail. The video covers reciprocal identities such as secant, cosecant, tangent, and cotangent, along with essential Pythagorean identities. The script walks through various examples, demonstrating how to simplify complex trigonometric expressions step by step. These include replacing functions with their reciprocal identities, applying Pythagorean identities, and performing algebraic simplifications to reach simpler expressions. The video is a valuable resource for anyone looking to master the simplification of trigonometric functions in mathematical problems.
Takeaways
- 😀 Always keep key trigonometric identities in mind, such as reciprocal identities (secant, cosecant, etc.) and Pythagorean identities (like sin² + cos² = 1).
- 😀 Secant (sec) is equal to 1 over cosine, and cosecant (csc) is equal to 1 over sine. These are important for simplifying expressions.
- 😀 Tangent (tan) is the ratio of sine to cosine, and cotangent (cot) is the ratio of cosine to sine. These are essential for rewriting expressions.
- 😀 Use the Pythagorean identities to simplify expressions, such as sin² + cos² = 1, 1 + tan² = sec², and 1 + cot² = csc².
- 😀 In simplifying expressions, you may need to cancel out common factors like cosine or sine terms that appear in both the numerator and denominator.
- 😀 When simplifying a complex expression, consider breaking it into smaller parts and applying identities to make it easier to solve.
- 😀 For expressions like sec² times (1 - sin²), recognize that 1 - sin² can be replaced with cos², making the expression easier to simplify.
- 😀 Convert terms into single fractions, and when necessary, find common denominators to combine them into simpler expressions.
- 😀 Recurring patterns, like 1 + tan² = sec² or 1 + cot² = csc², are helpful for simplifying and reducing trigonometric expressions to their simplest form.
- 😀 Simplification often involves canceling out common terms and replacing complex fractions with simpler expressions, sometimes using reciprocal identities.
Q & A
What are the reciprocal identities mentioned in the video?
-The reciprocal identities are: secant = 1/cosine, cosecant = 1/sine, tangent = sine/cosine, and cotangent = cosine/sine.
What are the three Pythagorean identities mentioned in the video?
-The three Pythagorean identities are: sine² + cosine² = 1, 1 + tangent² = secant², and 1 + cotangent² = cosecant².
How is secant simplified in the example with secant * sine / cosine?
-Secant is simplified using the reciprocal identity secant = 1/cosine. The expression then becomes sine / cosine, which is equivalent to tangent.
What is the final simplification of the expression tangent² + sine² + cosine²?
-Using the Pythagorean identity sine² + cosine² = 1, the expression simplifies to tangent² + 1, which is equivalent to secant².
How do you simplify sine² * cosecant * secant?
-First, apply the reciprocal identities: cosecant = 1/sine and secant = 1/cosine. This simplifies the expression to sine * (1/sine) * (1/cosine), which reduces to tangent.
What simplification occurs in the expression cotangent * tangent + cotangent?
-First, cotangent is written as cosine/sine and tangent as sine/cosine. After simplifying the terms, the expression becomes 1 + cotangent², which equals cosecant².
How is the expression secant² * (1 - sine²) simplified?
-The expression secant² * (1 - sine²) simplifies by using the Pythagorean identity sine² + cosine² = 1. Replacing 1 - sine² with cosine² results in secant² * cosine², which simplifies to 1.
What simplification occurs in the expression cosine + sine * tangent?
-By substituting tangent as sine/cosine, and then combining terms with a common denominator, the expression simplifies to secant.
What is the final simplification of secant * sine + cotangent * tangent?
-The expression secant * sine + cotangent * tangent simplifies to 1 using the reciprocal and Pythagorean identities.
Why is it important to remember the Pythagorean and reciprocal identities when simplifying trigonometric expressions?
-These identities provide essential relationships that allow you to simplify complex trigonometric expressions by substituting equivalent terms and canceling out factors, making it easier to solve or simplify problems.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
Proving Trigonometric Identities (Tagalog/Flipino Math)

Identitas Trigonometri | Matematika Wajib Kelas X

IDENTITAS TRIGONOMETRI
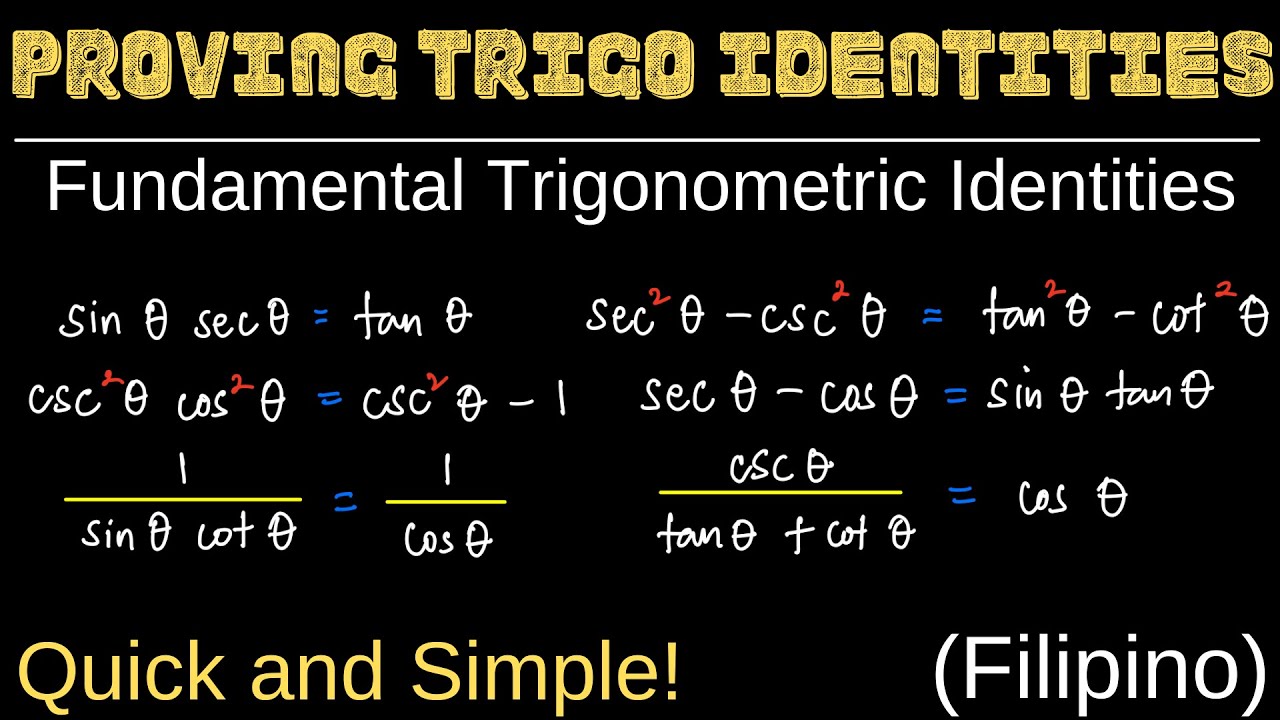
Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Sample Problems

Soal-soal Identitas Trigonometri
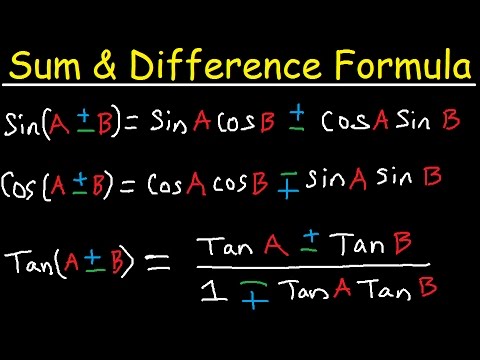
Sum and Difference Identities & Formulas - Sine, Cosine, Tangent - Degrees & Radians, Trigonometry
5.0 / 5 (0 votes)