Combined Variation - Equation and Constant of Variation - Grade 9 Math
Summary
TLDRIn this educational video, the concept of combined variation is explored, explaining how variables can vary both directly and inversely with one another. The video covers the mathematical formulas and processes for solving combined variation problems, including translating word problems into equations. Through practical examples, such as how resistance varies with length and diameter, viewers learn how to derive the constant of variation and apply it to real-world scenarios. The video also provides step-by-step instructions for solving these problems, making complex concepts accessible for students.
Takeaways
- 😀 Combined variation describes the relationship between three or more variables that vary directly and inversely with one another.
- 😀 In combined variation, variables can vary both directly (one increases as another increases) and inversely (one decreases as another increases).
- 😀 The general form for combined variation is Z = KX/Y, where Z varies directly as X and inversely as Y, and K is the constant of variation.
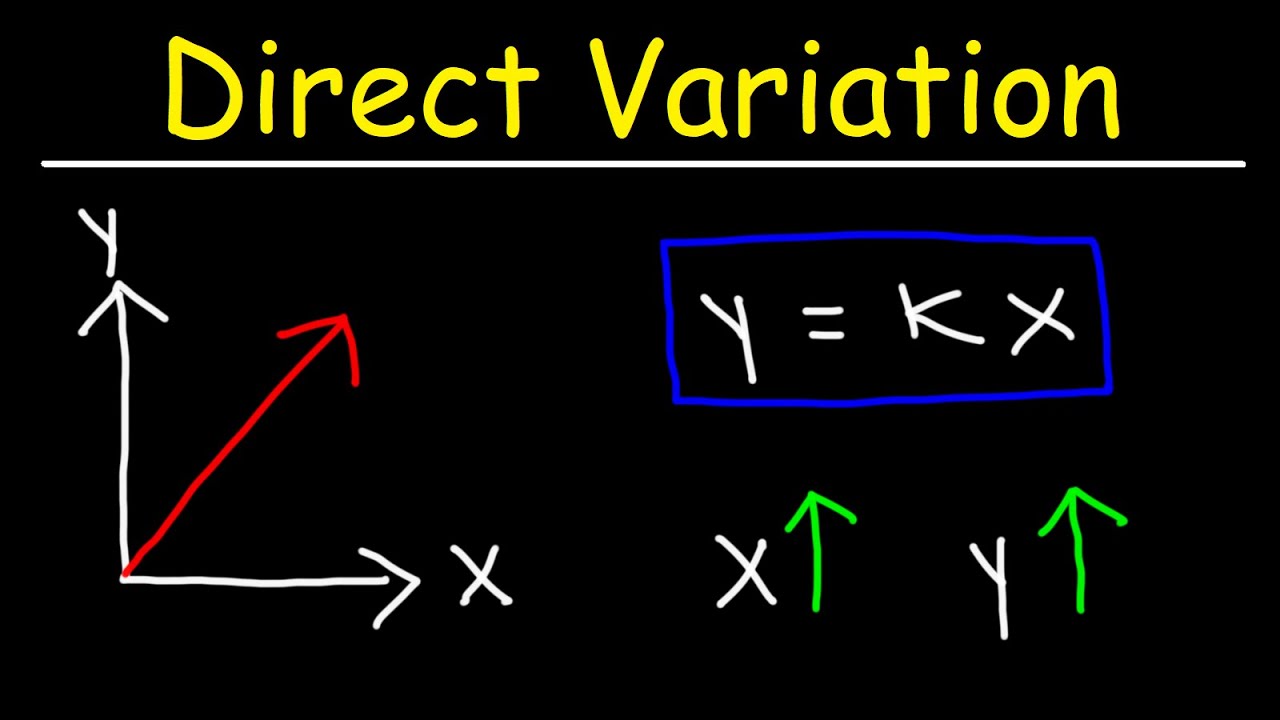
- 😀 Direct variation means that when one variable increases, another variable also increases, and is represented by the formula Z = KX.
- 😀 Inverse variation means that when one variable increases, another variable decreases, and is represented by the formula Z = K/Y.
- 😀 Joint variation is an extension of direct variation, where a variable depends on more than one other variable. For example, W varies jointly as C and the square of A and inversely as B.
- 😀 In the formula for joint variation, the variables in the numerator vary directly, and the variables in the denominator vary inversely.
- 😀 To find the constant of variation (K), substitute known values of the variables into the formula and solve for K.
- 😀 After finding K, you can use it to solve for unknown values in other combined variation problems.
- 😀 Example 1: W varies jointly as C and A^2 and inversely as B, which translates to the formula W = kCA^2/B.
- 😀 Example 2: The electrical resistance R varies directly as the length L and inversely as the square of the diameter D, represented by R = kL/D^2.
Q & A
What is combined variation?
-Combined variation describes a relationship between three or more variables that vary directly and inversely with one another. It combines both direct and inverse variations, allowing us to handle more complex relationships than simple direct or inverse variations alone.
How is combined variation different from direct and inverse variation?
-In direct variation, one variable increases or decreases in proportion to another. In inverse variation, one variable increases as the other decreases. Combined variation, however, involves both direct and inverse relationships simultaneously, with more than two variables involved.
What does it mean for a variable to vary directly as another variable?
-When a variable varies directly as another, it means that as one variable increases, the other also increases, or as one decreases, the other decreases, in a constant ratio. For example, if Z varies directly as X, the equation would be Z = K * X, where K is the constant of variation.
What does it mean for a variable to vary inversely as another?
-When a variable varies inversely as another, it means that as one variable increases, the other decreases, or vice versa, in a constant ratio. For example, if Z varies inversely as Y, the equation would be Z = K / Y, where K is the constant of variation.
How do you write a mathematical expression for combined variation?
-In combined variation, the expression combines both direct and inverse variations. For example, if Z varies directly as X and inversely as Y, the equation would be Z = (K * X) / Y, where K is the constant of variation.
What is the formula for the constant of variation K?
-The constant of variation K can be found by rearranging the combined variation formula. For example, in the equation Z = (K * X) / Y, solving for K gives K = (Z * Y) / X.
How do you solve for the constant of variation in an example problem?
-To solve for K, you substitute known values for the variables into the combined variation equation and solve for K. For instance, if Z = 3, X = 10, and Y = 4, you can plug these values into the equation Z = (K * X) / Y and solve for K by cross-multiplying.
What does 'jointly' mean in the context of combined variation?
-In combined variation, 'jointly' refers to direct variation involving more than one variable. For example, if W varies jointly as C and the square of A, the equation would be W = (K * C * A^2) / B, where C and A are both directly proportional to W.
How do you translate a word problem into a mathematical equation for combined variation?
-To translate a word problem, identify the direct and inverse relationships between variables. Then, write an equation where the variables involved are expressed in terms of direct and inverse variations. For example, if X varies directly as Y and inversely as Z, the equation is X = (K * Y) / Z.
Can you provide a step-by-step example of solving a combined variation problem?
-Sure! For example, if X varies directly as Y and inversely as Z, and you are given X = 10, Y = 4, and Z = 3, follow these steps: 1) Write the equation X = (K * Y) / Z. 2) Substitute the known values: 10 = (K * 4) / 3. 3) Solve for K by cross-multiplying: K = 30 / 4 = 15/2. 4) To find X when Y = 4 and Z = 3, substitute the values back into the equation and solve for the unknown variable.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード5.0 / 5 (0 votes)