SISTEM PERTIDAKSAMAAN LINEAR DUA VARIABEL SPtLDV
Summary
TLDRThis educational video explains how to solve systems of linear inequalities in two variables using graphical methods. The process involves plotting each inequality's graph, shading the appropriate region based on the inequality signs (e.g., 'less than' or 'greater than'), and identifying the solution area as the unshaded region. The video walks through examples to help viewers understand the steps clearly and demonstrates how to determine the solution region for various systems of inequalities. Ideal for students learning to graph and solve linear inequalities, the video emphasizes visual methods for clarity and precision.
Takeaways
- 😀 Linear inequalities in two variables involve two or more inequalities, each with two variables.
- 😀 The solution to a system of linear inequalities is the region where all inequalities are satisfied simultaneously.
- 😀 To graph the system, first plot the boundary lines by converting the inequalities to equalities.
- 😀 The shading on the graph helps identify the solution region: the correct side of the line is shaded based on the inequality's sign.
- 😀 If the inequality is 'less than or equal to' (≤), the solution lies below or to the left of the boundary line.
- 😀 If the inequality is 'greater than or equal to' (≥), the solution lies above or to the right of the boundary line.
- 😀 The feasible region is where all inequalities overlap and is the area not shaded in some cases.
- 😀 When plotting the boundary line, if x = 0, solve for y, and if y = 0, solve for x to find the intercepts.
- 😀 For inequalities like x + 2y ≤ 10, the process involves finding the intercepts, graphing, and shading the appropriate region.
- 😀 Practice examples are crucial for understanding graphing systems of inequalities and identifying the solution regions.
Q & A
What is a system of linear inequalities in two variables?
-A system of linear inequalities in two variables consists of two or more inequalities, each involving two variables such as 'x' and 'y'. The solution is represented by the regions on a graph that satisfy all the inequalities simultaneously.
How do you graph a linear inequality on a Cartesian plane?
-To graph a linear inequality, first graph the boundary line by setting the inequality equal to an equation (e.g., 'x + 2y = 10'). Then, identify the points where the line intersects the x-axis and y-axis. Next, shade the region that satisfies the inequality based on whether the inequality is 'greater than' or 'less than'.
What is the difference between a 'clean' and 'shaded' region when solving inequalities?
-The 'clean' region represents the solution area, where no shading occurs, while the 'shaded' region represents areas that do not satisfy the inequality. The 'clean' region is where all inequalities overlap and the solution exists.
Why do we shade areas above or below the line in linear inequalities?
-Shading above or below the line in linear inequalities depends on the inequality sign. If the inequality is 'greater than' (e.g., 'y > 2x + 5'), the region above the line is shaded. If it's 'less than' (e.g., 'y < 2x + 5'), the region below the line is shaded.
What does the intersection of two lines represent in a system of linear inequalities?
-The intersection of two lines in a system of linear inequalities represents a point where both inequalities are satisfied. The area around the intersection, where all conditions are met, is the solution to the system.
How do you determine which side of the line to shade when solving an inequality?
-To determine which side to shade, consider the direction of the inequality. If the inequality is 'less than' or 'less than or equal to', shade the side of the line where the region is below it. If the inequality is 'greater than' or 'greater than or equal to', shade the region above the line.
In the example 'x + 2y ≤ 10', how do we find the intercepts?
-To find the intercepts, set 'y = 0' to find the x-intercept and 'x = 0' to find the y-intercept. For 'x + 2y = 10', the x-intercept is (10, 0) and the y-intercept is (0, 5).
What is the significance of shading the 'incorrect' region when solving inequalities?
-Shading the incorrect region helps to identify which areas do not satisfy the inequality. This ensures that only the correct solution region, where all conditions are met, remains unshaded or 'clean'.
In the second example '2x + 3y ≤ 12', how do you graph the inequality?
-First, find the intercepts by setting 'x = 0' to get the y-intercept (0, 4) and 'y = 0' to get the x-intercept (6, 0). Then, draw the line connecting these points and shade the region that satisfies the inequality, which is below the line.
What does it mean when an inequality is written with '≥' or '≤' in a system of linear inequalities?
-When an inequality is written with '≥' or '≤', it indicates that the boundary line is included in the solution. This means that points on the boundary line also satisfy the inequality, and the shading includes the line itself.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

ILLUSTRATING LINEAR INEQUALITIES IN TWO VARIABLES || GRADE 8 MATHEMATICS Q2

PERSAMAAN LINEAR DUA VARIABEL (PLDV) KELAS 9
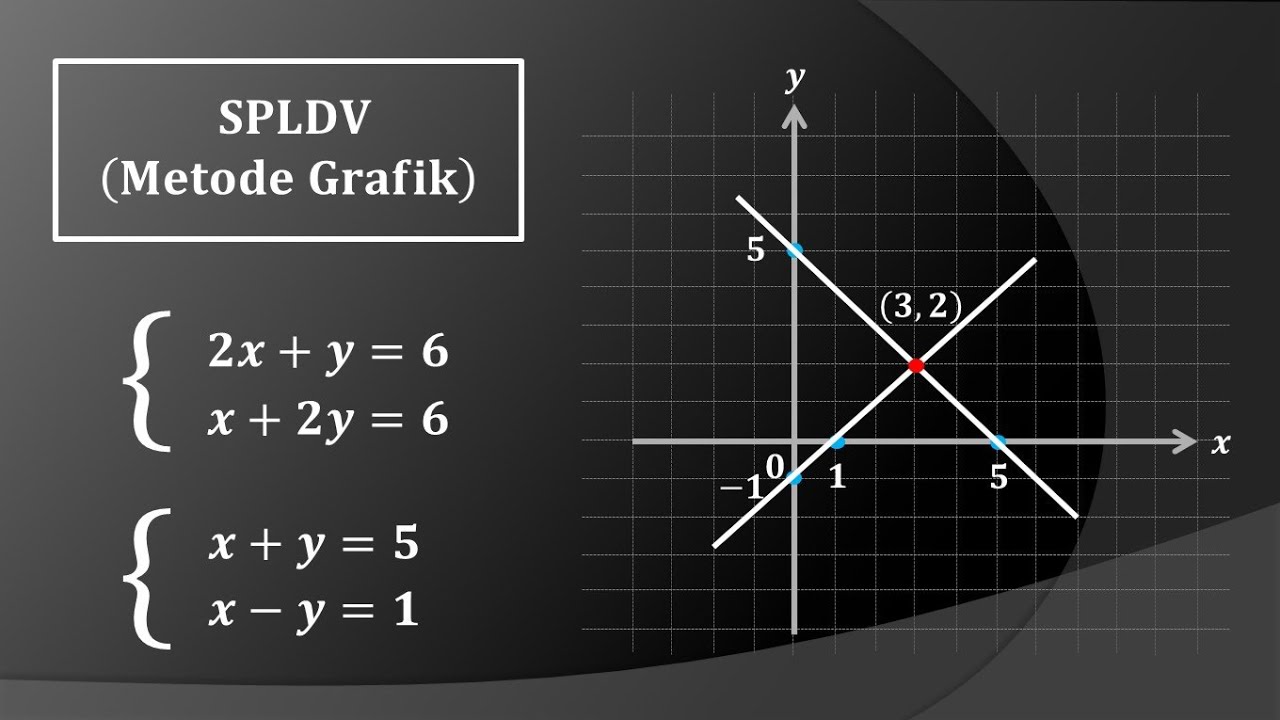
SPLDV (Sistem persamaan linear dua variabel) Metode grafik

SPLDV [Part 1] - Mengenal SPLDV + Metode Grafik
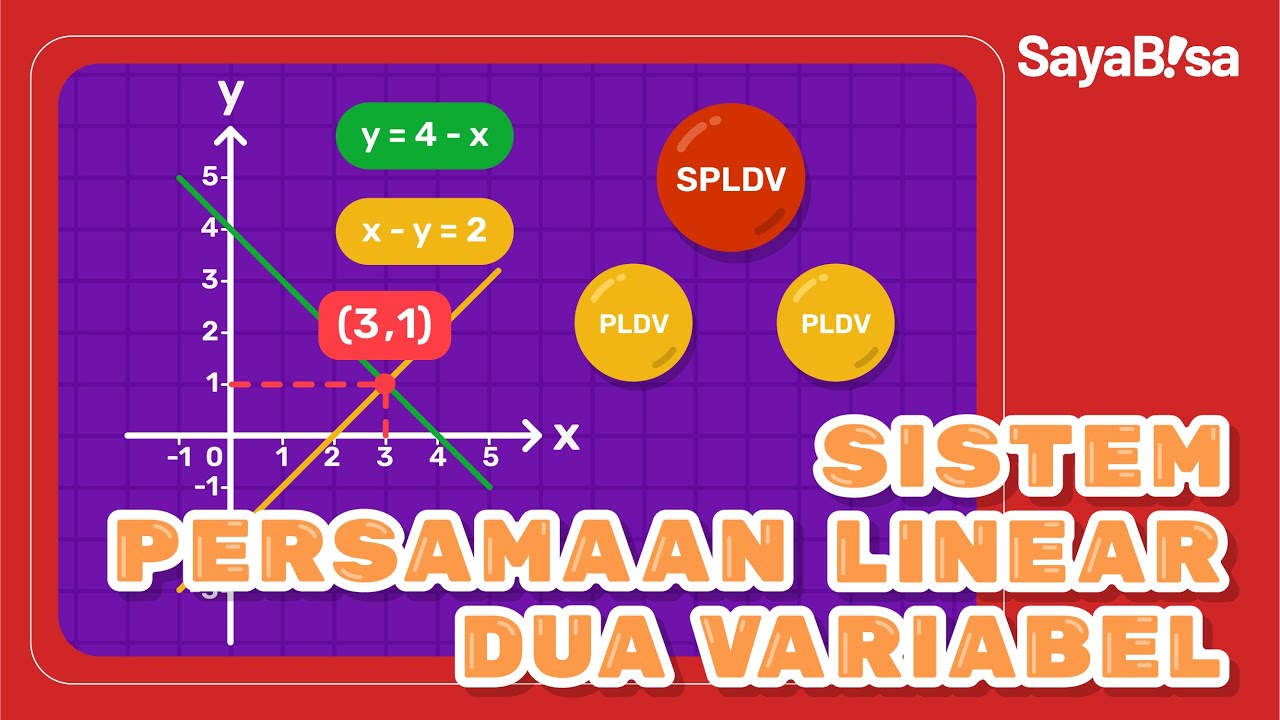
Sistem Persamaan Linear Dua Variabel | Matematika | SayaBisa

Sistem Pertidaksamaan Linear Dua Variabel | Pengetahuan Kuantitatif | Alternatifa
5.0 / 5 (0 votes)
