RUANG SAMPEL: Kaidah Penjumlahan dan Kaidar Perkalian dalam Mencacah Titik Sampel
Summary
TLDRThe video introduces key concepts in combinatorics, focusing on sample points and probabilities in different experiments. It explains two fundamental principles: the addition rule and the multiplication rule. Through examples involving coins, dice, and card sets (Gaple and Bridge), the speaker demonstrates how to calculate the total number of possible outcomes in various scenarios. The video emphasizes that performing all experiments results in more sample points than selecting just one experiment. It concludes with practical applications of these rules in probability, encouraging viewers to share the knowledge.
Takeaways
- 😊 The video introduces combinatorial analysis and explains its importance in determining possible outcomes in sample spaces.
- 🤔 Two fundamental principles in combinatorics are explained: the addition rule and the multiplication rule.
- 💡 The addition rule applies when only one experiment is selected from multiple possible experiments.
- 🔄 The multiplication rule applies when all experiments are conducted, and the total outcomes are the product of individual outcomes.
- 🪙 An example using a coin toss demonstrates the two possible outcomes (heads or tails) in a single trial.
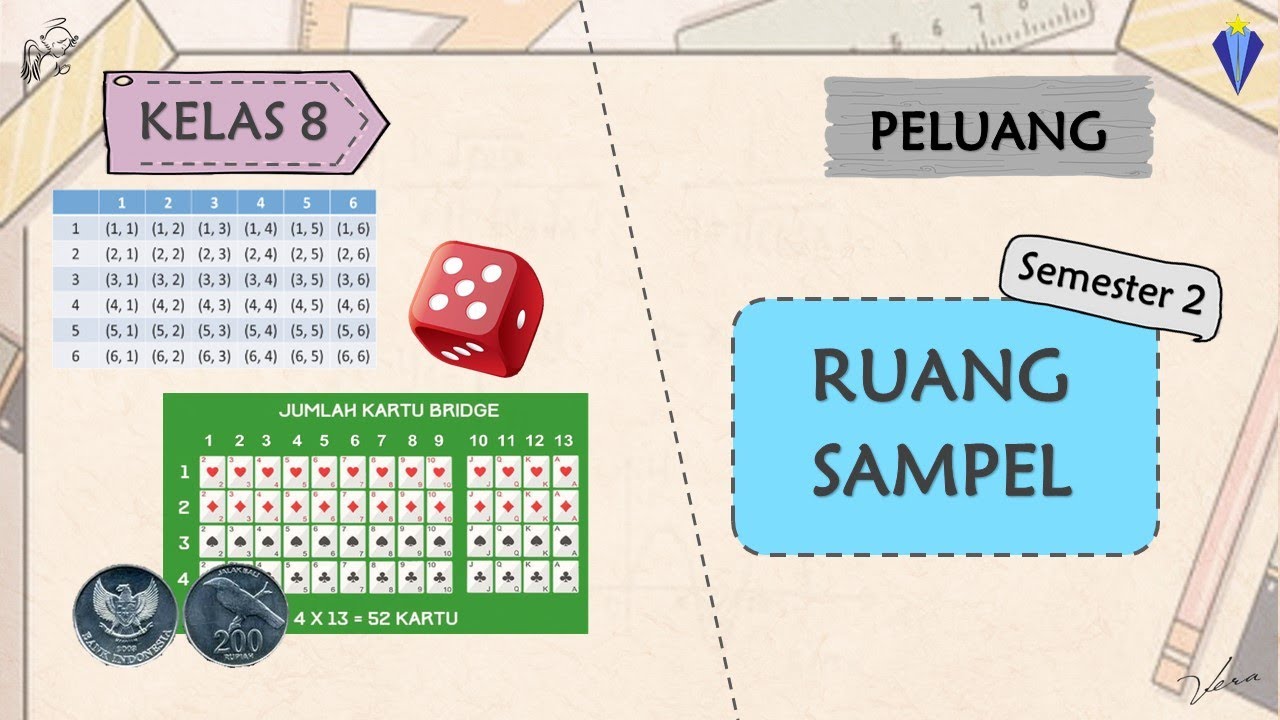
- 🎲 Another example with dice explains how multiple trials can produce a larger number of possible outcomes.
- 🃏 A card game example compares the number of possible outcomes from two decks: a gaple card set (28 outcomes) and a bridge card set (52 outcomes).
- 🧠 The total number of outcomes when combining multiple experiments (such as dice rolls and card draws) is much larger than from a single experiment.
- 🔢 The video shows how multiplying the possible outcomes of each experiment (e.g., 28 gaple cards and 52 bridge cards) gives a total of 1,456 outcomes.
- 📚 The lesson emphasizes understanding when to use the addition rule versus the multiplication rule in combinatorial problems.
Q & A
What are the two main principles discussed in combinatorial analysis?
-The two main principles discussed are the addition principle and the multiplication principle.
When is the addition principle used in combinatorics?
-The addition principle is used when only one of several possible experiments is chosen. The total number of outcomes is the sum of the possible outcomes from each experiment.
How is the multiplication principle applied in determining outcomes?
-The multiplication principle is applied when all experiments are conducted. The total number of outcomes is the product of the possible outcomes from each experiment.
What example is used to explain the addition principle?
-An example involving choosing between flipping a coin or rolling a die is used. In this case, only one experiment is performed, so the number of outcomes is the sum of the possible outcomes from both experiments.
What is the total number of outcomes if two experiments (coin flip and dice roll) are conducted together?
-If both a coin flip (2 outcomes) and a dice roll (6 outcomes) are conducted together, the total number of outcomes is 12, calculated by multiplying 2 and 6.
How many possible outcomes are there when using a set of 'gaple' cards?
-There are 28 possible outcomes when using a set of 'gaple' cards.
How many possible outcomes are there when using a standard deck of 'bridge' cards?
-There are 52 possible outcomes when using a standard deck of 'bridge' cards.
What is the total number of possible outcomes when combining both 'gaple' and 'bridge' cards in one experiment?
-The total number of possible outcomes when combining 'gaple' (28 outcomes) and 'bridge' (52 outcomes) cards is 1,456, calculated by multiplying 28 and 52.
Why is the number of outcomes greater when performing all experiments compared to just one?
-The number of outcomes is greater when performing all experiments because the multiplication principle is applied, resulting in a product of all possible outcomes, unlike the addition principle which only sums outcomes from one selected experiment.
What is the key takeaway regarding the addition and multiplication principles in combinatorial analysis?
-The key takeaway is that the addition principle applies when choosing one experiment, while the multiplication principle applies when conducting all experiments, leading to a much larger number of possible outcomes.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード5.0 / 5 (0 votes)