PELUANG: MATEMATIKA KELAS 8 SMP

Summary

In this educational video, Ka Febri from Sigma SmartStudy teaches the basics of probability, including key concepts like experiments, sample spaces, sample points, and types of probability (empirical and theoretical). The video covers examples such as coin tosses and dice rolls, demonstrating how to calculate probabilities and work with different types of events like simple and compound events. Viewers are introduced to important terms such as complementary events and joint events, and learn how to calculate probabilities for different scenarios. The video encourages students to keep practicing and learning from anywhere.

Takeaways

• 😀 Understanding of basic probability terms: experiment, sample space, and sample points.
• 😀 An experiment is an action that leads to possible outcomes, like tossing a coin with two possibilities: heads or tails.
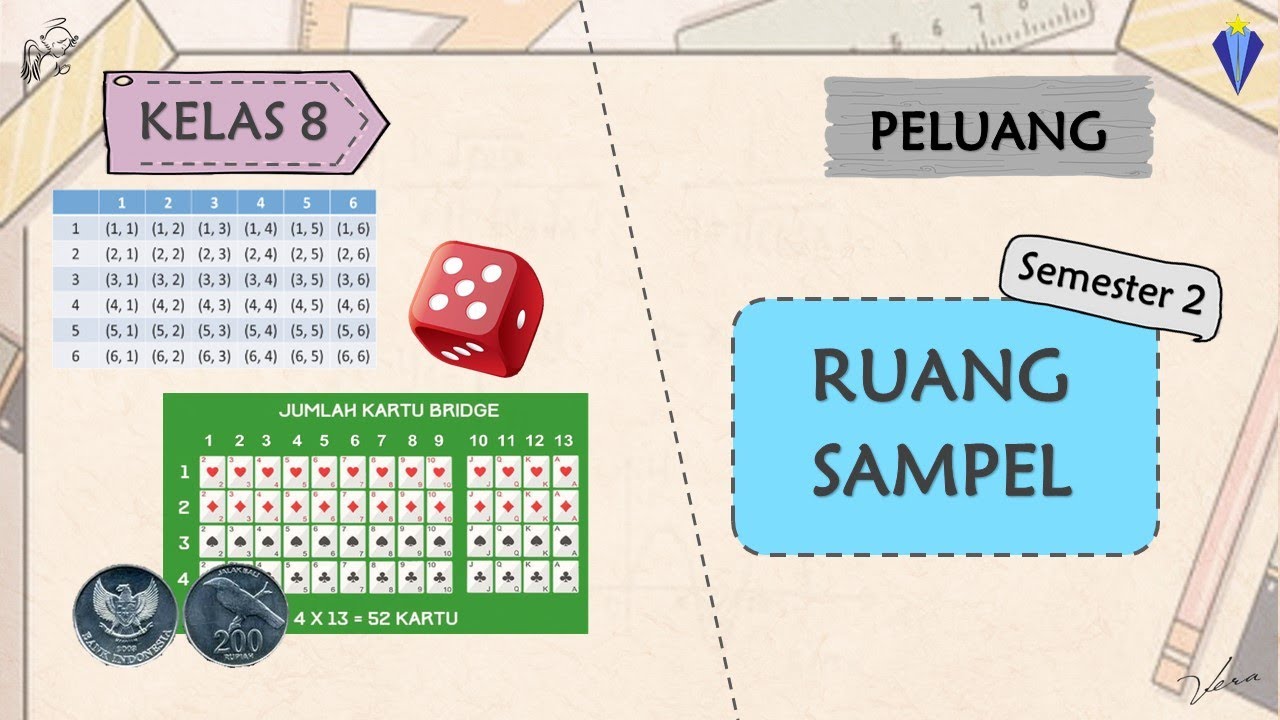
• 😀 Sample space (S) is the set of all possible outcomes in an experiment, such as {heads, tails} for a coin toss.
• 😀 A sample point refers to an individual outcome in the sample space, such as 'heads' or 'tails' in a coin toss.
• 😀 Different methods of listing sample points for experiments include direct listing, tree diagrams, and tables.
• 😀 Empirical probability refers to the probability based on actual experiments or trials, calculated as the ratio of favorable outcomes to total trials.
• 😀 Theoretical probability is based on possible outcomes calculated from a known sample space, such as rolling a fair die.
• 😀 The concept of events is explained, where events are subsets of a sample space, and can be simple or compound.
• 😀 The complement of an event refers to the probability that the event does not occur, and its probability is 1 minus the probability of the event.
• 😀 Compound events with union and intersection are discussed, including how to calculate probabilities for either-or (union) or both (intersection) events.
• 😀 The script also covers the calculation of probabilities for independent events, where the probability of both events occurring is the product of their individual probabilities.

Q & A

What is the definition of 'experiment' or 'trial' in probability?

An experiment or trial is an action or procedure that results in a set of possible outcomes. For example, tossing a coin is an experiment where the possible outcomes are 'heads' or 'tails'.

What is a 'sample space' in probability?

A sample space is the set of all possible outcomes of an experiment. For example, when tossing a coin, the sample space consists of two outcomes: 'heads' and 'tails'.

What does a 'sample point' represent in probability?

A sample point is an individual outcome within the sample space. In the example of tossing a coin, 'heads' and 'tails' are the sample points.

How can sample points be organized in a probability experiment?

Sample points can be organized using different methods, such as listing the outcomes directly, using a tree diagram, or creating a table that shows all possible outcomes.

What is empirical probability and how is it calculated?

Empirical probability, or experimental probability, is calculated by dividing the number of successful outcomes by the total number of trials. The formula is P(E) = n(E) / n(T), where n(E) is the number of successful outcomes and n(T) is the total number of trials.

Can you provide an example of calculating empirical probability?

For example, if a die is rolled 20 times and the number 1 appears 5 times, the empirical probability of rolling a 1 is 5/20 or 1/4.

What is the difference between theoretical and empirical probability?

Theoretical probability is based on the possible outcomes of an event based on mathematical reasoning, while empirical probability is based on the actual outcomes observed in an experiment.

What is a 'complementary event' in probability?

A complementary event is the event where the original event does not occur. For example, if the event is rolling a 5 on a die, the complementary event would be not rolling a 5.

What is the formula for calculating the probability of the complement of an event?

The probability of the complement of an event A is calculated as P(A') = 1 - P(A), where P(A') is the probability of the complementary event and P(A) is the probability of the event itself.

How are combined events with 'or' (union) and 'and' (intersection) different in probability?

When calculating the probability of combined events with 'or' (union), the formula is P(A ∪ B) = P(A) + P(B) - P(A ∩ B). For combined events with 'and' (intersection), the formula is P(A ∩ B) = P(A) * P(B) if the events are independent.

What is the formula for calculating the probability of two independent events occurring together?

The probability of two independent events A and B occurring together is calculated by multiplying their individual probabilities: P(A ∩ B) = P(A) * P(B).

What is the formula for calculating the probability of two mutually exclusive events occurring?

For two mutually exclusive events A and B, the probability of either A or B occurring is calculated by P(A ∪ B) = P(A) + P(B), since there is no overlap between the two events.

Can you explain how to calculate the probability of getting an even number on the first die and a prime number on the second die when rolling two dice?

To calculate the probability of rolling an even number on the first die (2, 4, or 6) and a prime number (2, 3, or 5) on the second die, you multiply the probabilities: P(even on first die) = 3/6 and P(prime on second die) = 3/6. The total probability is (3/6) * (3/6) = 9/36 or 1/4.