Pythagoras (1) - Pengenalan Teorema Pythagoras, Pythagoras Theorem - Matematika SMP
Summary
TLDRThis educational video introduces the Pythagorean theorem, exploring its history and various proofs. It demonstrates how to apply the theorem to solve problems involving right triangles, including practical scenarios like ladders against walls and ships' movements. The presenter encourages viewers to explore more proofs online and practice solving related problems for better understanding.
Takeaways
- 😀 The Pythagorean theorem is introduced, stating that in a right triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides.
- 🏛️ Pythagoras, the ancient Greek philosopher, is credited with the discovery of the theorem, although the concept predates him.
- 📚 There are numerous ways to prove the Pythagorean theorem, with one website reportedly listing a hundred different proofs.
- 🔍 To solve Pythagorean problems, it's important to identify the right triangle and its angles, and then apply the theorem accordingly.
- 📐 The script provides examples of solving for missing sides of a right triangle using the theorem, emphasizing the importance of recognizing the hypotenuse and the other sides.
- 📈 The transcript illustrates the process of solving Pythagorean problems step by step, including how to handle calculations and what to look for in the problem setup.
- 🌐 It mentions practical applications of the Pythagorean theorem, such as calculating the height of a ladder against a wall or the distance a ship has traveled.
- 🚢 A real-world problem involving a ship sailing east and then north is used to demonstrate the application of the theorem in calculating distances.
- 👨🏫 The script encourages viewers to practice solving Pythagorean problems and to explore the many available proofs to better understand and apply the theorem.
- 📺 The video is designed to be educational and engaging, with a call to action for viewers to like, subscribe, and share the content.
Q & A
What is the Pythagorean theorem?
-The Pythagorean theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
Who was Pythagoras and what is he known for?
-Pythagoras was an ancient Greek philosopher and the founder of the Pythagorean Brotherhood, known for the Pythagorean theorem which is a fundamental principle in Euclidean geometry.
How is the Pythagorean theorem applied in the context of the video?
-In the video, the Pythagorean theorem is applied to solve various mathematical problems involving right triangles by calculating the length of one side when the lengths of the other two sides are known.
What is the formula represented by 'bc² = ab² + AC²' in the context of the video?
-This formula represents the Pythagorean theorem where 'bc' is the hypotenuse, and 'ab' and 'AC' are the other two sides of a right-angled triangle.
Can you provide an example of how to solve a Pythagorean problem as described in the video?
-Sure, if you have a right triangle with sides of lengths 3 and 4, and you want to find the hypotenuse, you would calculate it as √(3² + 4²) = √(9 + 16) = √25, which equals 5.
What does the video suggest as an alternative to memorizing the Pythagorean theorem formula?
-The video suggests visualizing the triangle and its sides to understand the relationship between them, which can help in remembering and applying the theorem.
How many different proofs of the Pythagorean theorem are there according to the video?
-The video mentions a website that displays a hundred different proofs of the Pythagorean theorem, indicating there are numerous ways to prove it.
What is the significance of identifying the 'elbows' in a triangle when applying the Pythagorean theorem?
-Identifying the 'elbows' or right angles in a triangle helps to determine which sides are perpendicular and thus which sides can be used in the Pythagorean theorem to find the hypotenuse or one of the other sides.
How does the video approach solving story problems involving the Pythagorean theorem?
-The video suggests drawing the scenario to visualize the problem, identifying the right triangle, and then applying the Pythagorean theorem to find the unknown side.
Can you provide a real-world example given in the video where the Pythagorean theorem is applied?
-Yes, one example is a ladder leaning against a wall where the ladder's length, the distance from the ladder's base to the wall, and the height up the wall where the ladder touches are used to form a right triangle. The Pythagorean theorem is then used to calculate the height up the wall.
What is the final advice given in the video regarding solving Pythagorean problems?
-The video advises starting with the largest triangle when there are multiple triangles involved and to remember that the process involves looking at the 'elbows' or right angles to apply the theorem correctly.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

How many ways are there to prove the Pythagorean theorem? - Betty Fei

Teorema Pythagoras Kelas 8 Semester 2

TRIPEL PYTHAGORAS - TEOREMA PYTHAGORAS (3) - MATEMATIKA SMP KELAS 8

MATEMATIKA Kelas 8 - Teorema Phytagoras | GIA Academy
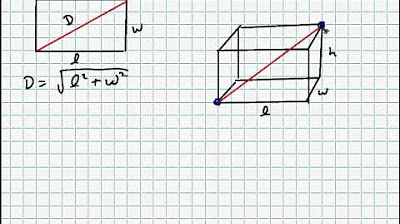
The Pythagorean Theorem: Extensions and Applications
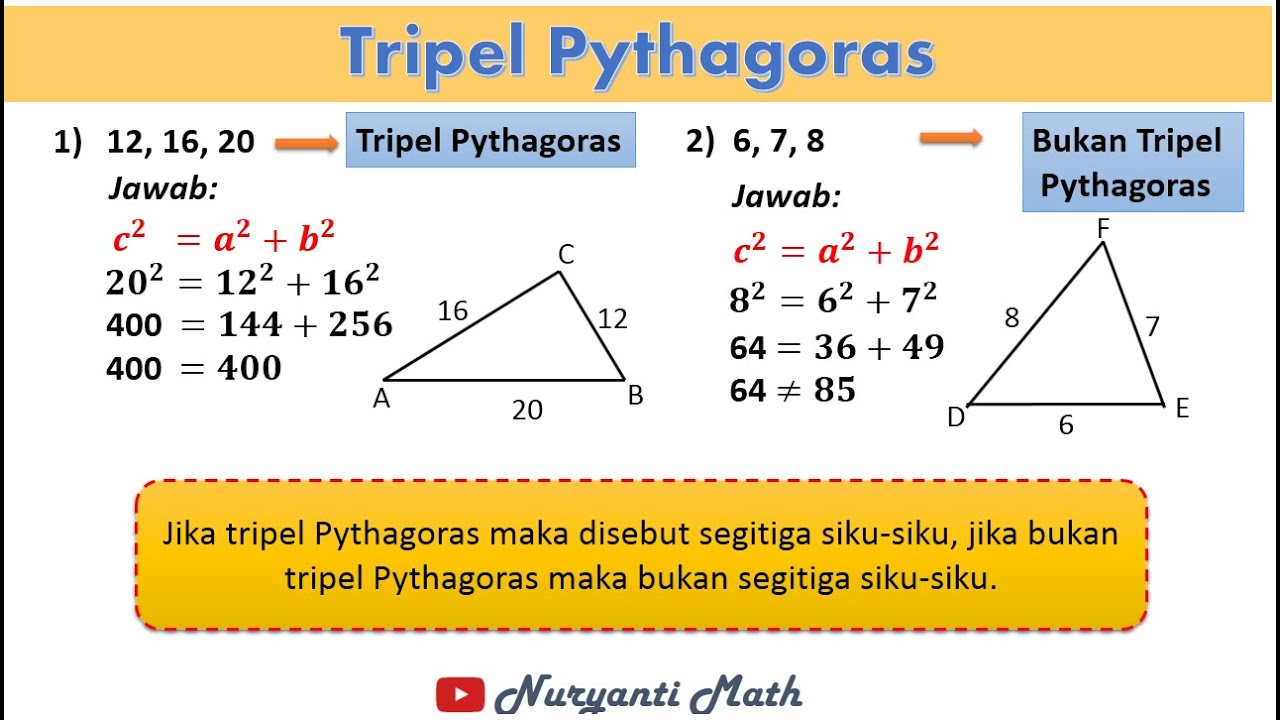
Tripel Pythagoras, Menentukan Jenis Segitiga - Matematika Kelas 8 SMP/MTs
5.0 / 5 (0 votes)
