Thermodynamics - A-level Physics
Summary
TLDRThis educational video script delves into the complexities of thermodynamics, focusing on the first law and its implications for gases. It explains the concepts of heat supply (Q), work done by gas (W), and internal energy changes. The script simplifies these ideas through discussions on isothermal and adiabatic processes, using the gas laws PV=nRT and PV relationships. It further illustrates these principles with PV diagrams, explaining how they represent different thermodynamic processes such as compression, combustion, and expansion in engines. The goal is to clarify thermodynamics for viewers, making it less daunting and more understandable.
Takeaways
- 🔍 The first law of thermodynamics is introduced as \( Q = \Delta U + W \), where \( Q \) is heat supplied to a gas, \( \Delta U \) is the change in internal energy, and \( W \) is the work done by the gas.
- 🌡️ The script explains that thermodynamics principles apply to gases, focusing on the relationship between heat, work, and internal energy.
- 🔥 Heat supplied to a gas (Q) increases the kinetic energy of its particles, but if the gas expands, it does work (W), not retaining all the supplied heat.
- 🔄 The concept of isothermal processes is discussed, where the temperature remains constant, and thus the internal energy doesn't change, implying all supplied heat is lost as work.
- 📉 In an isothermal process, the product of pressure and volume (PV) remains constant, as temperature dictates the relationship between these variables.
- 🔙 The script touches on adiabatic processes, where no heat is exchanged with the surroundings, leading to a change in internal energy equal to the negative work done by the gas.
- 🔢 The adiabatic constant, which is different for various gases, is introduced, showing how it affects the relationship between pressure and volume in adiabatic changes.
- ⚖️ Work done by or on a gas is defined as \( P \Delta V \), and the script clarifies that no work is done if the volume remains constant.
- 📈 The script describes how to represent thermodynamic processes on PV diagrams, illustrating isothermal and adiabatic changes, and how work is represented by the area under the curve.
- 🚗 The concept is applied to the operation of a four-stroke engine, detailing the intake, compression, combustion, power, and exhaust strokes, and how they relate to PV diagrams.
Q & A
What is the first law of thermodynamics?
-The first law of thermodynamics, also known as the law of energy conservation, states that the change in internal energy (ΔU) of a system is equal to the heat added to the system (Q) minus the work done by the system (W), expressed as ΔU = Q - W.
What does the term 'Q' represent in the context of thermodynamics?
-In thermodynamics, 'Q' represents the heat supplied to a system. It's the energy transferred to the system due to a temperature difference.
What is meant by 'W' in thermodynamics?
-In thermodynamics, 'W' stands for work done by the system. It refers to the energy transferred by the system as it expands or contracts, typically in relation to its surroundings.
Why does a gas do work when it expands?
-A gas does work when it expands because the kinetic energy of its particles increases as they move faster due to added heat. This increased kinetic energy is converted into mechanical work as the gas pushes against its surroundings.
What is an isothermal process in thermodynamics?
-An isothermal process is one in which the temperature of the system remains constant. In this process, any heat added to the system is entirely used to do work, resulting in no change in internal energy.
How does the relationship between pressure and volume change during an isothermal process?
-During an isothermal process, the relationship between pressure and volume is inversely proportional, as described by Boyle's Law, which states PV = constant.
What is an adiabatic process in thermodynamics?
-An adiabatic process is one in which there is no heat exchange with the surroundings, meaning Q = 0. In this process, the change in internal energy is equal to the negative of the work done by the system, ΔU = -W.
What is the significance of the adiabatic constant in thermodynamics?
-The adiabatic constant, often represented by the Greek letter gamma (γ), is used in the equation relating pressure, volume, and temperature during an adiabatic process. It varies depending on the type of gas and is crucial for calculating the work done or the change in temperature in adiabatic processes.
What does it mean when work is done by a gas at constant volume?
-When work is done by a gas at constant volume, it means that the volume does not change, and therefore, no work is being done on or by the gas. This situation is represented by W = 0 in the thermodynamic equations.
How is work done related to the pressure and volume of a gas?
-The work done by a gas is directly related to the pressure and volume changes. The work done (W) is equal to the pressure (P) times the change in volume (ΔV), expressed as W = PΔV.
What is a PV loop and why is it important in thermodynamics?
-A PV loop is a graphical representation of the cyclic processes that a gas undergoes, such as in an engine. It shows the relationship between pressure and volume at different stages of the cycle, and the area under the curve represents the work done by or on the gas during these processes.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示
12th Physics | Chapter 4 | Thermodynamics | Lecture 2 | Maharashtra Board |
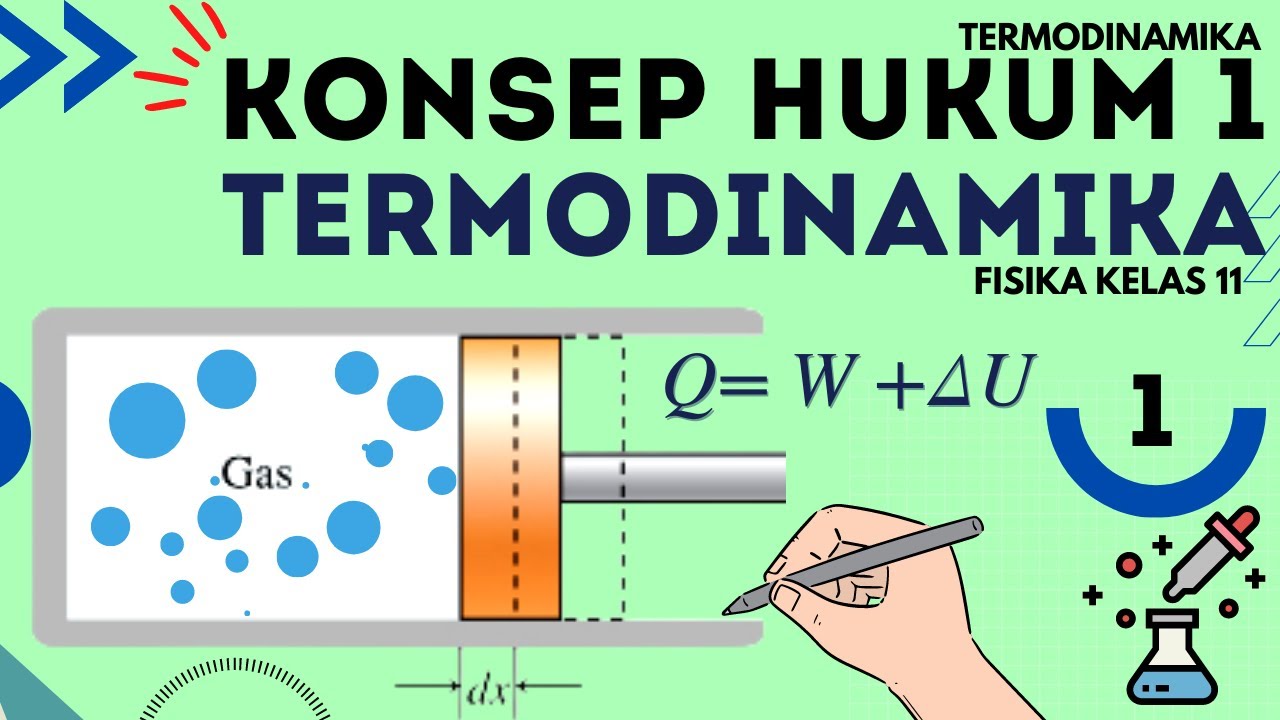
Hukum 1 Termodinamika : Termodinamika Fisika Kelas 11 - Part 1

VIDEO PEMBELAJARAN - FISIKA - HUKUM I TERMODINAMIKA

Lei dos Gases Ideais e o Ciclo de Carnot

Termodinamika • Part 1: Hukum I Termodinamika Isobarik Isokhorik Isotermik Adiabatik

Temperature: Crash Course Physics #20
5.0 / 5 (0 votes)
