Learn Fractions In 7 min ( Fast Review on How To Deal With Fractions)

Summary

This video aims to provide a quick review of fractions, covering basic concepts like mixed numbers, improper fractions, and proper fractions. It teaches how to convert between these forms, simplify fractions, and perform arithmetic operations like addition, subtraction, multiplication, and division. The presenter also introduces a shortcut method for adding and subtracting fractions, making it accessible for those struggling with fractions.

Takeaways

• 📚 This video aims to review basic fractions in seven minutes, covering mixed fractions, improper fractions, simplifying fractions, multiplication, division, addition, and subtraction.
• 🔢 Mixed fractions combine a whole number and a fraction, like 3 1/2, which can be converted to an improper fraction by multiplying the denominator with the whole number and adding the numerator (e.g., 3 1/2 = 7/2).
• ↔️ Improper fractions, where the numerator is larger than the denominator (e.g., 10/3), can be converted to mixed numbers by dividing the numerator by the denominator (e.g., 10/3 = 3 1/3).
• 📈 Proper fractions have a numerator smaller than the denominator (e.g., 1/2).
• ✂️ Simplifying fractions involves reducing them to their simplest form by dividing both the numerator and denominator by their greatest common divisor (e.g., 10/24 simplifies to 5/12).
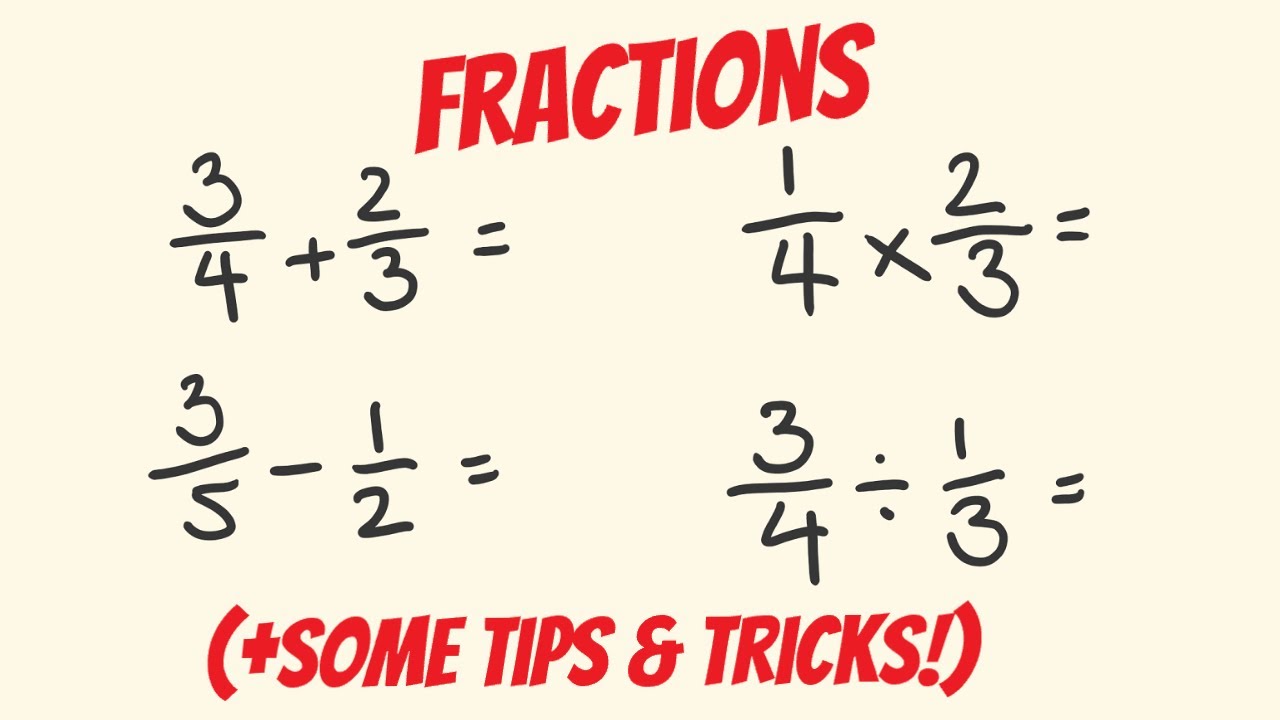
• ✖️ Multiplying fractions is straightforward: multiply the numerators and denominators respectively (e.g., 2/5 * 3/4 = 6/20, which simplifies to 3/10).
• ➗ Dividing fractions involves flipping the second fraction (reciprocal) and then multiplying (e.g., 3/8 ÷ 6/10 becomes 3/8 * 10/6 = 30/48, which simplifies).
• ➕➖ Adding and subtracting fractions can be simplified using the 'bowtie method': multiply diagonally for the numerators, and then multiply the denominators (e.g., 2/3 + 5/7 = (2*7 + 5*3) / (3*7) = 29/21).
• 🔄 The 'bowtie method' also applies to subtraction, following the same steps but using a subtraction operator (e.g., 3/8 - 1/4 = (3*4 - 1*8) / (8*4) = 20/32, which simplifies to 5/8).
• 🔄 Mixed number operations involve converting them to improper fractions first, then applying the appropriate operation (e.g., 3 1/2 ÷ 2 1/3 becomes 7/2 ÷ 7/3).

Q & A

What is the main goal of the video?

The main goal of the video is to help viewers understand fractions within about seven minutes, providing a quick review of the basic concepts and common problems related to fractions.

What is a mixed fraction?

A mixed fraction is a number that combines a whole number and a fraction, such as 3 1/2.

How can you convert a mixed fraction to an improper fraction?

To convert a mixed fraction to an improper fraction, multiply the whole number by the denominator of the fraction, then add the numerator. For example, for 3 1/2, you would calculate 2*3 + 1 = 7, resulting in the improper fraction 7/2.

What defines an improper fraction?

An improper fraction is a fraction where the numerator is larger than the denominator, such as 10/3.

How do you simplify a fraction?

To simplify a fraction, find the greatest common divisor (GCD) of the numerator and denominator and divide both by that number. For example, to simplify 10/24, divide both by 2 to get 5/12.

What is the method for multiplying fractions?

To multiply fractions, multiply the numerators together and the denominators together. For example, (2/5) * (3/4) = (2*3)/(5*4) = 6/20, which simplifies to 3/10.

How do you handle division of fractions?

To divide fractions, invert (flip) the second fraction and then multiply. For example, (3/8) ÷ (5/6) becomes (3/8) * (6/5) = (3*6)/(8*5) = 18/40, which simplifies to 9/20.

What is the 'bowtie' method mentioned in the video?

The 'bowtie' method is a technique for adding and subtracting fractions. It involves multiplying across the fractions in a crisscross pattern and then summing or subtracting the products, and finally multiplying the denominators for the new denominator.

Can you explain the 'bowtie' method with an example?

For example, to add 2/5 and 3/7 using the 'bowtie' method, calculate (2*7) + (5*3) for the numerator and (5*7) for the denominator. This gives (14 + 15)/35 = 29/35.

How can mixed numbers be handled in operations like division?

Mixed numbers can be converted into improper fractions before performing operations. For example, 3 1/2 ÷ 2 1/3 is converted to 7/2 ÷ 7/3, then solved by inverting the second fraction and multiplying, resulting in 21/14, which simplifies to 3/2.