Similar triangles to prove that the slope is constant for a line | Algebra I | Khan Academy
Summary
TLDRThis video script explores the concept of slope in algebra using geometry. It demonstrates that the slope of a line remains constant by proving the similarity of triangles formed between any two points on the line. The argument relies on the congruence of corresponding angles, established through the properties of parallel lines and transversals.
Takeaways
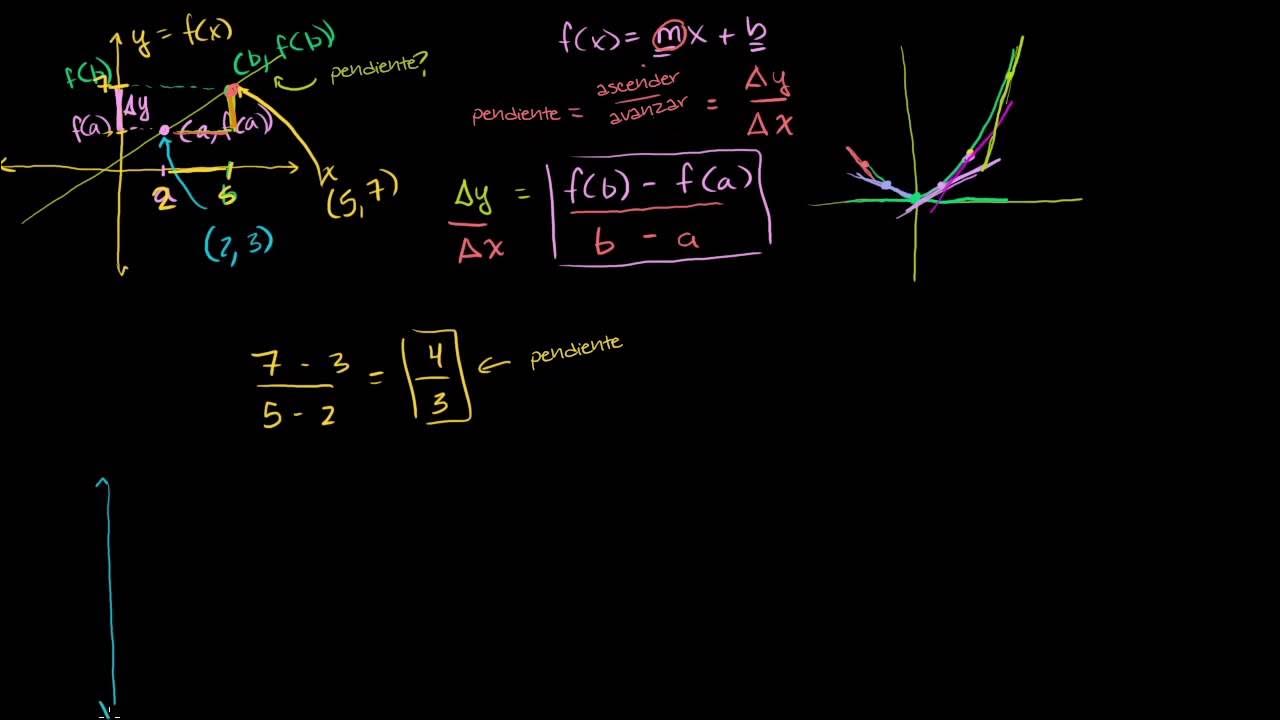
- 📚 The concept of a line in algebra is characterized by a constant rate of change of y with respect to x, which is also known as a constant slope.
- 📐 Slope is defined as the ratio of the change in y (delta y) to the change in x (delta x), indicating the line's inclination.
- 🔍 The video aims to prove the constancy of slope using similar triangles from geometry.
- 📏 Two sets of points are considered to demonstrate the change in x and y, highlighting how these changes relate to the slope.
- 🔄 The ratio of the change in y to the change in x must be the same for any two sets of points on a line to prove the slope's constancy.
- 🔺 The script introduces the idea of similarity in triangles, where corresponding angles must be equal or congruent for triangles to be considered similar.
- 🔶 Demonstrating similarity between two triangles allows for the establishment of a constant ratio between corresponding sides, which is crucial for proving the constant slope.
- 📏 The script uses the properties of parallel lines and transversals to show that the angles formed by the intersection of the line segments are congruent.
- 🔄 By establishing that the triangles formed by the line segments are similar, the script concludes that the ratio of the vertical to horizontal side lengths (the slope) is constant.
- 🔍 The constancy of the slope is proven by showing that any right triangles formed between points on the line are similar, thus maintaining a consistent ratio of side lengths.
Q & A
What is the primary concept discussed in the video?
-The primary concept discussed in the video is the constant rate of change or constant slope of a line in algebra, and how to prove it using similar triangles from geometry.
What is the Greek letter delta used to represent in the context of the video?
-In the context of the video, the Greek letter delta (Δ) is used as a shorthand for 'change in,' specifically representing the change in y (Δy) over the change in x (Δx).
What does the video aim to prove using similar triangles?
-The video aims to prove that the ratio of the change in y to the change in x (i.e., the slope) is constant for a line, using the concept of similar triangles in geometry.
How does the video define the slope of a line?
-The video defines the slope of a line as the ratio of the change in y (Δy) to the change in x (Δx), which is a constant value for a straight line.
What is the significance of the triangles being similar in the video's argument?
-The significance of the triangles being similar is that it allows the video to establish that the ratio of corresponding sides (which represents the slope) is the same for any two sets of points on the line, proving the constancy of the slope.
What are the conditions for two triangles to be considered similar?
-Two triangles are considered similar if all corresponding angles are congruent, or if the ratio of corresponding sides is the same.
How does the video use parallel lines and transversals to prove the similarity of triangles?
-The video uses the fact that the horizontal and vertical lines are parallel to each other and that the line connecting the points on the line acts as a transversal. This setup shows that corresponding angles are congruent, thus proving the similarity of the triangles.
What is the role of the horizontal and vertical lines in the video's argument?
-The horizontal and vertical lines serve as the basis for creating right triangles. They are parallel to each other, which allows the video to use the properties of parallel lines and transversals to prove the similarity of triangles formed by any two points on the line.
How does the video conclude that the slope is constant for a line?
-The video concludes that the slope is constant for a line by showing that any right triangles formed between points on the line are similar. Since the ratio of corresponding sides (the slope) is the same for similar triangles, the slope remains constant.
What is the practical application of understanding the constant slope of a line?
-Understanding the constant slope of a line is crucial in algebra and geometry as it helps in analyzing linear relationships, predicting outcomes in linear equations, and understanding the behavior of linear functions.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
Cálculo: Derivadas 1 (nueva versión HD)

Geometria analítica - Inclinação e coeficiente angular Equação da reta sabendo ponto e a declividade
Questão de Geometria Analítica - equação da reta
Greatest Math Theories Explained
The Pythagorean Theorem: Extensions and Applications

Average vs Instantaneous Rates of Change
5.0 / 5 (0 votes)
