EQUAÇÃO DA FUNÇÃO AFIM - EXERCÍCIO - Professora Angela Matemática
Summary
TLDRThis educational video explains how to find the equation of a linear function (first-degree function) passing through two given points. The instructor demonstrates how to use the point-slope form and the slope formula to derive the equation step by step. First, they use substitution to solve for the slope (a) and the y-intercept (b). Then, a second method involving the slope formula is presented, making the process quicker. The video ends with encouragement to visit the instructor's website for more math courses, especially for those struggling with fundamental math or preparing for public exams.
Takeaways
- 😀 The lesson discusses how to find the equation of an affine function (first-degree function) passing through specific points (2, 5) and (4, 11).
- 😀 The graph of an affine function is a straight line, and the goal is to determine its equation.
- 😀 The basic form of an affine function is given by f(x) = ax + b, where 'a' represents the slope and 'b' is the y-intercept.
- 😀 To find the equation, you substitute the given points into the function's equation to create two equations with 'a' and 'b' as unknowns.
- 😀 The script demonstrates solving the system of equations step-by-step to find the values of 'a' and 'b'.
- 😀 The value of 'a' (slope) is determined to be 3 through algebraic manipulation.
- 😀 Once 'a' is found, 'b' is calculated by substituting 'a' into one of the original equations.
- 😀 The final equation of the affine function is f(x) = 3x - 1, which matches one of the given alternatives.
- 😀 Another method to solve this problem is by calculating the slope (a) directly using the formula for the coefficient angular: a = (y2 - y1) / (x2 - x1).
- 😀 Once the slope 'a' is known, 'b' can be calculated by substituting the known point into the equation f(x) = ax + b.
- 😀 The lesson concludes by offering additional courses and encouraging viewers to engage with the content, providing links to further resources and a math course.
Q & A
What is the goal of the exercise presented in the video?
-The goal is to find the equation of an affine function (also known as a linear function or first-degree function) that passes through the points (2, 5) and (4, 11).
What is an affine function?
-An affine function is a first-degree function, which is represented by the equation f(x) = ax + b, where 'a' is the coefficient that determines the slope, and 'b' is the y-intercept.
How can we find the equation of the affine function from the two given points?
-We can find the equation by first determining the values of 'a' (slope) and 'b' (y-intercept). This can be done by substituting the coordinates of the two points into the equation f(x) = ax + b and solving for 'a' and 'b'.
What is the formula to calculate the slope (a) of an affine function?
-The formula to calculate the slope 'a' is: a = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are the coordinates of the two points through which the function passes.
How did the teacher find the value of 'a'?
-The teacher used the formula for the slope, substituting the points (2, 5) and (4, 11) into the formula: a = (11 - 5) / (4 - 2), which simplifies to a = 6 / 2 = 3.
After finding the slope 'a', how do we find 'b'?
-To find 'b', the teacher used one of the points (2, 5) and substituted the values into the equation f(x) = ax + b, where 'a' is already known to be 3. Solving the equation 5 = 3 * 2 + b, we get b = -1.
What is the final equation of the affine function?
-The final equation of the affine function is f(x) = 3x - 1.
What alternative method is mentioned for solving the problem?
-An alternative method involves using the formula for the slope (a) and then substituting the values into the equation f(x) = ax + b to find 'b'. This method is quicker and more straightforward than using a system of equations.
What is the benefit of using the coefficient of the slope (a) in this context?
-Using the coefficient of the slope (a) makes it easier to determine the steepness of the line and simplifies the process of finding the equation of the affine function.
What other courses does the teacher offer in the video?
-The teacher offers courses for students in elementary and high school, as well as courses for those preparing for public exams. The courses focus on teaching mathematics in a more practical and accessible way.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

RÁPIDO e FÁCIL | COMO CONSTRUIR GRÁFICO DA FUNÇÃO DO 1º GRAU

FUNÇÃO DO 1º GRAU | COMO CONSTRUIR GRÁFICO | RAIZ DA FUNÇÃO
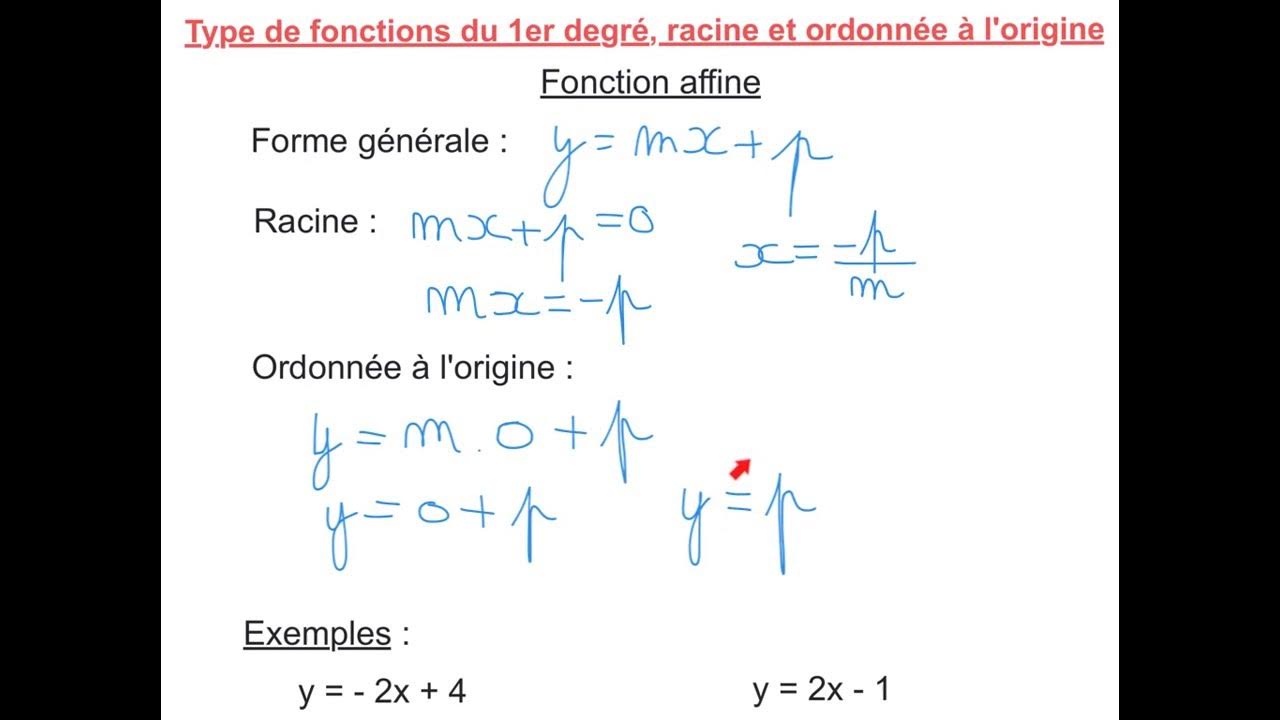
13.2 Fonctions du 1er degré , racine et ordonnée à l'origine

FUNÇÃO AFIM - EXERCÍCIOS # 1
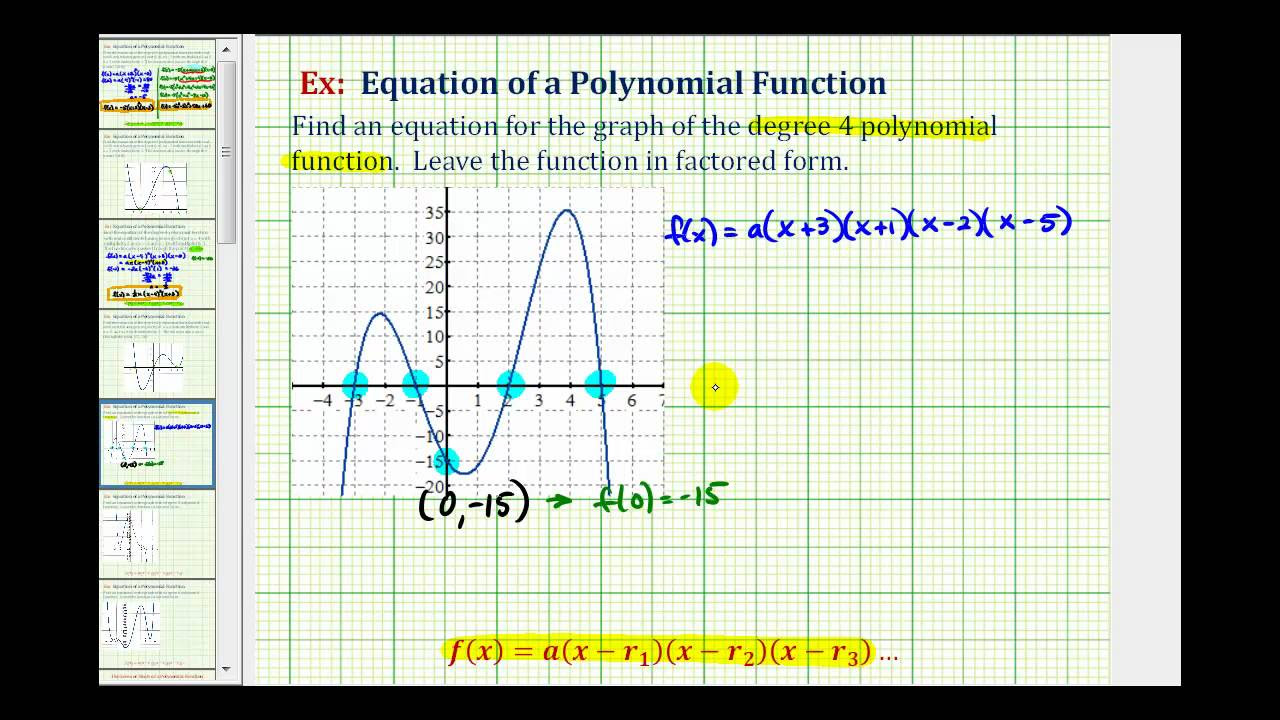
Ex1: Find an Equation of a Degree 4 Polynomial Function From the Graph of the Function
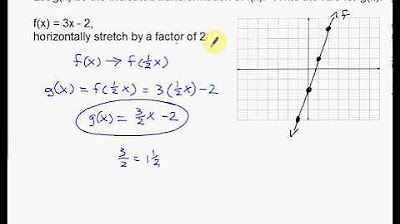
Linear Transformations Vertical and Horizontal Stretching and Compressing Examples
5.0 / 5 (0 votes)
