Ruang Sampel dan Peluang Suatu Kejadian (Materi dan Contoh Soal diserta Pembahasan)

Summary

In this video, the presenter explains fundamental concepts in probability, starting with sample spaces and the likelihood of different outcomes. Using practical examples such as flipping coins, rolling dice, and tossing multiple coins, the presenter illustrates how to determine sample spaces and calculate the probability of specific events. The video also covers the calculation of probabilities for events like rolling an odd number on a die or getting one head and two tails when tossing three coins. Throughout, the presenter emphasizes the importance of understanding these basic principles to solve probability problems.

Takeaways

• 😀 Sample space refers to the set of all possible outcomes of an experiment.
• 😀 Example 1: Tossing a coin has two possible outcomes: heads or tails.
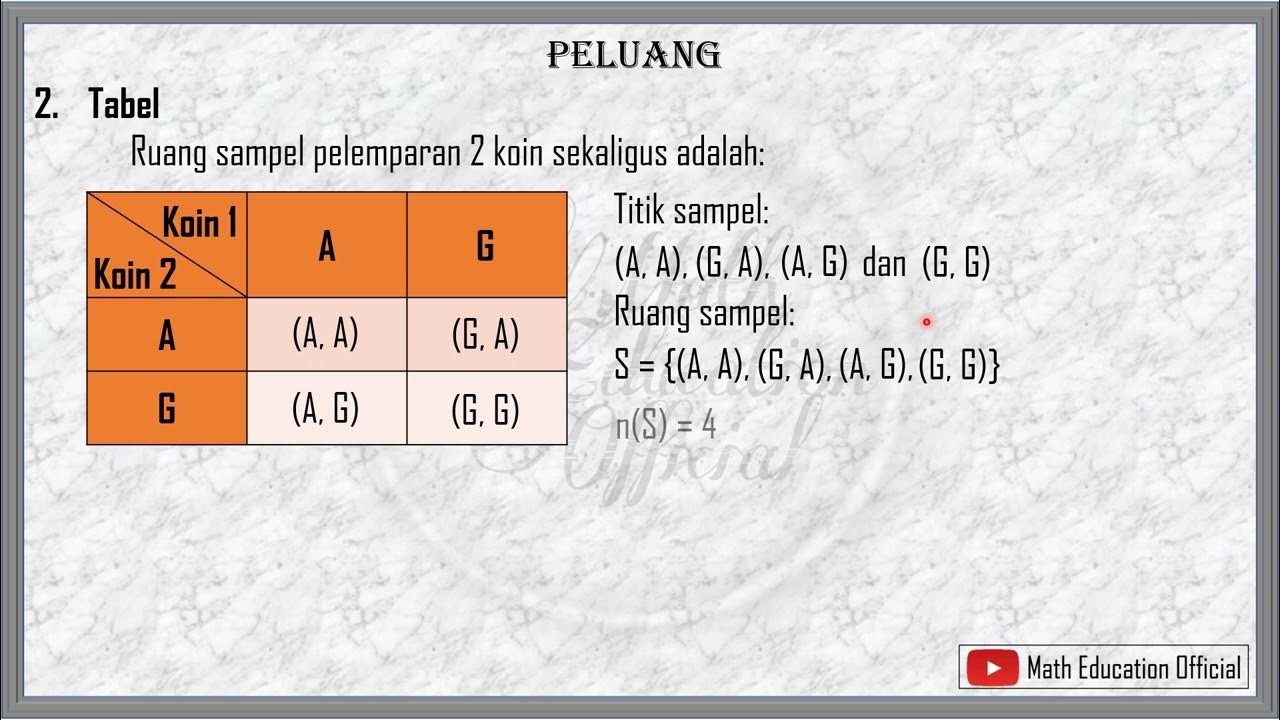
• 😀 Example 2: Tossing two coins results in four possible outcomes: HH, HT, TH, TT.
• 😀 Example 3: Tossing three coins results in eight possible outcomes, e.g., HHH, HHT, TTT.
• 😀 Rolling a die has six possible outcomes: 1, 2, 3, 4, 5, 6.
• 😀 Rolling two dice results in 36 possible outcomes, with combinations like (1,1), (1,2), ..., (6,6).
• 😀 Probability is calculated by dividing the number of favorable outcomes by the total number of outcomes in the sample space.
• 😀 Example 1: The probability of rolling an odd number on a die is 1/2, as there are 3 favorable outcomes (1, 3, 5) out of 6.
• 😀 Example 2: The probability of getting exactly one head and two tails when tossing three coins is 3/8.
• 😀 Example 3: The probability of rolling a prime number on a die (2, 3, or 5) and getting heads on a coin is 1/4.
• 😀 The value of probability always ranges from 0 (impossible event) to 1 (certain event).

Q & A

What is the sample space in probability?

The sample space refers to the set of all possible outcomes in an experiment. For example, when flipping a coin, the sample space consists of two outcomes: heads (gambar) and tails (angka).

How do we determine the sample space for multiple coin tosses?

For two coin tosses, the sample space consists of four possible outcomes: (gambar, gambar), (gambar, angka), (angka, gambar), and (angka, angka). Similarly, for three coin tosses, the sample space will have eight possible outcomes.

What is the sample space for rolling a single die?

The sample space for rolling a single die is the set of all possible outcomes: {1, 2, 3, 4, 5, 6}.

How do we determine the sample space for two dice rolls?

For two dice rolls, the sample space is determined by the possible outcomes of the first die and the second die. This results in 36 possible outcomes, as there are 6 possible outcomes for each die.

What is the probability of rolling an odd number on a die?

The probability of rolling an odd number on a die is calculated by dividing the number of favorable outcomes (1, 3, 5) by the total number of possible outcomes (6). Hence, the probability is 3/6 or 1/2.

How do we calculate the probability of getting one head and two tails when tossing three coins?

To calculate the probability of getting exactly one head and two tails when tossing three coins, we first count the favorable outcomes (one head and two tails), which are (angka, gambar, gambar), (gambar, angka, gambar), and (gambar, gambar, angka). There are 3 favorable outcomes out of 8 total outcomes. Hence, the probability is 3/8.

What is the probability of rolling a prime number on a die and getting heads on a coin?

The prime numbers on a die are 2, 3, and 5. The favorable outcomes for rolling a prime number and getting heads on a coin are: (2, gambar), (3, gambar), and (5, gambar). There are 3 favorable outcomes out of 12 total outcomes (6 outcomes for the die and 2 for the coin). Hence, the probability is 3/12 or 1/4.

What does it mean when the probability of an event is 0?

When the probability of an event is 0, it means the event is impossible and will never happen. For example, rolling a 7 on a standard die has a probability of 0, as 7 is not a possible outcome.

What does it mean when the probability of an event is 1?

When the probability of an event is 1, it means the event is certain to happen. For example, if you roll a die, the probability of getting a number between 1 and 6 (inclusive) is 1, as it is guaranteed.

How is probability calculated using the formula P(A) = n(A) / n(S)?

Probability is calculated by dividing the number of favorable outcomes (n(A)) by the total number of possible outcomes (n(S)). For instance, when rolling a die and finding the probability of getting an odd number, the number of favorable outcomes is 3 (1, 3, 5), and the total number of outcomes is 6. So, the probability is 3/6 or 1/2.