(LENGKAP) Kumpulan soal-soal ujian turunan fungsi trigonometri
Summary
TLDRIn this educational video, the presenter walks viewers through a variety of algebraic function derivative problems, specifically focusing on trigonometric and algebraic functions. Each problem is broken down step by step, with clear explanations of key concepts, such as how to differentiate functions like sine, cosine, and combinations of algebraic expressions. The video also covers the application of rules such as the product rule, quotient rule, and chain rule. Throughout the video, the presenter encourages viewers to follow along and reach out with questions, aiming to help them understand and master derivative calculations with ease.
Takeaways
- 😀 The video focuses on explaining how to find the derivatives of algebraic functions, particularly trigonometric ones.
- 😀 The first example involves differentiating the function f(x) = 2 Sin x, where the derivative is 2 Cos x.
- 😀 The second example shows how to differentiate f(x) = Sin x - Cos x, resulting in f'(x) = Cos x + Sin x.
- 😀 In the third example, f(x) = Sin(3x - 4) is differentiated, showing that the derivative is 3 Cos(3x - 4).
- 😀 For the fourth example, f(x) = 3x Sin(5 - 4x) is treated using the product rule, resulting in a more complex derivative expression.
- 😀 The fifth example involves differentiating a function in fractional form, f(x) = Sin x / (Sin x + Cos x), using the quotient rule.
- 😀 The sixth example illustrates how to differentiate f(x) = (3x² + Sin⁴ x), where both polynomial and trigonometric terms are involved.
- 😀 The seventh example demonstrates differentiating a squared trigonometric function, f(x) = (Sin x + Cos x)², using the chain rule.
- 😀 In the eighth example, the differentiation of f(x) = Sin³(2x - 4) is done, applying the chain rule and simplifying the expression.
- 😀 The ninth example shows how to handle a cubic root function, f(x) = ³√(Sin²(3x)), converting the radical into a fractional exponent for easier differentiation.
Q & A
What is the main topic covered in the video?
-The video primarily focuses on discussing derivative problems related to algebraic functions, especially trigonometric functions such as sine and cosine, with step-by-step solutions.
What is the derivative of the function f(x) = 2sin(x)?
-The derivative of f(x) = 2sin(x) is f'(x) = 2cos(x), since the derivative of sin(x) is cos(x), and the constant 2 remains unchanged.
How is the derivative of f(x) = sin(x) - cos(x) calculated?
-The derivative of f(x) = sin(x) - cos(x) is f'(x) = cos(x) + sin(x), where the derivative of sin(x) is cos(x) and the derivative of -cos(x) is sin(x).
What is the process for finding the derivative of a composite function like f(x) = sin(3x - 4)?
-For the function f(x) = sin(3x - 4), first take the derivative of the inner function (3x - 4), which is 3, and then multiply by the derivative of sin(x), which is cos(x). The final result is f'(x) = 3cos(3x - 4).
How do you find the derivative of a product of functions, such as f(x) = 3x * sin(5 - 4x)?
-To find the derivative of f(x) = 3x * sin(5 - 4x), apply the product rule: f'(x) = u'v + uv', where u = 3x and v = sin(5 - 4x). The derivative is f'(x) = 3sin(5 - 4x) - 12xcos(5 - 4x).
What is the derivative of a function involving a quotient, such as f(x) = sin(x) / (sin(x) + cos(x))?
-For the function f(x) = sin(x) / (sin(x) + cos(x)), use the quotient rule: f'(x) = (u'v - uv') / v^2, where u = sin(x) and v = sin(x) + cos(x). The derivative simplifies to f'(x) = (cos(x) * (sin(x) + cos(x)) - sin(x) * (cos(x) - sin(x))) / (sin(x) + cos(x))^2.
How do you find the derivative of f(x) = sin^4(x)?
-To find the derivative of f(x) = sin^4(x), use the chain rule. The derivative is f'(x) = 4sin^3(x) * cos(x), as the derivative of sin(x) is cos(x), and the power rule applies to the exponent 4.
What method is used to differentiate a function like f(x) = (sin^3(2x - 4))?
-To differentiate f(x) = sin^3(2x - 4), use the chain rule. First, differentiate the outer function (the cube) and then multiply by the derivative of the inner function (2x - 4). The final derivative is f'(x) = 6cos(2x - 4)sin^2(2x - 4).
What is the process for differentiating a function involving an identity like f(x) = sin^2(x) + cos^2(x)?
-The function f(x) = sin^2(x) + cos^2(x) is an identity that simplifies to 1 due to the Pythagorean trigonometric identity. Therefore, the derivative of f(x) is f'(x) = 0.
How is the derivative of f(x) = sqrt[3]{sin^2(3x)} found?
-To find the derivative of f(x) = sqrt[3]{sin^2(3x)}, rewrite it as f(x) = sin^(2/3)(3x). Apply the chain rule, then simplify the expression, resulting in f'(x) = 2cos(3x) * sin^(-1/3)(3x) * 3.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes
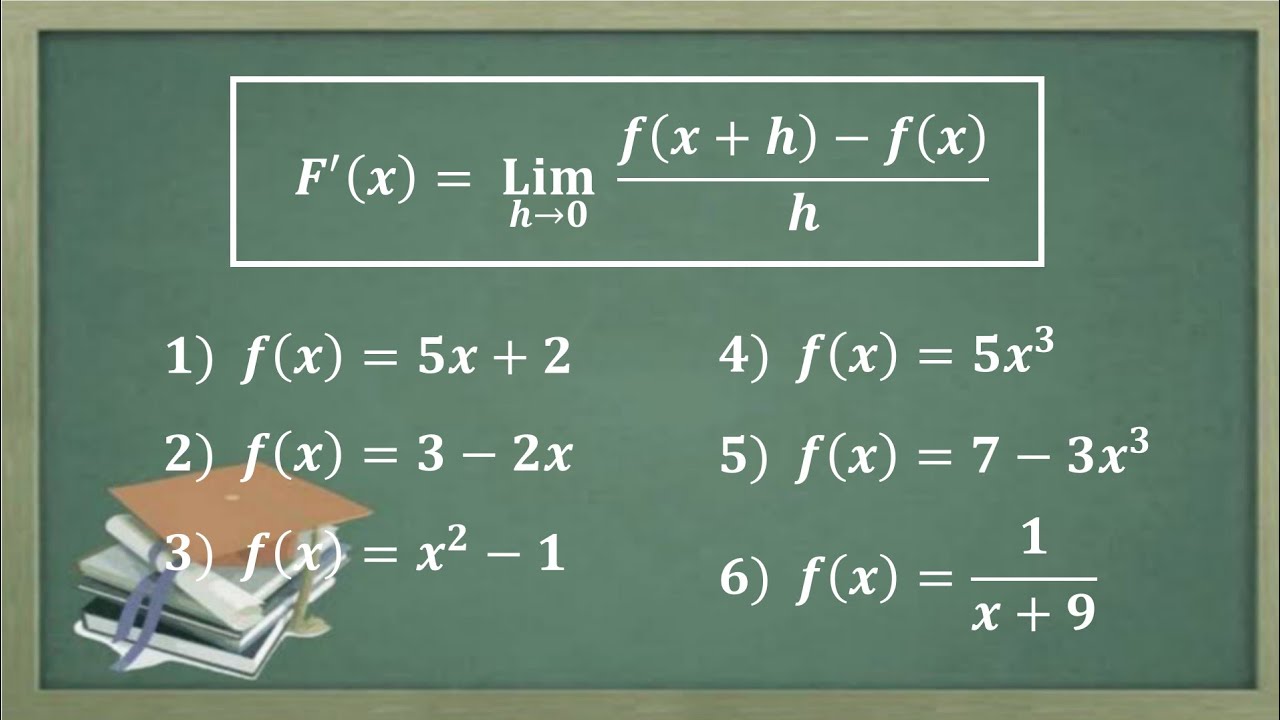
Turunan dengan menggunakan defnisi turunan Turunan menggunakan limit

BUKU MATEMATIKA PKS GEMATAMA KELAS XI FASE F KURIKULUM MERDEKA MATERI OPERASI ALJABAR PADA FUNGSI
Konsep Dasar Turunan Fungsi Aljabar Matematika Wajib Kelas 11 m4thlab

Identidades trigonométricas resoluções de exercícios

Simplifying Trigonometric Expressions

Operasi aljabar pada fungsi
5.0 / 5 (0 votes)
