PENYELESAIAN PDP DENGAN PEMISAHAN VARIABEL CONTOH SOAL 1A
Summary
TLDRThis video explains how to solve a differential equation for the deflection of a taut rope under tension, with specific boundary and initial conditions. The rope, initially displaced 5 cm upwards at the center, is modeled using separation of variables. The process involves determining the displacement equation based on linear boundary conditions, performing integrations, and arriving at a final result expressed as a series. The explanation combines theoretical steps with detailed mathematical formulations to derive the rope's deflection behavior over time, providing insight into solving similar physical problems.
Takeaways
- 😀 The lecture focuses on solving Partial Differential Equations (PDP) using the method of separation of variables.
- 😀 The problem involves a taut string with fixed ends, initially displaced vertically by 5 cm and then released.
- 😀 The string is 80 cm long and is stretched tightly, with no horizontal or vertical movement at the ends.
- 😀 The goal is to determine the equation of motion or deflection (UX) for the string under these conditions.
- 😀 The general wave equation is presented and used to solve for the string’s deflection.
- 😀 Boundary conditions are established, where the displacement at both ends (X = 0 and X = 80) is zero.
- 😀 Initial conditions are also given, stating that the initial velocity of the string is zero.
- 😀 The initial shape of the string is assumed to be linear, leading to a linear function for deflection (y = AX + B).
- 😀 Mathematical techniques such as integration are applied to determine the coefficients and solve for the deflection equation.
- 😀 The solution to the PDE results in a series expression for UX(t), involving summation over modes and coefficients.
- 😀 The lecture ends by emphasizing the application of these methods to obtain the desired solution for the string's deflection.
- 😀 The lecturer encourages students to practice calculating the integrals and applying the derived formulas themselves.
Q & A
What is the main topic discussed in this lecture?
-The main topic of the lecture is solving partial differential equations (PDP) using the method of separation of variables, with a specific focus on the motion of a stretched string.
What is the setup of the problem involving the string?
-The problem involves a string that is initially stretched to a length of 80 cm. Both ends of the string are fixed, and the string is then pulled upwards by 5 cm in the middle and released with zero velocity.
Why is it important to determine the boundary conditions for this problem?
-Boundary conditions are important because they define the fixed positions of the string at both ends, where there is no movement. This helps in formulating the solution to the partial differential equation correctly.
What boundary conditions are used in this problem?
-The boundary conditions are that at both ends of the string (x=0 and x=80 cm), the displacement of the string is zero, meaning there is no movement at those points.
How is the initial deflection of the string modeled?
-The initial deflection is modeled as a linear function, given by the equation y = AX + B, where A and B are constants determined based on the geometry of the setup.
What is the significance of the zero initial velocity in this problem?
-The zero initial velocity means that the string is released from rest, which simplifies the solution since the initial velocity terms vanish in the equations for motion.
How are the coefficients of the solution determined?
-The coefficients are determined by solving integrals that result from the boundary and initial conditions. These integrals help calculate the necessary parameters for the series solution.
What role does the series solution play in solving the problem?
-The series solution represents the displacement of the string as a sum of sinusoidal terms. This series is derived from the solution to the partial differential equation and reflects the oscillatory motion of the string.
What is the final form of the solution for the displacement of the string?
-The final solution for the displacement of the string is expressed as a series: u(x,t) = (40 / v^2) * Σ (n=1 to ∞) [n^2 * b^2 * sin(nπx / 80)] * cos(nπvt / 80).
How does the method of separation of variables help in solving the partial differential equation?
-The method of separation of variables allows the partial differential equation to be split into two simpler ordinary differential equations—one for time and one for space—making the solution process more manageable.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

The Key Definitions of Differential Equations: ODE, order, solution, initial condition, IVP
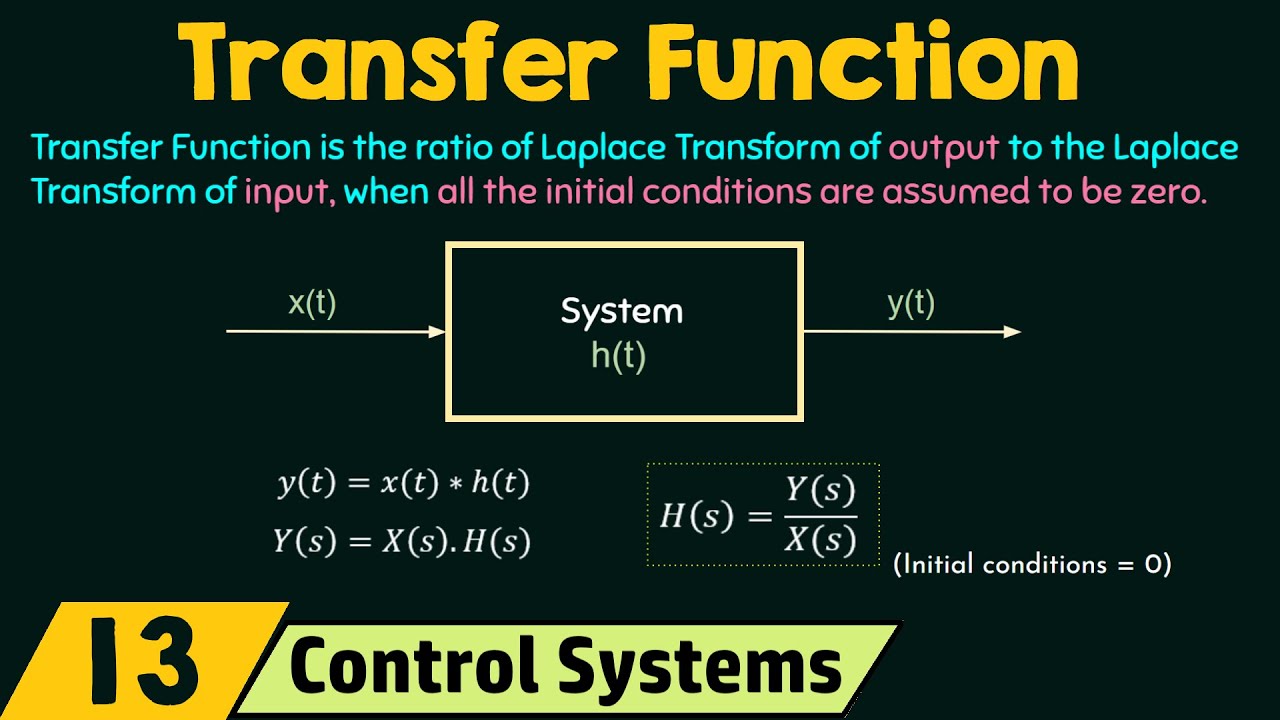
Introduction to Transfer Function

Logistic Differential Equation (general solution)

Oxford Calculus: Separable Solutions to PDEs

LECTURE NOTES: AIRCRAFT AERODYNAMICS I, CHAPTER I, PART 3

Taylor Series Method To Solve First Order Differential Equations (Numerical Solution)
5.0 / 5 (0 votes)
