Matematika SMA - Barisan dan Deret (6) - Barisan Geometri, Rumus Un Barisan Geometri (A)

Summary

This video provides a comprehensive guide to understanding geometric sequences (barisan geometri). It covers essential concepts such as the common ratio, the formula for finding the nth term, and how to solve real-world problems involving geometric sequences. The tutorial includes examples to calculate terms, find ratios, and solve problems involving modified sequences with inserted numbers. By the end, viewers will have a solid understanding of geometric sequences, how to apply formulas, and how to handle variations such as inserted numbers. The video is designed for learners seeking a detailed explanation of these mathematical concepts.

Takeaways

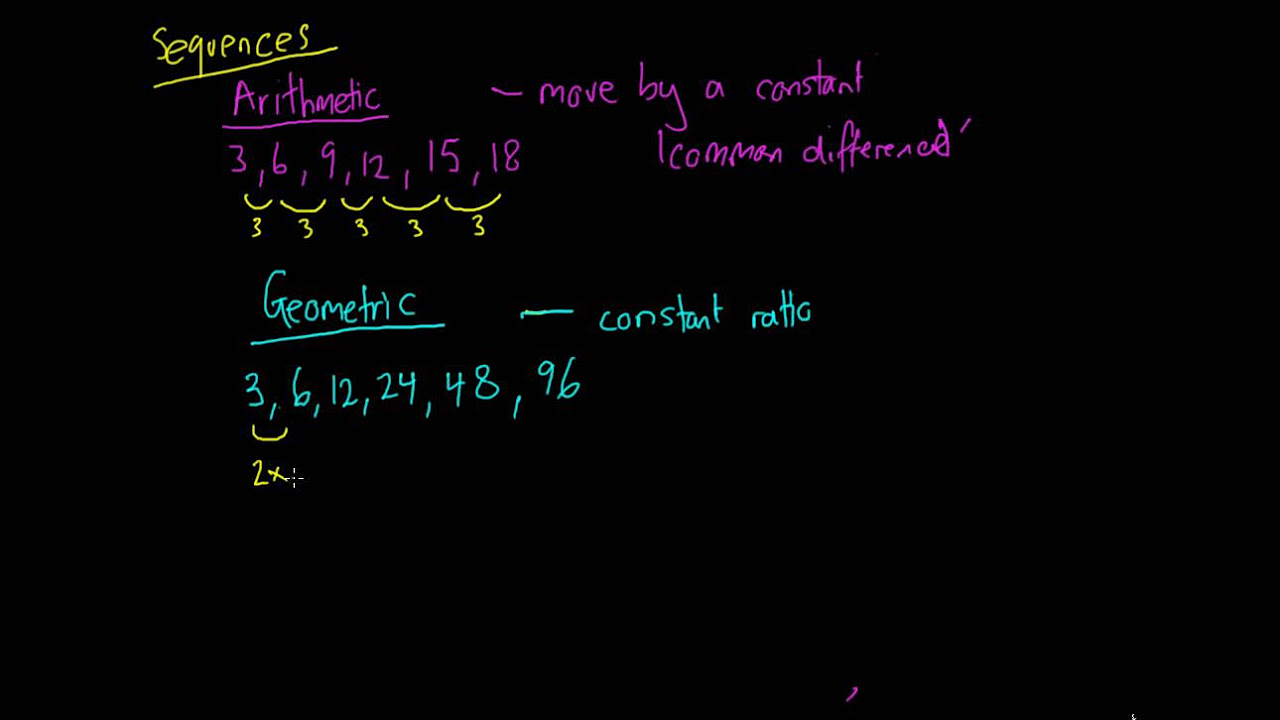
• 😀 Geometric sequences have a constant ratio between consecutive terms, known as the common ratio.
• 😀 To find the common ratio of a geometric sequence, divide any term by its previous term.
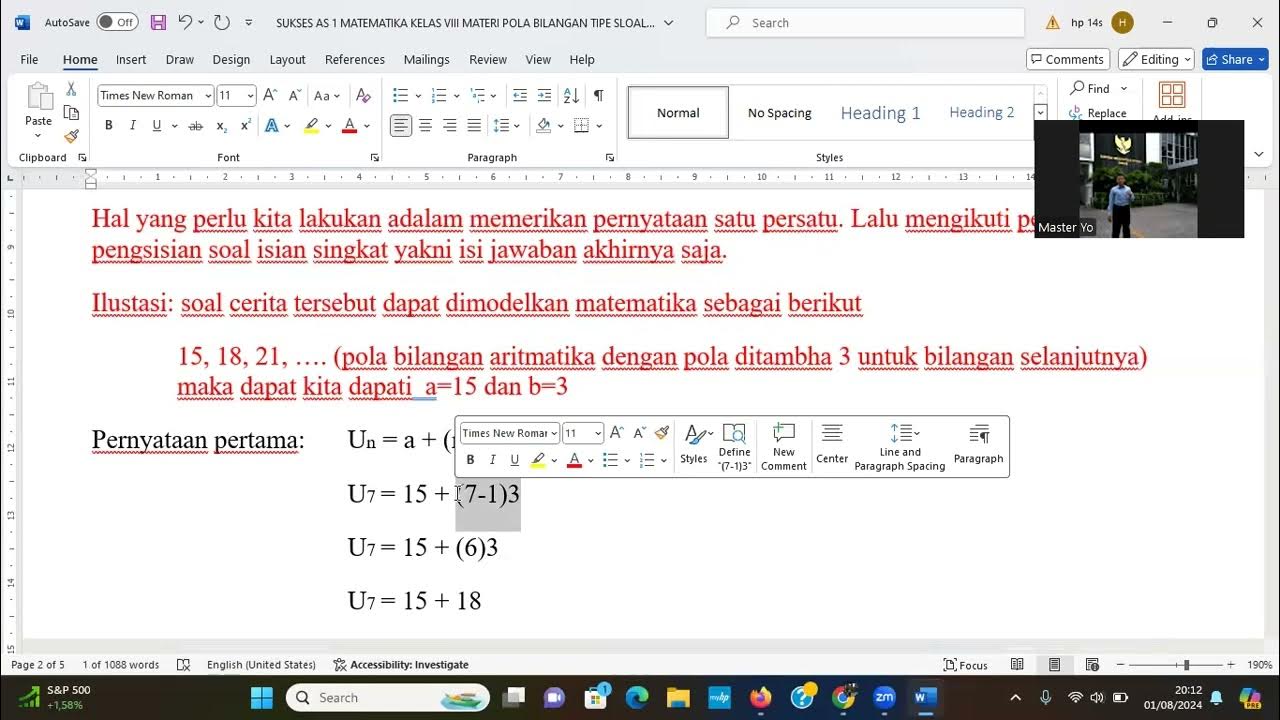
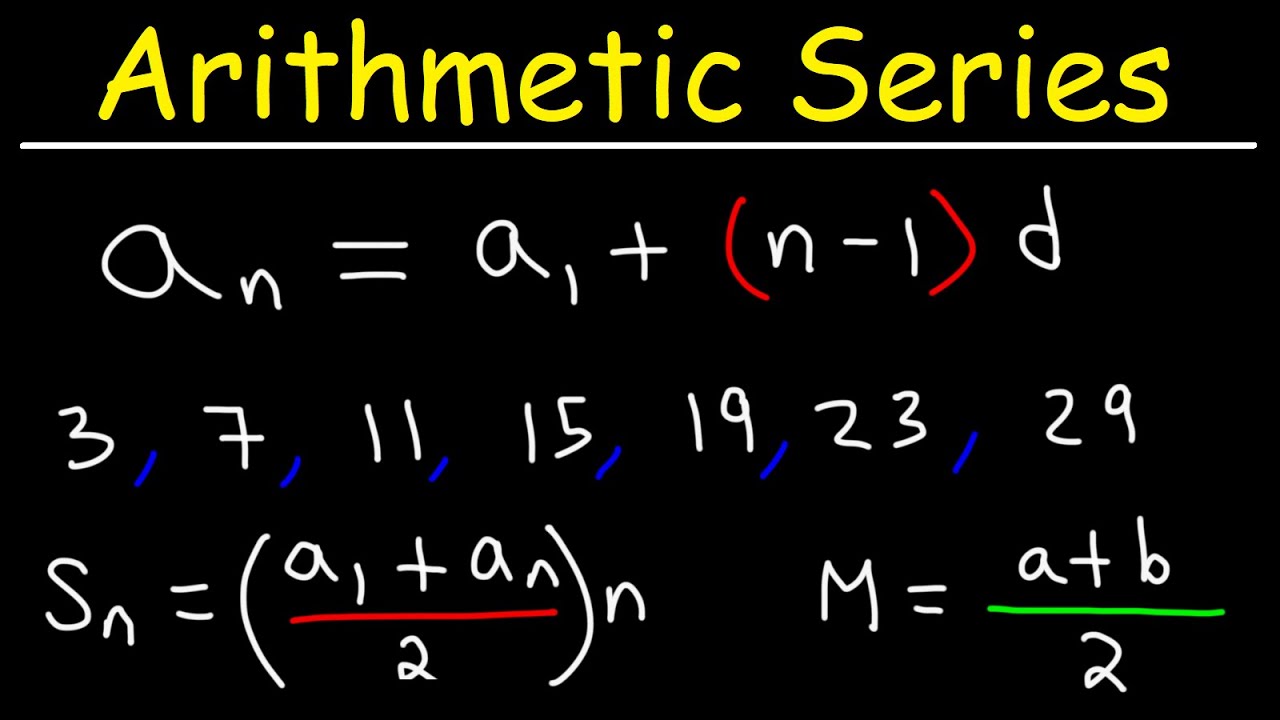
• 😀 The formula for finding the nth term of a geometric sequence is: u_n = a * r^(n-1), where 'a' is the first term and 'r' is the common ratio.
• 😀 Inserting terms into a geometric sequence creates a new ratio, which can be calculated using the formula: r' = r^(1/(k+1)), where 'r' is the original ratio and 'k' is the number of inserted terms.
• 😀 To find the 10th term in a geometric sequence, use the formula with the first term and common ratio, raising the ratio to the power of n-1.
• 😀 The common ratio of a geometric sequence can be negative, as shown in exercises where the ratio was found to be -3.
• 😀 The value of the 10th term in a geometric sequence can grow very large, especially with a high common ratio, as shown by the example of u_10 = 39366.
• 😀 For sequences with fractional terms, such as 1/32, 1/16, 1/8, the common ratio can be calculated just like with integer sequences.
• 😀 Geometric sequences can be manipulated by adjusting the number of terms between existing terms, which changes the sequence's common ratio and resulting values.
• 😀 The step-by-step process of solving for the common ratio and nth terms is demonstrated through multiple exercises, reinforcing the key concepts of geometric sequences.

Q & A

What is a geometric sequence?

A geometric sequence is a sequence of numbers where each term is obtained by multiplying the previous term by a constant called the common ratio (r).

How is the common ratio (r) of a geometric sequence calculated?

The common ratio (r) is calculated by dividing any term in the sequence by the previous term. For example, r = second term / first term, or r = third term / second term.

What is the formula for finding the n-th term (U_n) of a geometric sequence?

The formula for the n-th term of a geometric sequence is U_n = a * r^(n-1), where 'a' is the first term, 'r' is the common ratio, and 'n' is the term number.

How do you find the 10th term of a geometric sequence?

To find the 10th term, use the formula U_10 = a * r^(10-1). Substitute the values for 'a' and 'r', and calculate the result.

In the sequence 2, 6, 18, 54,... what is the common ratio?

The common ratio (r) is 3 because 6/2 = 18/6 = 54/18 = 3.

What is the 10th term of the sequence 2, 6, 18, 54,...?

To find the 10th term, use the formula U_10 = 2 * 3^(10-1). This gives U_10 = 2 * 3^9 = 2 * 19683 = 39366.

What happens when numbers are inserted into a geometric sequence?

When numbers are inserted into a geometric sequence, a new ratio is formed. The new common ratio (r') is calculated using the formula r' = r^(1/(k+1)), where 'k' is the number of inserted terms and 'r' is the original common ratio.

How do you find the new common ratio after inserting numbers into a geometric sequence?

The new common ratio r' is found by using the formula r' = r^(1/(k+1)), where 'r' is the original common ratio and 'k' is the number of terms inserted.

What is the formula for the n-th term of a geometric sequence with inserted numbers?

The formula for the n-th term in a sequence with inserted numbers is still U_n = a * r'^(n-1), where 'a' is the first term, 'r' is the new common ratio, and 'n' is the term number.

How do you find the common ratio from given terms such as U5 = 162 and U2 = 6?

To find the common ratio, use the formula U_n = a * r^(n-1). For U5 = 162 and U2 = 6, you can set up two equations and solve for 'r'. In this case, r = -3.