Factoring Perfect Square Trinomials

Summary

This educational video teaches how to factor perfect square trinomials. It explains the process with examples, starting with x^2 + 6x + 9, which factors into (x + 3)^2. The video then discusses identifying perfect square trinomials by checking if the square root of the leading coefficient times the square root of the constant term equals half the middle term. It further demonstrates factoring with examples like x^2 + 10x + 25 and 4x^2 + 12x + 9, explaining the use of the formula a^2 + 2ab + b^2 = (a + b)^2. The video concludes with a complex example involving variables, showing step-by-step factoring.

Takeaways

• 🔢 The video focuses on factoring perfect square trinomials, breaking down the process step-by-step.
• ❓ The method involves finding two numbers that multiply to the constant term and add to the middle coefficient.
• ✖️ Example 1: For x² + 6x + 9, the numbers 3 and 3 work, so it's factored as (x + 3)².
• 🧪 FOIL method can be used to verify that the factorization is correct.
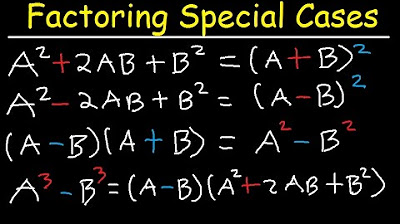
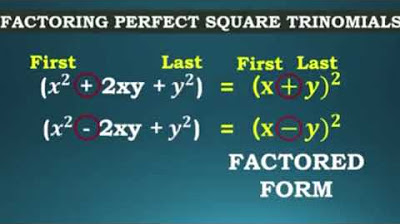
• 📝 A perfect square trinomial has the form a² + 2ab + b², which can be factored into (a + b)².
• 🔍 Example 2: x² + 10x + 25 is also a perfect square trinomial, factored as (x + 5)².
• ✅ To check if a trinomial is a perfect square, the product of the square roots of the first and last terms should be half of the middle term.
• 💡 Example 3: 4x² + 12x + 9 is factored as (2x + 3)² using a similar method.
• 📐 When the leading coefficient is not 1, additional steps like factoring by grouping may be required.
• 🔗 In more complex examples, such as 9a² + 30ab + 25b², the same principles apply, and it can be factored as (3a + 5b)².

Q & A

What is a perfect square trinomial?

A perfect square trinomial is a quadratic expression that can be factored into the square of a binomial. For example, \( x^2 + 6x + 9 \) can be factored as \( (x + 3)^2 \).

How do you factor a perfect square trinomial?

To factor a perfect square trinomial, you find two numbers that multiply to the constant term and add to the middle coefficient. Then, you write the expression as the square of a binomial. For example, \( x^2 + 6x + 9 \) factors as \( (x + 3)^2 \).

How can you verify that your factored expression is correct?

You can verify the factored form by expanding the binomial back to its original form. For example, \( (x + 3)(x + 3) \) expands to \( x^2 + 6x + 9 \), which matches the original expression.

What formula can help identify perfect square trinomials?

The formula \( a^2 + 2ab + b^2 = (a + b)^2 \) can help identify and factor perfect square trinomials. By comparing the terms to this structure, you can determine whether the trinomial is a perfect square.

What is an example of factoring a perfect square trinomial where the leading coefficient is not 1?

For the expression \( 4x^2 + 12x + 9 \), the square root of 4 is 2 and the square root of 9 is 3. Since \( 2 \times 3 = 6 \) and 6 is half of 12, this is a perfect square trinomial, factored as \( (2x + 3)^2 \).

How can you factor a perfect square trinomial using grouping?

In some cases, such as with \( 4x^2 + 12x + 9 \), you can factor by grouping. After breaking the middle term into two parts, you factor out the greatest common factor (GCF) from each group and then factor the entire expression.

Why is the middle term important in identifying a perfect square trinomial?

The middle term helps confirm whether the expression is a perfect square trinomial because it should be equal to twice the product of the square roots of the first and last terms. If this condition is met, the expression is a perfect square.

How do you factor a trinomial with both variables, like \( 9a^2 + 30ab + 25b^2 \)?

First, find the square roots of the first and last terms, which are 3a and 5b respectively. Then check that twice their product (15ab) is half of the middle term (30ab). Since it is, the expression factors as \( (3a + 5b)^2 \).

What steps are involved in factoring a more complex perfect square trinomial like \( 9a^2 + 30ab + 25b^2 \)?

First, calculate the square roots of 9a² and 25b² (which are 3a and 5b). Then, confirm that twice their product equals the middle term. Once confirmed, factor the expression as \( (3a + 5b)^2 \).

Can the method for factoring perfect square trinomials be applied to any quadratic expression?

No, this method only works if the expression fits the pattern of a perfect square trinomial. If the conditions don't match (i.e., if the middle term isn't twice the product of the square roots of the first and last terms), other factoring methods must be used.