Angle Measure (Precalculus)
Summary
TLDRIn this lesson on trigonometry, learners transition from understanding trigonometry in triangles to its application in circles. The video covers key concepts such as defining angles in trigonometry, converting between degrees and radians, and introduces the unit circle. The lesson emphasizes the periodic nature of trigonometric functions, central angles, and the significance of angles in standard position. With examples, it demonstrates how to convert angle measurements between degrees and radians. This foundation is essential for applications in science and engineering, with references to historical figures like Hipparchus.
Takeaways
- 📐 Trigonometry extends beyond right triangles to include circles, with applications in science and engineering.
- 🕰️ Circular functions like sine, cosine, and tangent are periodic and relate to natural phenomena, such as the movement of a clock's hands.
- 👨🏫 Hipparchus, a Greek astronomer, is considered the founder of trigonometry due to his study of angles in spheres, including the Earth's radius.
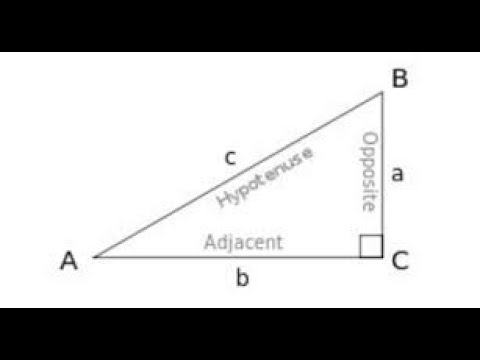
- 🔺 Trigonometry isn't limited to right triangles; it also includes oblique triangles and triangles inscribed in circles.
- 🔄 An angle in trigonometry is defined by the rotation of a ray about its endpoint, with positive angles rotating counterclockwise and negative angles rotating clockwise.
- 📊 Angles in standard position must be drawn on the Cartesian plane, with the vertex at the origin and the initial side along the positive x-axis.
- ⭕ A unit circle has its center at the origin, with a radius of one unit. The standard equation is x² + y² = 1.
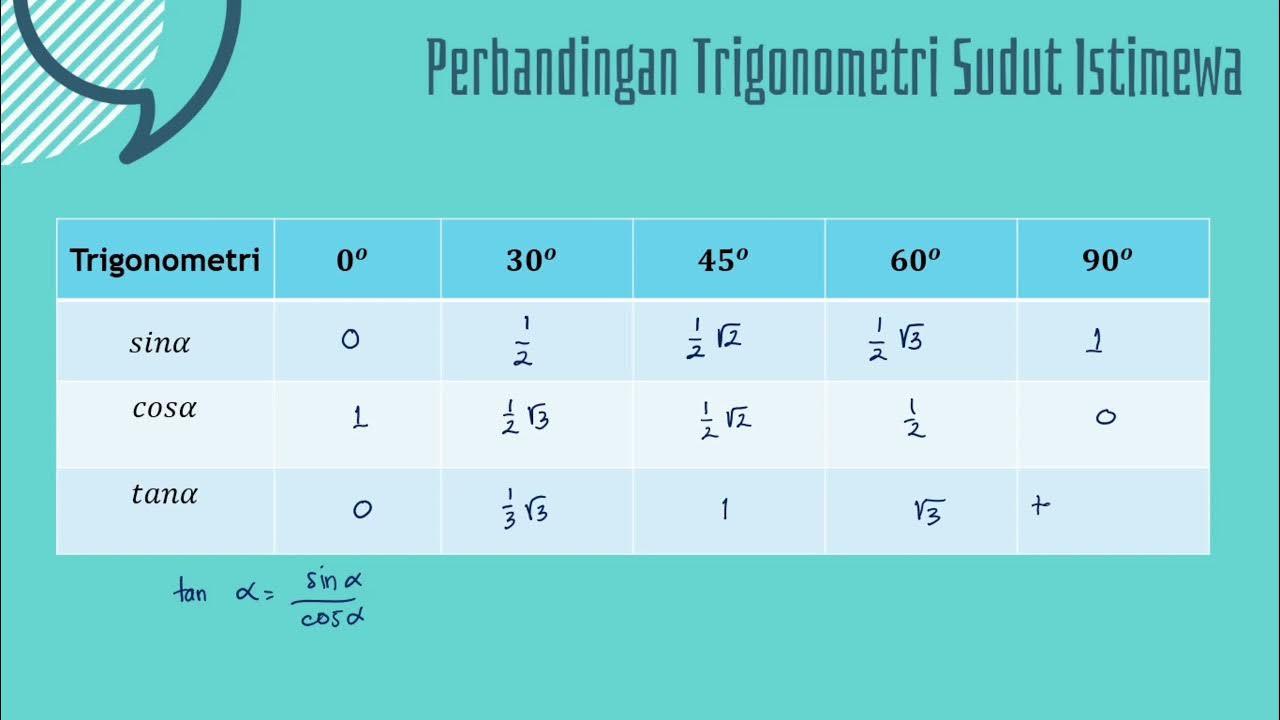
- 📏 Degrees and radians are two units of angle measurement, with 360 degrees equal to 2π radians.
- 🧮 To convert degrees to radians, multiply by π/180; to convert radians to degrees, multiply by 180/π.
- ⏳ Degree measures can also be broken down into minutes and seconds, where 1 degree = 60 minutes and 1 minute = 60 seconds.
Q & A
What is the focus of the lesson in quarter two, week one?
-The focus of the lesson is on angle measures in a unit circle, including defining angles in trigonometry and converting between degree and radian measurements.
How does trigonometry transition from triangles to circles?
-Trigonometry transitions from triangles to circles by moving from right triangle trigonometry, studied in Grade 9, to circular functions, where trigonometric functions are placed in a unit circle, showing their periodic nature.
What are circular functions, and how are they related to trigonometric functions?
-Circular functions are the trigonometric functions, including sine, cosine, tangent, and their reciprocals (secant, cosecant, cotangent), but placed within a circle to reflect their periodic nature.
Who is considered the founder of trigonometry, and why?
-Hipparchus, a Greek astronomer and mathematician, is considered the founder of trigonometry because he studied the Earth as a sphere and defined angles, contributing to the foundation of the subject.
What is the etymology of the word 'trigonometry'?
-The word 'trigonometry' comes from the Greek words 'trigonon,' meaning triangle, and 'metron,' meaning measure. It originally referred to the study of the relationships between the sides and angles of triangles.
What is an angle in trigonometry, and how is it different from a geometric angle?
-An angle in trigonometry is formed by rotating a ray about its endpoint. Unlike a geometric angle, which is static, trigonometric angles can have both positive and negative measurements depending on the direction of rotation.
What defines an angle in standard position in trigonometry?
-An angle is in standard position if it is drawn on the Cartesian plane with its vertex at the origin, and its initial side lies along the positive x-axis.
What is a unit circle, and why is it important in trigonometry?
-A unit circle is a circle centered at the origin with a radius of one unit. It is crucial in trigonometry because it helps define circular functions and the relationship between angles and their corresponding coordinates on the circle.
How is a central angle in a unit circle related to radian and degree measures?
-A central angle in a unit circle can be measured in either degrees or radians. One full revolution (360°) is equivalent to 2π radians. The radian measure is often used in higher-level mathematics.
How can you convert between degree and radian measures?
-To convert degrees to radians, multiply the degree measure by π/180. To convert radians to degrees, multiply the radian measure by 180/π.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenant5.0 / 5 (0 votes)