Geometric Means Between Two Terms | How to Insert? | Grade 10 MELC

Math Corner

27 Sept 202005:02

Summary

TLDRIn this 'Math Corner' video, the host teaches how to insert geometric means between two numbers, specifically between 2/3 and 1/12. The process involves setting up a geometric sequence with a common ratio 'r'. By using the formula for the nth term of a geometric sequence, the host calculates 'r' to be 1/2. Subsequently, the second and third terms of the sequence are determined as 1/3 and 1/6, respectively, showcasing the method to find geometric means between given numbers.

Takeaways

📚 The video is focused on teaching how to insert geometric means between two numbers.
🔢 The terms chosen for the example are 'two over three' and 'one over twelve'.
📈 Two geometric means are to be inserted, making the total number of terms, including the first and last, four.
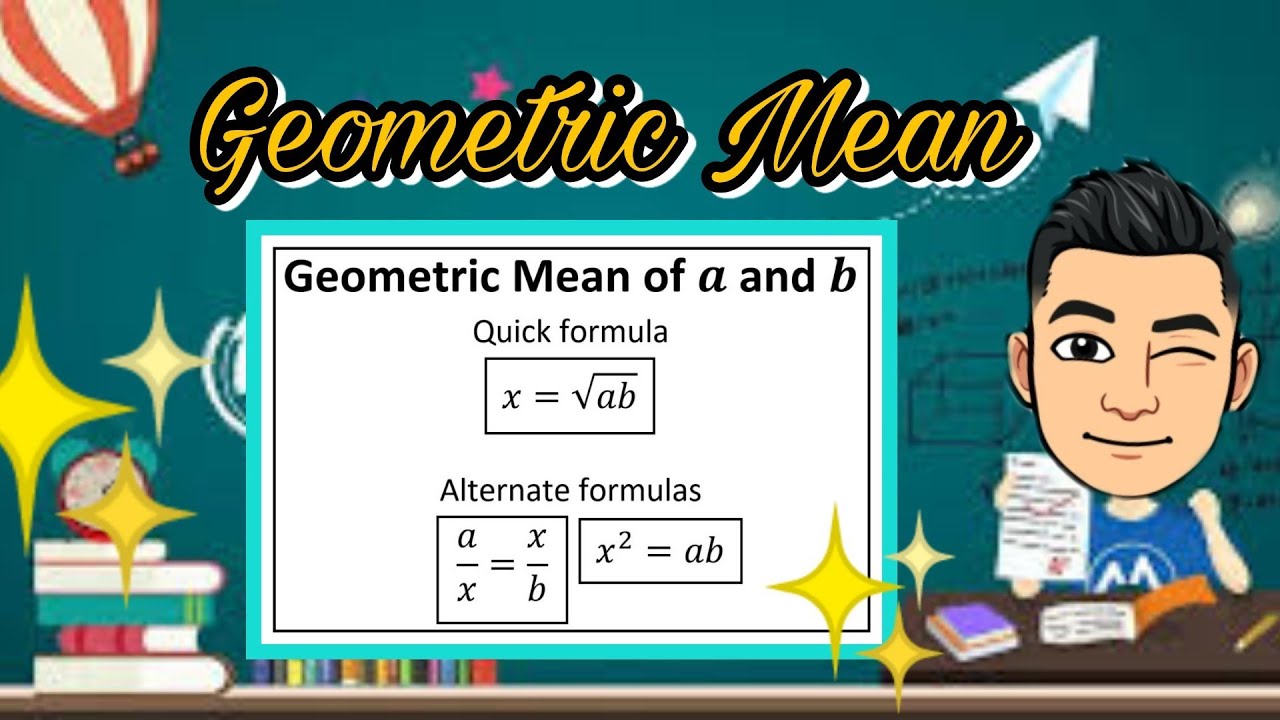
✅ The formula used to find the nth term of a geometric sequence is applied: a_n = a_1 × r^(n-1).
🔄 The common ratio (r) is solved using the given terms and the formula, resulting in r = √[3]{1/8}.
📉 The calculation simplifies to find r = 1/2 after taking the cube root.
📌 The second term (a_2) is found by multiplying the first term (a_1) by the common ratio (r), resulting in 'one over six'.
📐 The third term (a_3) is calculated by multiplying the second term by the common ratio, yielding 'one over six'.
🔑 The two geometric means between 'two over three' and 'one over twelve' are 'one over three' and 'one over six'.
🎓 The video concludes by summarizing the process of inserting geometric means between two given terms.

Q & A

What is the main topic of the video?
-The main topic of the video is how to insert geometric means between two given terms.
What are the two terms between which geometric means are to be inserted?
-The two terms are two over three and one over twelve.
How many geometric means are being inserted in the sequence?
-Two geometric means are being inserted between the given terms.
What is the formula used to find the nth term of a geometric sequence?
-The formula used to find the nth term of a geometric sequence is \( a_n = a_1 \times r^{(n-1)} \).
What is the value of 'n' in the context of the problem?
-In the context of the problem, 'n' is equal to four, as one over twelve is the fourth term.
How is the common ratio 'r' calculated in the video?
-The common ratio 'r' is calculated by isolating 'r' in the equation \( \frac{1}{12} = \frac{2}{3} \times r^3 \) and then taking the cube root of both sides.
What is the value of the common ratio 'r' after solving?
-The value of the common ratio 'r' is one over two.
How is the second term of the sequence determined?
-The second term is determined by multiplying the first term (two over three) by the common ratio (one over two).
What is the value of the second term after calculation?
-The value of the second term is one over six.
How is the third term of the sequence calculated?
-The third term is calculated by multiplying the second term (one over six) by the common ratio (one over two).
What are the two geometric means inserted between the given terms?
-The two geometric means inserted between two over three and one over twelve are one over three and one over six.