FACTORING GENERAL TRINOMIALS || GRADE 8 MATHEMATICS Q1
Summary
TLDRThis educational video script focuses on factoring quadratic trinomials of the form x^2 + bx + c and solving related polynomial problems. It guides viewers through listing integer factor pairs for given numbers, identifying pairs that match the middle term 'b' and product 'c', and then forming the factored expression (x + m)(x + n). Examples are provided to demonstrate the process, including cases where trinomials cannot be factored with integer coefficients, highlighting the concept of prime trinomials. The script concludes with a practical application, using factoring to determine the dimensions of a box from a given volume expression, emphasizing the importance of understanding both positive and negative integer pairs in factoring.
Takeaways
- 📘 The video focuses on factoring quadratic trinomials of the form \(x^2 + bx + c\) and solving problems involving factors of polynomials.
- 🔢 It begins by listing all pairs of integers or factors for given numbers, which is a preparatory step for factoring trinomials.
- 📐 The process involves identifying pairs of integers whose product equals \(c\) (the constant term) and whose sum equals \(b\) (the coefficient of the middle term).
- 🔑 The factored form of a quadratic trinomial \(x^2 + bx + c\) is presented as \((x + m)(x + n)\), where \(m\) and \(n\) are the chosen integers.
- 🌰 Several examples are provided to demonstrate the factoring process, including \(x^2 + 10x + 16\), \(x^2 - 9x + 18\), and \(x^2 - 2x - 24\).
- ❌ The video clarifies that not all quadratic trinomials can be factored with integer coefficients, such as \(x^2 + 3x + 3\), which is a prime trinomial.
- 📦 An application of factoring is shown by solving for the dimensions of a box given its volume represented by a cubic polynomial expression.
- ✂️ The video demonstrates that if the polynomial is not a quadratic trinomial, such as \(4x^3 + 16x^2 - 48x\), it should first be simplified by factoring out the greatest common factor.
- 📉 The importance of considering both positive and negative integer pairs in the factoring process is emphasized, with examples illustrating how to choose the correct pair based on the sum and product requirements.
- 🎓 The video concludes with a summary of the key points and a call to action for viewers to engage with the content by liking, subscribing, and watching more.
Q & A
What is the main topic of the video?
-The main topic of the video is factoring quadratic trinomials of the form x^2 + bx + c and solving problems involving factors of polynomials.
How does the video begin?
-The video begins by listing all pairs of integers or factors for various numbers such as 4, 6, 8, 10, 12, 18, 20, and 30.
What is the general form of a quadratic trinomial?
-The general form of a quadratic trinomial is x^2 + bx + c.
What is the factored form of a quadratic trinomial?
-The factored form of a quadratic trinomial is (x + m)(x + n), where b is the sum of m and n, and c is their product.
How does the video demonstrate the process of factoring a quadratic trinomial?
-The video demonstrates the process by first listing pairs of integers whose product is equal to 'c' and then choosing a pair whose sum is equal to 'b' to form the factors (x + m) and (x + n).
What is the first example given in the video for factoring a quadratic trinomial?
-The first example given is x^2 + 10x + 16, where the factors are identified as (x + 2) and (x + 8).
What is the significance of choosing a pair of integers whose sum is equal to 'b'?
-Choosing a pair of integers whose sum is equal to 'b' ensures that when the factors (x + m) and (x + n) are expanded, the middle term of the trinomial matches the original expression.
What does the video say about quadratic trinomials that cannot be factored using integer coefficients?
-The video states that if a quadratic trinomial cannot be factored using integer coefficients, it is an example of a prime trinomial.
How does the video handle a polynomial with a degree higher than two?
-The video first factors out the greatest common factor (GCF) for polynomials with a degree higher than two, reducing the problem to factoring a quadratic trinomial.
What is the application of factoring quadratic trinomials shown in the video?
-The video shows an application of factoring quadratic trinomials by solving a problem to find the dimensions of a box given its volume represented by a cubic polynomial.
What is the final advice given by the video to viewers?
-The video advises viewers to ensure that the integers chosen for factoring are either both positive or both negative, depending on the sign of the middle term 'b'.
Outlines

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantMindmap

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantKeywords

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantHighlights

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantTranscripts

Cette section est réservée aux utilisateurs payants. Améliorez votre compte pour accéder à cette section.
Améliorer maintenantVoir Plus de Vidéos Connexes

Pemfaktoran Suku Bentuk Aljabar - bagian 2 💡Pasti Bisa

Persamaan Kuadrat Kelas 10 Kurikulum Merdeka
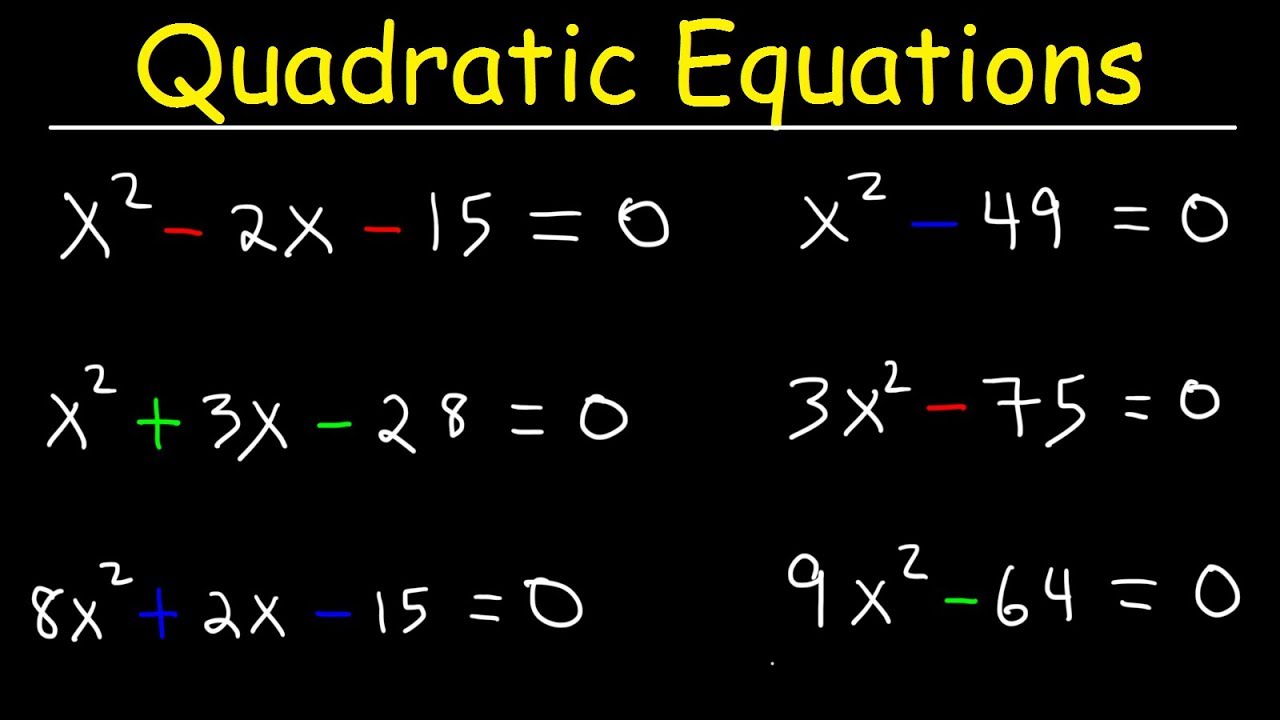
How To Solve Quadratic Equations By Factoring - Quick & Simple! | Algebra Online Course

Persamaan Kuadrat part 1

TRANSFORMING QUADRATIC FUNCTIONS FROM GENERAL FORM TO STANDARD/VERTEX FORM AND VICE VERSA
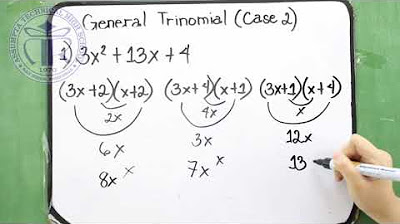
ATHS Factoring General Trinomial
5.0 / 5 (0 votes)
