completing the square with complex roots (KristaKingMath)
Summary
TLDRThis educational video demonstrates the process of solving a quadratic equation with complex roots by completing the square. It begins by simplifying the equation to isolate the quadratic term, then divides through by the coefficient to normalize it. The video guides viewers through adjusting the equation to form a perfect square trinomial, adding and subtracting the square of half the coefficient of the linear term. It concludes by taking the square root of both sides to solve for x, revealing the complex roots involving the imaginary unit i, and illustrates how to express these roots in a simplified form.
Takeaways
- 📚 Start by ensuring the quadratic equation is in descending order of terms.
- 🔍 Check if the quadratic can be factored into binomials, which is not possible in this case.
- 🔑 To complete the square, adjust the coefficient of the x² term to 1 by dividing all terms by it.
- 📉 Subtract the square of half the coefficient of the x term from both sides to complete the square.
- 📐 The result is a perfect square trinomial on the left side of the equation.
- 🧩 Factor the left side to find the square root, simplifying the equation to a binomial squared.
- ➗ Find a common denominator to combine terms on the right side of the equation.
- ➖ Subtract the constant term from both sides to isolate the binomial squared.
- 🔢 Take the square root of both sides to solve for x, considering both positive and negative roots.
- 🤔 Recognize that the square root of a fraction can be simplified by taking the square root of the numerator and denominator separately.
- 🌐 Understand that the square root of -1 is represented by the imaginary unit 'i', leading to complex roots.
- 📝 The final roots of the equation are complex numbers, expressed as a real part plus or minus an imaginary part multiplied by 'i'.
Q & A
What is the first step when solving a quadratic equation by completing the square?
-The first step is to ensure that the terms of the quadratic equation are in descending order, meaning the highest degree terms are first and the lowest degree terms are last.
Why can't the given equation 3x^2 - x + 5 = 0 be factored into two binomials?
-The equation cannot be factored into two binomials because there is no combination of factors of 3, -1, and 5 that will result in a middle term of -1.
What does it mean to complete the square for a quadratic equation?
-Completing the square is a method of solving quadratic equations by manipulating the equation to form a perfect square trinomial, which allows for easier identification of the roots.
Why is it necessary to divide every term by the coefficient of the x^2 term when completing the square?
-Dividing every term by the coefficient of the x^2 term is necessary to make the coefficient of the x^2 term equal to one, simplifying the process of completing the square.
How do you determine the value to add to both sides of the equation when completing the square?
-You take the coefficient of the x term, divide it by 2, and then square the result. This value is added to both sides of the equation to complete the square.
What is the perfect square trinomial formed after adding 1/36 to both sides of the equation?
-The perfect square trinomial formed is (x - 1/6)^2, which is derived from the expression x^2 - 1/3x + 1/36.
How do you find the roots of the equation after completing the square?
-After completing the square, you set the equation equal to zero and solve for x by taking the square root of both sides of the equation.
What does it mean when the roots of a quadratic equation involve the imaginary number i?
-When the roots involve the imaginary number i, it means that the roots are complex numbers, as i represents the square root of -1.
How can you simplify the expression involving the square root of -59?
-You can simplify the expression by recognizing that the square root of -1 is i, and then separating the square root of the product into the product of the square roots.
What are the final roots of the quadratic equation after completing the square and solving for x?
-The final roots are x = (1 + √59i)/6 and x = (1 - √59i)/6, which are complex numbers involving the imaginary unit i.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

2. Ecuaciones cuadráticas completas, solución por el Método de completar el binomio

SOLVING QUADRATIC EQUATIONS USING QUADRATIC FORMULA | Grade 9 Learning Task 3 Week 1

PERSAMAAN KUADRAT part ZERO-ONE
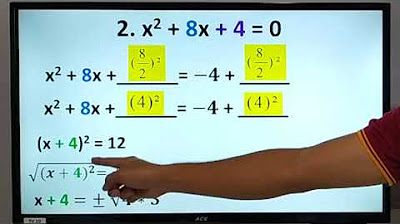
Math8 1G LV4 - Completing the Square and Quadratic Formula

How to Solve Quadratic Equations by Completing the Square? Grade 9 Math

How to Solve Quadratic Equations by Extracting the Square Root? @MathTeacherGon
5.0 / 5 (0 votes)
