The Binomial Experiment and the Binomial Formula (6.5)
Summary
TLDRThis video script offers an insightful exploration of the binomial setting and formula within the context of probability distribution. It explains the concept of binomial experiments, which involve fixed trials with two outcomes, constant success probability, and independent trials. Through examples like coin flipping and marble drawing, the script demonstrates how to calculate probabilities of specific outcomes and verifies binomial conditions. It also introduces the binomial formula as a shortcut for these calculations, emphasizing its applicability to binomial experiments only.
Takeaways
- 📚 The binomial setting and formula are discussed, focusing on the probability of success or failure in repeated experiments.
- 🔢 The prefix 'bi' signifies two outcomes, such as success or failure, in binomial probabilities.
- 🧩 Four conditions must be met for a binomial setting: fixed number of trials, two outcomes per trial, constant probability of success, and independence of trials.
- 🪙 An example of a binomial experiment is flipping a coin multiple times, calculating the probability of getting a certain number of heads.
- 🎲 The probability of getting exactly one head in three coin flips is calculated by considering all possible outcomes and their probabilities.
- 📉 Each outcome's probability is calculated using the product of the probabilities of individual flips, which in this case is 0.125.
- 📈 The total probability of getting exactly one head is the sum of the probabilities of all individual outcomes, resulting in 0.375.
- 🟢 The script checks if the coin flip experiment is binomial by confirming it satisfies all four binomial conditions.
- 🔄 Another example involves drawing marbles with replacement from a box, illustrating the binomial setting with a different context.
- 🎯 The probability of drawing exactly two green marbles from a box of ten is calculated using both direct enumeration and the binomial formula.
- 📝 The binomial formula provides a shortcut for calculating probabilities in binomial experiments, represented as 'n choose k' times the success probability raised to the power of successes, times the failure probability raised to the power of failures.
Q & A
What is the binomial probability distribution?
-The binomial probability distribution refers to the probability of a success or failure in an experiment that is repeated multiple times, resulting in two possible outcomes for each trial.
What does the prefix 'bi' signify in the term 'binomial'?
-The prefix 'bi' signifies two, as seen in words like 'bicycle' and 'binoculars', and in the context of binomial probabilities, it refers to the two outcomes: a success or a failure.
What are the four conditions that must be satisfied for a setting to be considered binomial?
-The four conditions are: 1) a fixed number of trials, 2) only two possible outcomes for each trial, 3) the probability of success must be constant for every trial, and 4) each trial must be independent of the others.
Can you provide an example of a binomial experiment?
-An example of a binomial experiment is flipping a regular coin three times, where the probability of getting heads (success) or tails (failure) remains constant and each flip is independent.
How many possible ways are there to get exactly one head when flipping a coin three times?
-There are three possible ways to get exactly one head when flipping a coin three times: H-T-T, T-H-T, and T-T-H.
What is the probability of getting exactly one head when flipping a coin three times?
-The probability of getting exactly one head when flipping a coin three times is 0.375, which is calculated by adding the probabilities of the three different outcomes (0.125 each).
What does it mean for the trials in a binomial experiment to be independent?
-For trials to be independent in a binomial experiment means that the outcome of one trial does not influence the outcome of another trial.
How does the binomial formula help in calculating probabilities in a binomial experiment?
-The binomial formula provides a shortcut for calculating probabilities by using the number of trials, the number of successes, and the probability of success, without having to list all possible outcomes.
What is the probability of drawing exactly two green marbles from a box of 10 marbles with 3 pink, 2 green, and 5 blue marbles when drawing 5 marbles with replacement?
-The probability is 0.2048, calculated using the binomial formula or by adding the probabilities of all possible outcomes where exactly two green marbles are drawn.
What is the binomial formula and how is it structured?
-The binomial formula is structured as P(k) = n choose k * (p^k) * ((1-p)^(n-k)), where k is the number of successes, n is the number of trials, p is the probability of success, and 'n choose k' is the combination formula representing the number of ways to choose k successes from n trials.
Why is it important to check if all four conditions are satisfied before applying the binomial formula?
-It is important to ensure that all four conditions are satisfied to confirm that the experiment is a binomial experiment, as the binomial formula can only be applied to binomial settings.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

Kelas XII / Distribusi Peluang Binomial

Binomial distribution # Explanation with notes# Properties of binomial distribution.

Pembahasan Contoh Soal Distribusi Binomial - Penjelasan Singkat dan Jelas
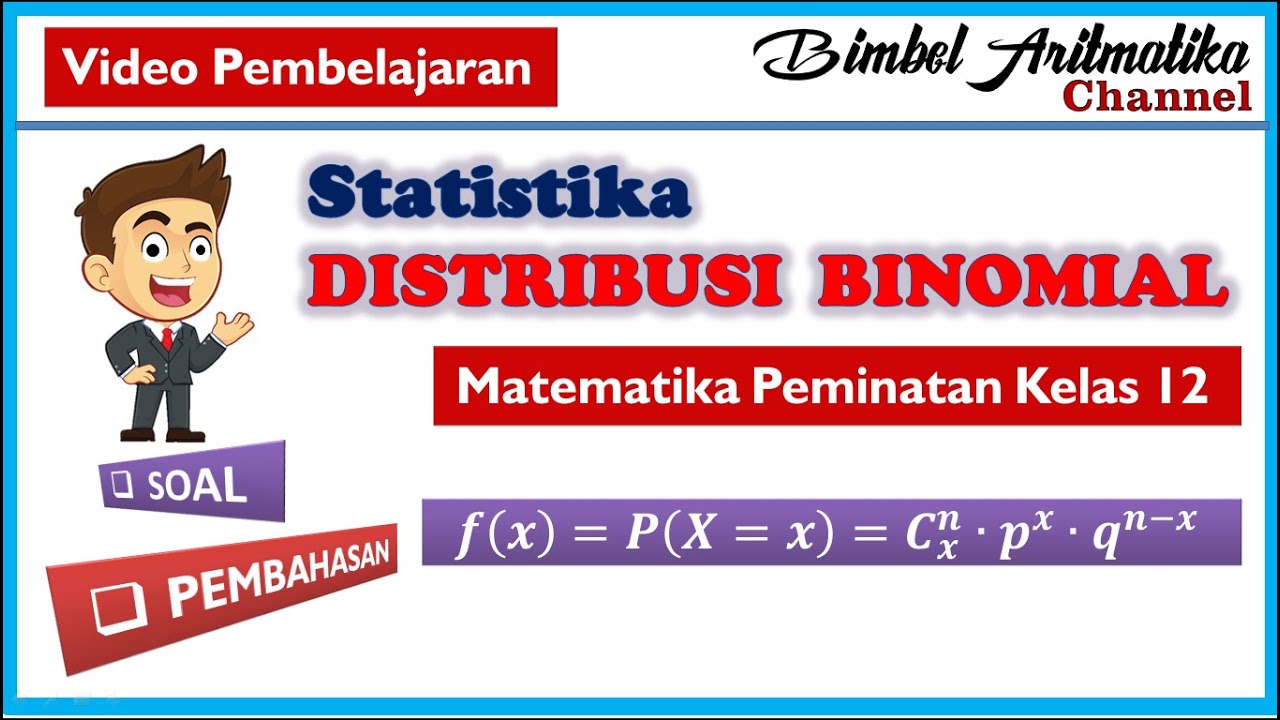
Distribusi Probabilitas Binomial | Matematika Peminatan Kelas 12

Repeated independent trial and Bernoulli distribution

KONSEP, SOAL, DAN PEMBAHASAN MATERI DISTRIBUSI BINOMIAL![MATEMATIKA PEMINATAN KELAS 12]
5.0 / 5 (0 votes)
