#173 Time response of first order control system || EC Academy

Summary

This ECE Academy lecture delves into the time response of first-order control systems, illustrating the system's block diagram and deriving its transfer function. It explains the system's order, time constant, and the Laplace transform's role in determining the system's response. The script demonstrates how the system approaches steady-state values, highlighting percentages reached at multiples of the time constant, and concludes that the steady state is only fully achieved at infinite time.

Takeaways

• 🎓 The lecture is about understanding the time response of a first-order control system.
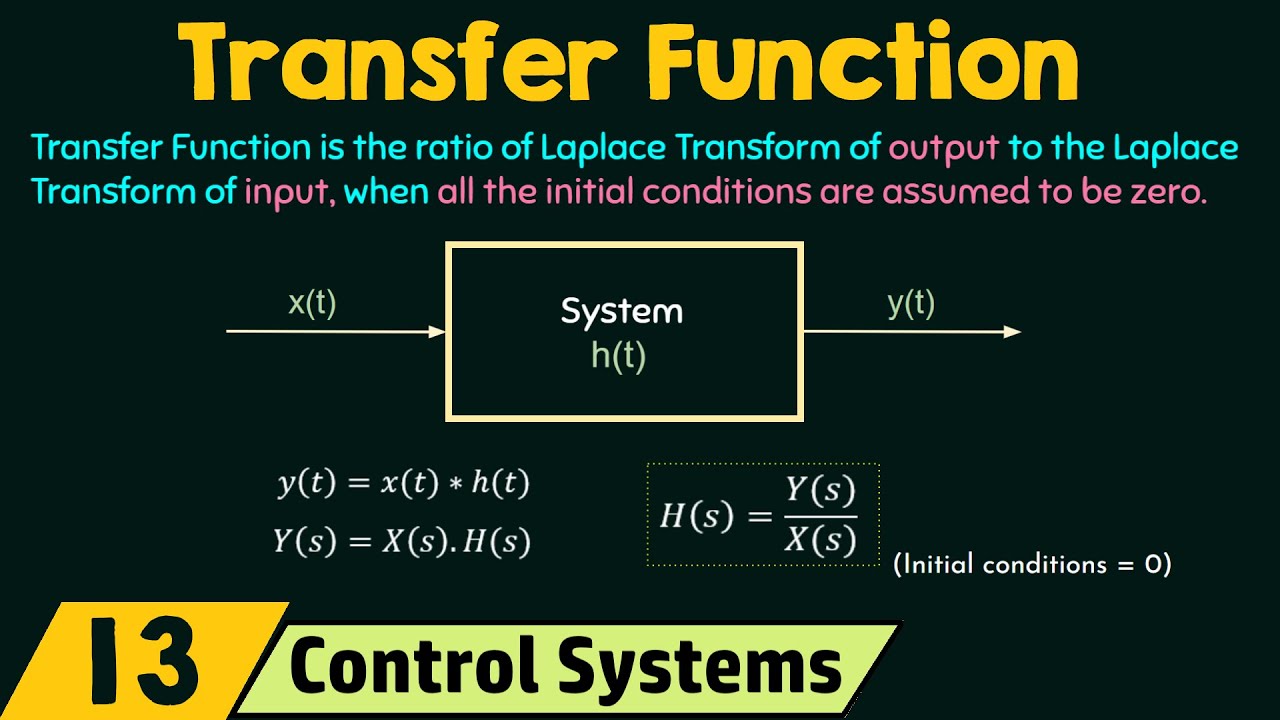
• 📈 The block diagram of a first-order control system is introduced, which includes the transfer function and components like G(s) and H(s).
• 🔍 The overall transfer function is derived as 1 / (sT + 1), indicating the system's first-order nature due to the highest power of s being 1.
• 🧩 The time constant 'T' is identified as a key parameter of the system, affecting the system's response.
• 📚 The Laplace transform of the input R(t) is explained, which is represented as R(s) = 1/s.
• 📝 Partial fraction decomposition is used to simplify the transfer function, leading to the identification of constants 'a' and 'b'.
• 🔢 The values of 'a' and 'b' are determined to be 1 and -1/T, respectively, through the application of limits.
• ⚙️ The inverse Laplace transform is applied to find the time-domain response C(t), which is an exponential function.
• 📉 The system's response is characterized by an exponential curve reaching certain percentages of the final value at multiples of the time constant 'T'.
• 🔮 At infinite time, the system reaches its steady state, with C(t) equalling the final value, demonstrating the long-term behavior of a first-order system.

Q & A

What is the transfer function of the first-order control system?

The transfer function of the first-order control system is C(s)/R(s) = 1 / (Ts + 1).

What does the variable 'T' represent in the transfer function?

'T' represents the time constant of the system.

How can the order of the system be identified from the transfer function?

The order of the system can be identified by the highest power of 's' in the denominator. In this case, the highest power is 1, indicating it is a first-order system.

What is R(s) when R(t) is equal to 1?

When R(t) is equal to 1, R(s) is the Laplace transform of R(t), which is 1/s.

How is the partial fraction decomposition of C(s) = 1 / (s(Ts + 1)) performed?

The partial fraction decomposition is performed by expressing C(s) as A/s + B/(Ts + 1) and then solving for A and B.

What are the values of A and B in the partial fraction decomposition?

The value of A is 1, and the value of B is -T.

What is the inverse Laplace transform of C(s) = 1/s - T/(s + 1/T)?

The inverse Laplace transform is C(t) = 1 - e^(-t/T).

What percentage of the final value does the exponential curve reach at T = T?

At T = T, the exponential curve reaches 63% of the final value.

What are the values of C(t) at T = 2T and T = 3T?

At T = 2T, C(t) is 0.86. At T = 3T, C(t) is 0.95.

What is the significance of T approaching infinity in the context of the first-order system?

As T approaches infinity, C(t) reaches the steady-state value of 1, indicating that the system's steady state is reached only at infinite time.