GRavity 1 Newton's Law of Gravity
Summary
TLDRThis video script covers the core principles of Newton's Law of Universal Gravitation, explaining how gravity operates between objects in the universe. The speaker delves into the historical context, mentioning Isaac Newton and his conceptualization of gravitational force. The law is explained in terms of force proportionality to mass and inversely proportional to the square of the distance between objects. Practical examples, including mathematical equations, are provided to demonstrate the concept's application. The lecturer emphasizes the importance of understanding gravity for students in engineering fields and illustrates how this fundamental principle plays a crucial role in various real-world scenarios.
Takeaways
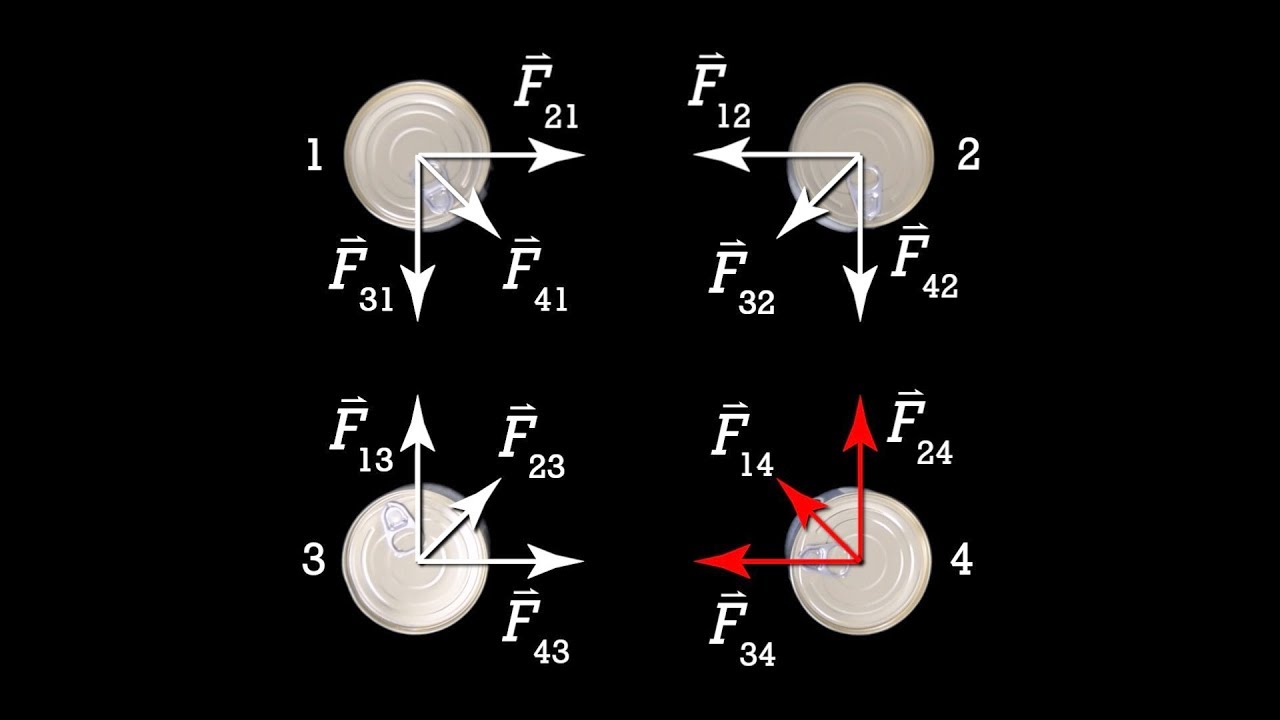
- 😀 Newton's law of universal gravitation states that every object in the universe attracts every other object with a force proportional to the product of their masses and inversely proportional to the square of the distance between them.
- 😀 The gravitational force is mathematically expressed as F = G * (m1 * m2) / r², where F is the force, m1 and m2 are the masses, r is the distance, and G is the gravitational constant.
- 😀 The gravitational constant (G) is universally constant at 6.67 × 10⁻¹¹ N·m²·kg⁻², regardless of location in the universe.
- 😀 The gravitational force between two objects is stronger when their masses are larger and weaker when the distance between them increases.
- 😀 The inverse square law means that as the distance between two objects increases, the gravitational force decreases exponentially.
- 😀 The concept of gravity applies not only to everyday objects but also governs the movement of celestial bodies like planets, moons, and satellites.
- 😀 Gravity affects the weight of objects, which changes depending on the mass of the object and the gravitational field they are in.
- 😀 Newton’s law of gravitation helped explain both terrestrial and astronomical phenomena, including the motion of the planets and the falling of an apple.
- 😀 The gravitational force calculated for everyday objects (like two people) is extremely small, making its effects practically negligible for objects of everyday scale.
- 😀 The law of universal gravitation is crucial for understanding planetary motion, satellite orbits, and space exploration, as it governs the force of attraction between massive bodies.
- 😀 The gravitational force between objects like Earth and the Moon is significant due to their large masses, whereas the force between small objects, like two people, is minimal and imperceptible.
Q & A
What inspired Isaac Newton to formulate the law of universal gravitation?
-According to the transcript, the story suggests that Newton's inspiration came when an apple supposedly fell on his head, prompting him to consider the forces involved. However, it's mentioned that this story might be fictional, and the actual inspiration could have come from his observations of the motion of celestial bodies.
How does Newton's law of gravitation relate to the motion of the moon and planets?
-Newton applied his law of gravitation to explain that the force of attraction between objects, like the Earth and the apple, is similar to the force that keeps the moon in orbit around the Earth and the planets around the Sun.
What is Newton's law of gravitation and what does it state?
-Newton's law of gravitation states that every object in the universe attracts every other object with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.
What are the key components of Newton's gravitational formula?
-The formula is F = G * (m1 * m2) / r^2, where F is the gravitational force between two masses, m1 and m2 are the masses of the two objects, r is the distance between their centers, and G is the universal gravitational constant.
What is the significance of the constant 'G' in the law of gravitation?
-'G' is called the universal gravitational constant, and it quantifies the strength of gravity in the law of gravitation. Its value is 6.67 × 10^-11 N m²/kg².
How is the gravitational force between two objects calculated?
-The gravitational force between two objects is calculated using the formula F = G * (m1 * m2) / r^2. This means you multiply the masses of the objects, divide by the square of the distance between them, and then multiply by the gravitational constant 'G'.
Why is the gravitational force between objects on Earth often very small?
-On Earth, the gravitational force between objects is usually very small because of the relatively low masses of everyday objects and the fact that the distance between them is small compared to planetary distances. For example, the force between two people standing a few meters apart is minimal.
How does the gravitational force differ between objects on Earth and larger celestial bodies?
-While the gravitational force on Earth is weak for small objects, it is much stronger between celestial bodies like planets and moons due to their massive sizes. This stronger force is responsible for planetary orbits and the movement of moons around their planets.
What is the practical application of Newton's law of gravitation in physics and engineering?
-Newton's law of gravitation is crucial in fields like astronomy, physics, and engineering. It helps explain planetary motions, satellite orbits, and even the design of space missions. Engineers need to understand gravitational forces when calculating trajectories and satellite placement.
What does the concept of 'inverse square law' mean in the context of gravity?
-The inverse square law means that the gravitational force between two objects decreases as the square of the distance between them increases. For example, if the distance between two objects doubles, the gravitational force becomes one-fourth of its original value.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahora5.0 / 5 (0 votes)