[Math 20] Lec 3.4 Fundamental Identities (1 of 2)
Summary
TLDRThis video lecture explores fundamental trigonometric identities, including reciprocal, quotient, and Pythagorean identities. The lecturer demonstrates how to use these identities to solve problems involving sine, cosine, tangent, and other circular functions. Through examples, viewers learn how to apply these identities in various quadrants, with a focus on calculating function values based on given conditions. Key concepts such as the relationship between tangent and sine, secant and cosine, and the use of Pythagorean identities for solving trigonometric equations are emphasized, providing a comprehensive understanding of fundamental trigonometry.
Takeaways
- 😀 The video introduces fundamental trigonometric identities such as reciprocal, quotient, and Pythagorean identities.
- 😀 Reciprocal identities are key, where secant is the reciprocal of cosine, cosecant is the reciprocal of sine, and cotangent is the reciprocal of tangent.
- 😀 Quotient identities are important: tangent equals sine over cosine, and cotangent equals cosine over sine.
- 😀 The Pythagorean identities are critical for solving trigonometric equations: sin²θ + cos²θ = 1, 1 + tan²θ = sec²θ, and 1 + cot²θ = csc²θ.
- 😀 When dividing by cosine squared in the Pythagorean identity, it results in 1 + tan²θ = sec²θ.
- 😀 The video emphasizes the importance of recognizing the signs of trigonometric functions based on the quadrant of the angle.
- 😀 Examples are used to demonstrate how to use fundamental identities to solve for unknown trigonometric values, such as tangent, cosine, and sine.
- 😀 A step-by-step method is shown to calculate the values of trigonometric functions using identities like the reciprocal identities and Pythagorean identities.
- 😀 The video reinforces that knowing the quadrant of the angle helps determine the correct sign for the trigonometric functions.
- 😀 Several examples illustrate how to apply the fundamental identities to calculate sine, cosine, secant, tangent, and other trigonometric functions, taking into account the correct quadrant for each case.
Q & A
What are the reciprocal identities in trigonometry?
-The reciprocal identities relate the six trigonometric functions to their reciprocals: 1) Secant (sec θ) is the reciprocal of cosine (cos θ), 2) Cosecant (csc θ) is the reciprocal of sine (sin θ), 3) Cotangent (cot θ) is the reciprocal of tangent (tan θ).
What are the quotient identities in trigonometry?
-The quotient identities express tangent and cotangent as ratios of sine and cosine: 1) Tangent (tan θ) is the ratio of sine to cosine: tan θ = sin θ / cos θ, 2) Cotangent (cot θ) is the ratio of cosine to sine: cot θ = cos θ / sin θ.
Can you explain the Pythagorean identities in trigonometry?
-The Pythagorean identities are fundamental relationships between the trigonometric functions sine, cosine, tangent, cotangent, secant, and cosecant: 1) sin² θ + cos² θ = 1, 2) 1 + tan² θ = sec² θ, 3) 1 + cot² θ = csc² θ.
How do you use the Pythagorean identity to find missing trigonometric values?
-If you know the value of one trigonometric function (e.g., sin θ or cos θ), you can use the Pythagorean identity to solve for the others. For example, given sin θ = 3/5, you can use the identity sin² θ + cos² θ = 1 to find cos θ.
What is the process to compute trigonometric functions using the reciprocal identities?
-To compute trigonometric functions using reciprocal identities, you start with a known value and apply the reciprocal relationships. For example, if cot θ = -12/5 and you know cos θ, you can use the reciprocal identity sec θ = 1/cos θ to find sec θ.
In which quadrants are the trigonometric functions positive?
-The signs of trigonometric functions depend on the quadrant: 1) In Quadrant I, all functions (sin, cos, tan) are positive. 2) In Quadrant II, sine and cosecant are positive. 3) In Quadrant III, tangent and cotangent are positive. 4) In Quadrant IV, cosine and secant are positive.
What happens when you divide the Pythagorean identity sin² θ + cos² θ = 1 by cos² θ?
-When you divide the Pythagorean identity sin² θ + cos² θ = 1 by cos² θ, you get the identity 1 + tan² θ = sec² θ. This is one of the quotient and Pythagorean identities.
How can you use the quotient identities to compute values for sine and cosine?
-You can use the quotient identities to express sine or cosine in terms of tangent or cotangent. For example, if tan θ is known, you can use the identity tan θ = sin θ / cos θ to solve for sine or cosine, depending on what is given.
What is the importance of understanding the signs of trigonometric functions in different quadrants?
-Understanding the signs of trigonometric functions in different quadrants is essential for determining the correct values for trigonometric functions based on the quadrant in which the angle lies. This helps in accurate calculations and determining the proper signs for sine, cosine, and tangent functions.
What is an example of how to apply the fundamental identities to solve trigonometric problems?
-For example, given cot θ = -12/5 and cos θ = 5/13, you can use the Pythagorean identity 1 + cot² θ = csc² θ to solve for cosecant, and then apply reciprocal identities to find secant, sine, and other functions.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados
Proving Trigonometric Identities (Tagalog/Flipino Math)
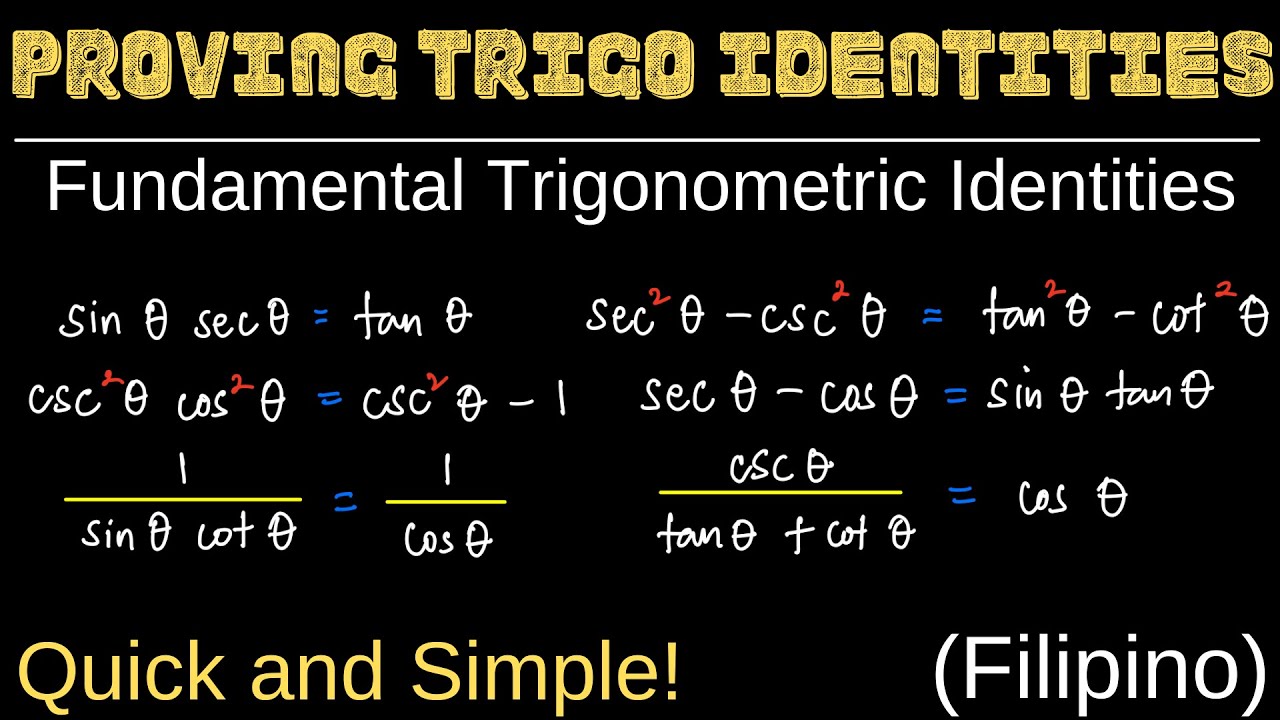
Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Sample Problems
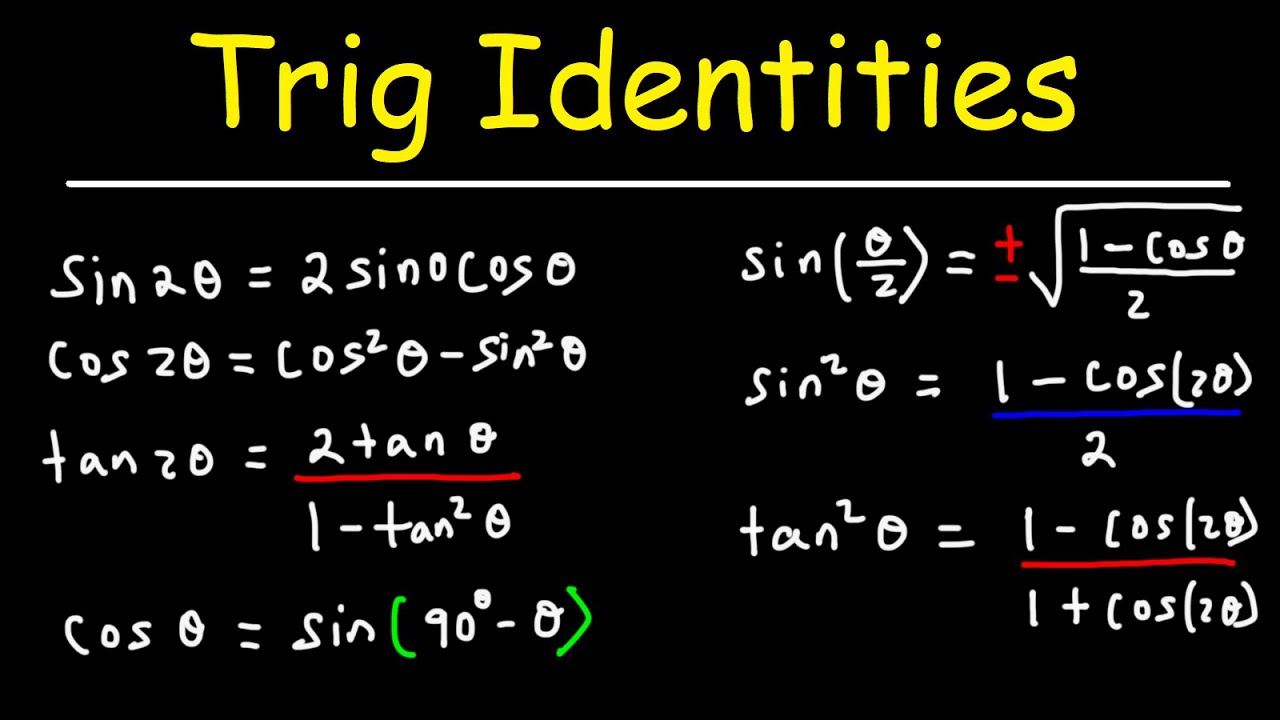
Trig Identities

Identitas Trigonometri | Matematika Wajib Kelas X

Simplifying Trigonometric Expressions

Identitas Trigonometri: Identitas Kebalikan, Perbandingan dan Pythagoras - SMA Kelas 10
5.0 / 5 (0 votes)
