Work due to Friction equals Change in Mechanical Energy Problem by Billy

Summary

In this video, Billy explains how to solve a physics problem involving the conservation of energy, friction, an incline, and a spring. The block, with an initial compression in the spring, slides along a frictionless surface before encountering a 25-degree incline. The solution involves applying the work-energy principle, factoring in kinetic friction and mechanical energy changes. Using a detailed step-by-step approach, Billy calculates the maximum height the block reaches on the incline, demonstrating the problem-solving process and emphasizing key concepts in energy conservation and frictional forces.

Takeaways

• 😀 The problem involves a block, a spring, an incline, and friction, with the goal of finding the block's maximum height after release.
• 😀 The conservation of energy principle is applied using the work-energy equation: work due to friction equals the change in mechanical energy.
• 😀 The block has initial elastic potential energy from the compressed spring, and final gravitational potential energy when it reaches maximum height.
• 😀 Friction is the key force that causes energy loss, and its work is calculated using the coefficient of kinetic friction and the normal force.
• 😀 The force of friction only acts while the block is on the incline and works against the block's motion up the incline.
• 😀 The normal force is derived from the gravitational force perpendicular to the incline, which balances out the block's motion perpendicular to the incline.
• 😀 The mechanical energy change equation is expanded to account for the work done by friction and the gravitational and spring potential energies.
• 😀 To calculate the displacement along the incline, the sine of the angle is used to relate the height to the displacement.
• 😀 After simplifying the equation, the final height is derived using a formula that includes the spring constant, the mass of the block, and the angle of the incline.
• 😀 The final calculated maximum height for the block is 0.040 meters, or 4 centimeters, after factoring in friction and spring compression.
• 😀 The process demonstrates how to combine concepts of energy conservation, friction, and forces in a real-world physics problem involving motion on an incline.

Q & A

What is the primary principle used to solve the problem in the script?

The primary principle used is the work-energy principle, which involves the work done by friction and the change in mechanical energy.

What is the role of friction in this problem?

Friction acts on the block as it moves along the incline, doing negative work that reduces the mechanical energy of the system, particularly by converting kinetic energy into heat.

What does the equation 'work due to friction = change in mechanical energy' imply in this context?

This equation implies that the work done by the frictional force is equal to the change in the system's total mechanical energy, which includes the potential and kinetic energies of the block.

How is the normal force calculated in the context of the incline?

The normal force is calculated by summing the forces in the perpendicular direction, where it equals the perpendicular component of the gravitational force acting on the block, which is mass times gravity times the cosine of the incline angle.

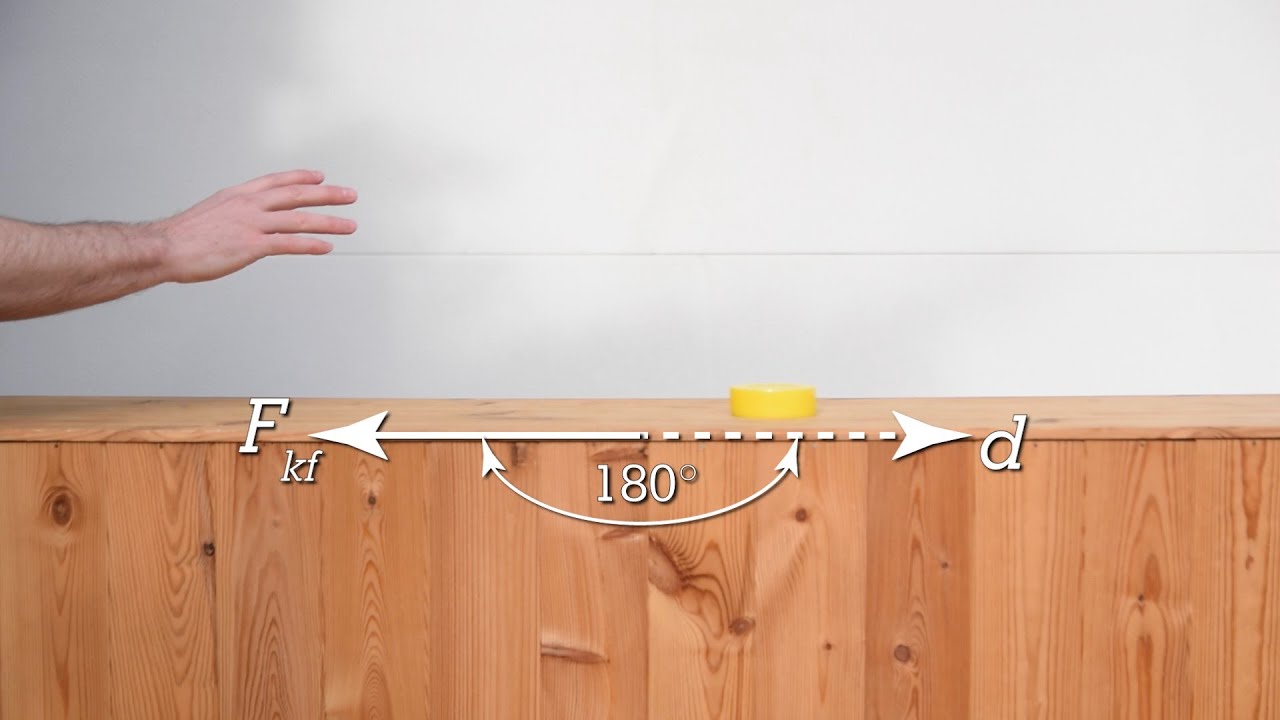
Why is the cosine of 180 degrees used in the equation for frictional work?

The cosine of 180 degrees is used because the frictional force opposes the direction of the block's displacement along the incline, making the angle between the force of friction and the displacement vector 180 degrees.

What is the significance of setting the initial point at the maximum compression of the spring?

Setting the initial point at the maximum compression of the spring allows us to start with the spring's elastic potential energy as the initial form of mechanical energy and solve for the final height where all energy is converted to gravitational potential energy and work done by friction.

How is the displacement along the incline related to the final height?

The displacement along the incline is related to the final height through the sine of the incline angle, where the displacement equals the final height divided by the sine of the incline angle.

Why do we divide by the sine of the incline angle when solving for displacement?

We divide by the sine of the incline angle to convert between the final vertical height (which we are solving for) and the displacement along the incline, as the sine of the angle relates the height to the hypotenuse of the right triangle formed by the incline.

What is the final answer for the maximum height the block reaches, and how is it obtained?

The final maximum height the block reaches is 0.040 meters (or 4 cm), which is obtained by solving the energy equation after substituting in the known values for the spring constant, mass, compression, friction coefficient, and incline angle.

Why is the final velocity of the block zero at the maximum height?

The final velocity is zero at the maximum height because at that point, all of the block's initial kinetic energy has been converted into gravitational potential energy and work done by friction, leaving the block momentarily stationary before it starts moving back down.