TEORI PITA ENERGI

Summary

This video explains the theory of energy bands in solids, contrasting it with the free electron theory. The free electron model, while effective for metals, cannot account for the behavior of insulators and semiconductors. The energy band theory introduces the concepts of the valence and conduction bands, separated by an energy gap. This gap determines a material’s electrical properties: conductors have no gap, semiconductors have a small gap, and insulators have a large one. This theory is crucial for understanding the differences in conductivity between conductors, semiconductors, and insulators.

Takeaways

• 😀 The free electron theory explains electrical conductivity by assuming electrons move freely in metals, but it has limitations in explaining the differences between conductors, insulators, and semiconductors.
• 😀 In the classical free electron theory, electrons follow a Maxwell-Boltzmann distribution, but this is replaced by the Fermi-Dirac distribution in modern theories for a more accurate description of electron behavior.
• 😀 The main limitation of the free electron theory is its assumption of a constant potential from positive ions, which fails to explain the different conductive properties of materials.
• 😀 Energy band theory overcomes the limitations of the free electron theory by considering the periodic potential experienced by electrons in the crystal lattice, leading to the formation of energy bands.
• 😀 The valence band and conduction band are the key components of the energy band model, with electrons in the valence band able to move to the conduction band under certain conditions.
• 😀 The energy gap between the valence and conduction bands explains whether a material behaves as a conductor, semiconductor, or insulator.
• 😀 Conductors have no energy gap or a very small gap, allowing electrons to flow freely in the conduction band, making them good conductors of electricity.
• 😀 Semiconductors have a small energy gap, which allows electrons to move to the conduction band under certain conditions, such as temperature or applied voltage.
• 😀 Insulators have a large energy gap, preventing electrons from moving into the conduction band, making them poor conductors of electricity.
• 😀 Quantum mechanical principles, such as the Fermi-Dirac distribution, provide a more accurate model of electron behavior in solids, especially at low temperatures, compared to classical theories.
• 😀 Energy band theory is essential for understanding the electrical properties of materials, helping to differentiate between conductors, semiconductors, and insulators based on their electronic structure.

Q & A

What is the Free Electron Theory and who proposed it?

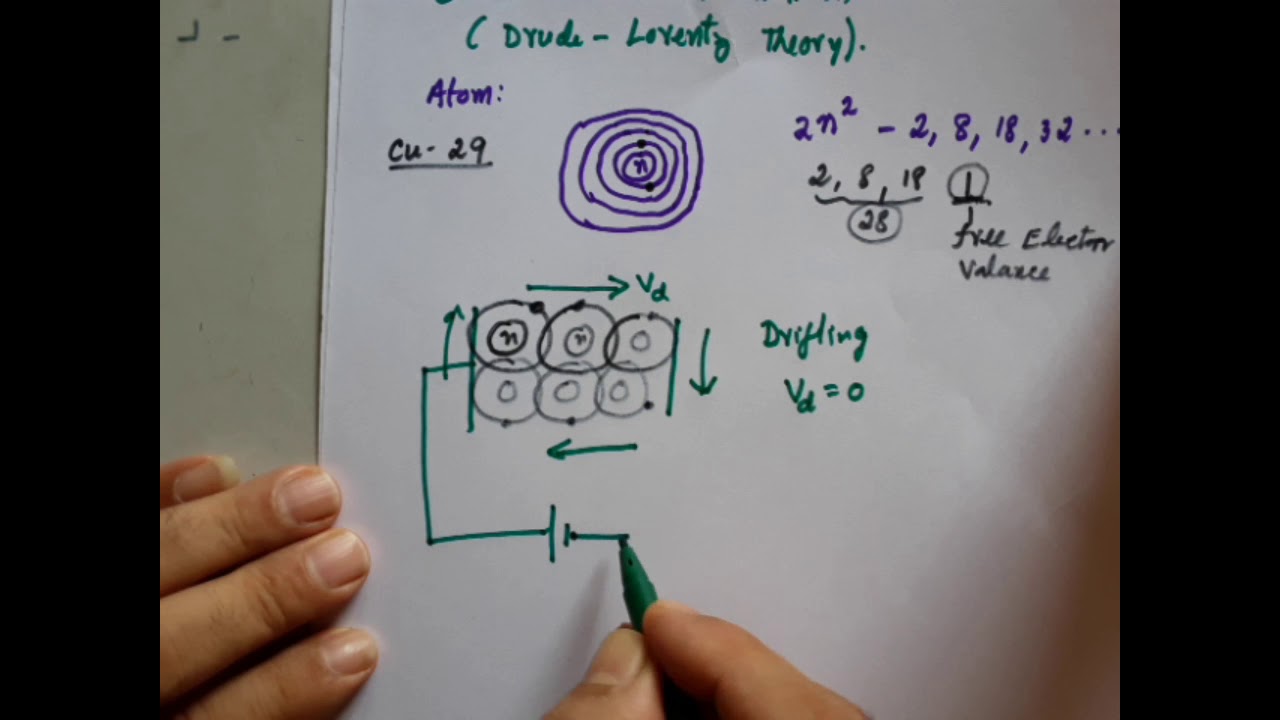
The Free Electron Theory is a classical theory that describes electrons in metals as free particles that can move without being affected by the surrounding ions. It was proposed by Drude and Lorentz, who used the Maxwell-Boltzmann distribution to explain the conductivity of metals.

What is the key limitation of the Free Electron Theory?

The key limitation of the Free Electron Theory is that it cannot explain the differences in conductivity between conductors, insulators, and semiconductors. It assumes that all metals should conduct electricity equally, which is not true in reality.

How does Sommerfeld's theory differ from the classical Free Electron Theory?

Sommerfeld's modern version of the Free Electron Theory uses the Fermi-Dirac distribution instead of the Maxwell-Boltzmann distribution. This improvement accounts for quantum mechanical effects and is more accurate in describing electron behavior in metals.

What is the role of valence electrons in electrical conductivity?

Valence electrons, or free electrons, are responsible for electrical conductivity in metals. These electrons move freely between positive ions in the metal lattice and are the primary carriers of electrical current.

Why does the Free Electron Theory fail to explain the behavior of semiconductors and insulators?

The Free Electron Theory assumes that electrons move freely under a constant potential, but this assumption does not hold true for semiconductors and insulators. These materials have significant energy gaps between the valence and conduction bands, preventing free electron movement and conductivity.

What is the Energy Band Theory and how does it improve upon the Free Electron Theory?

The Energy Band Theory explains electron behavior in solids by describing energy levels as discrete bands (valence and conduction bands) separated by an energy gap. This theory accounts for the differences in conductivity between conductors, semiconductors, and insulators, something the Free Electron Theory cannot do.

What is the energy gap (band gap) and how does it affect a material's conductivity?

The energy gap (band gap) is the difference in energy between the valence band and the conduction band. Materials with a small energy gap (semiconductors) allow electrons to move into the conduction band, while materials with a large energy gap (insulators) prevent this, making them poor conductors.

How does the Energy Band Theory describe the conductivity of conductors, semiconductors, and insulators?

In conductors, there is no energy gap between the valence and conduction bands, allowing electrons to move freely and conduct electricity. In semiconductors, the energy gap is small, and electrons can move into the conduction band under certain conditions. In insulators, the energy gap is large, preventing electron movement and thus, conductivity.

What assumption does the Energy Band Theory make about the potential experienced by electrons in a crystal?

The Energy Band Theory assumes that the potential experienced by electrons in a crystal is periodic, meaning it repeats in a regular pattern with a period equal to the lattice constant of the crystal.

How does the Fermi-Dirac distribution relate to electron behavior in metals?

The Fermi-Dirac distribution describes the probability of occupancy of energy states by electrons in a metal. At absolute zero (0 Kelvin), all energy states below the Fermi level are fully occupied, and those above it are empty. This distribution helps explain the behavior of electrons in metals, especially regarding their conductivity.