Finalizando a semana 3
Summary
TLDRThis video lesson covers key concepts from Week 3 of the Ordinary Differential Equations course. It provides a review of second-order linear differential equations, including both homogeneous and non-homogeneous cases. The lesson focuses on solving these equations using methods such as the characteristic equation, reduction of order, method of undetermined coefficients, and variation of parameters. Through examples, the video demonstrates how to handle different types of roots (real, distinct, repeated, or complex) and discusses solving techniques for both homogeneous and non-homogeneous equations. The lesson wraps up by summarizing the importance of identifying the equation type and using appropriate solution methods.
Takeaways
- 😀 The general form of a second-order ODE is: y'' + p(t)y' + q(t)y = g(t), where y'' is the second derivative, p(t) and q(t) are functions of t, and g(t) is the non-homogeneous term.
- 😀 Homogeneous second-order ODEs have the form: y'' + p(t)y' + q(t)y = 0, with the general solution being derived from the roots of the characteristic equation.
- 😀 For a homogeneous second-order ODE, the characteristic equation is: r^2 + pr + q = 0. The nature of the roots determines the form of the solution.
- 😀 If the characteristic equation has distinct real roots r1 and r2, the solution is: y(t) = C1 e^(r1 t) + C2 e^(r2 t).
- 😀 If the characteristic equation has complex conjugate roots, the solution is: y(t) = e^(λt) (C1 cos(μt) + C2 sin(μt)), where λ is the real part and μ is the imaginary part of the root.
- 😀 If the characteristic equation has repeated roots, the solution takes the form: y(t) = (C1 + C2t) e^(rt), where r is the repeated root.
- 😀 The reduction of order method transforms a second-order ODE into a first-order ODE if one solution is already known. This method involves substituting y2(t) = v(t)y1(t), where v(t) is an unknown function.
- 😀 The method of undetermined coefficients is used to solve non-homogeneous ODEs by guessing the form of a particular solution. The general solution is the sum of the homogeneous solution and the particular solution.
- 😀 The variation of parameters method is used when it is difficult to guess the form of the particular solution. It involves finding the particular solution as a linear combination of the homogeneous solutions, where the coefficients are determined by integration.
- 😀 In an example using the method of undetermined coefficients, the equation y'' - 3y' - 4y = 3e^(2t) was solved with the particular solution y_p(t) = -1/2 e^(2t), leading to the general solution y(t) = C1 e^(4t) + C2 e^(-t) - 1/2 e^(2t).
Q & A
What is a second-order ordinary differential equation (ODE)?
-A second-order ODE is an equation involving the second derivative of a function. It typically has the form Y''(t) + p(t)Y'(t) + q(t)Y(t) = g(t), where Y''(t) is the second derivative, Y'(t) is the first derivative, and Y(t) is the function itself. If the equation is homogeneous, g(t) = 0.
How does the solution of a homogeneous second-order ODE differ from a non-homogeneous one?
-For a homogeneous second-order ODE, the solution is the sum of constants multiplied by exponential terms, depending on the roots of the characteristic equation. For a non-homogeneous ODE, a particular solution is added to the homogeneous solution. The particular solution is often determined by methods like undetermined coefficients or variation of parameters.
What is the characteristic equation of a second-order linear ODE?
-The characteristic equation of a second-order linear homogeneous ODE is derived from the equation Y''(t) + p(t)Y'(t) + q(t)Y(t) = 0. It is a quadratic equation in terms of 'r', usually of the form r² + pr + q = 0. The roots of this equation determine the form of the solution.
What happens when the roots of the characteristic equation are distinct?
-When the roots of the characteristic equation are distinct, say r₁ and r₂, the solution to the homogeneous ODE is of the form Y(t) = C₁e^(r₁t) + C₂e^(r₂t), where C₁ and C₂ are constants determined by initial conditions.
What is the solution when the roots of the characteristic equation are complex?
-When the roots of the characteristic equation are complex, say λ ± iν, the solution to the homogeneous ODE is of the form Y(t) = e^(λt)(C₁cos(νt) + C₂sin(νt)), where λ is the real part and ν is the imaginary part of the roots.
How do you solve a second-order ODE when the roots of the characteristic equation are equal?
-When the roots of the characteristic equation are equal, say r₁ = r₂ = r, the solution to the homogeneous ODE is of the form Y(t) = (C₁ + C₂t)e^(rt). This accounts for the repeated root and includes a linear term in t.
What is the reduction of order method in solving ODEs?
-The reduction of order method is used to reduce a second-order ODE to a first-order ODE when one solution is already known. By substituting the second solution as a function times the first solution, the problem is transformed into a first-order equation, which can then be solved using standard first-order methods.
How is the method of undetermined coefficients applied to solve non-homogeneous ODEs?
-The method of undetermined coefficients is used to guess a form for the particular solution of a non-homogeneous ODE, based on the form of the non-homogeneous term (g(t)). After guessing the form, the solution is substituted back into the original ODE to determine the unknown coefficients.
What is the method of variation of parameters?
-The method of variation of parameters is used to solve non-homogeneous ODEs when the form of the particular solution is not easily guessed. It involves expressing the particular solution as a linear combination of the solutions to the corresponding homogeneous ODE, with the coefficients being functions of t, and then solving for those functions.
How do you find the solution to a second-order non-homogeneous ODE using the method of variation of parameters?
-To use the method of variation of parameters, first find the general solution to the associated homogeneous ODE. Then, express the particular solution as a linear combination of these solutions with undetermined functions as coefficients. Solve for these functions by substituting into the original ODE.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

GRINGS - Equações Diferenciais Ordinárias - Aula 1

Introduction to Differential Equations (Differential Equations 2)
Transform Calculus and its applications in Differential Equations

Oxford Calculus: Separable Solutions to PDEs

Pengantar Persamaan Diferensial
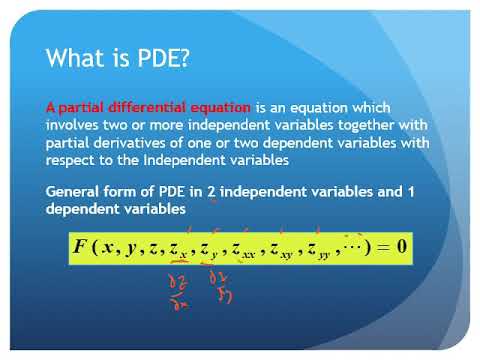
Persamaan Diferensial Parsial (Pengertian, Definisi, dan Klasifikasi)
5.0 / 5 (0 votes)
