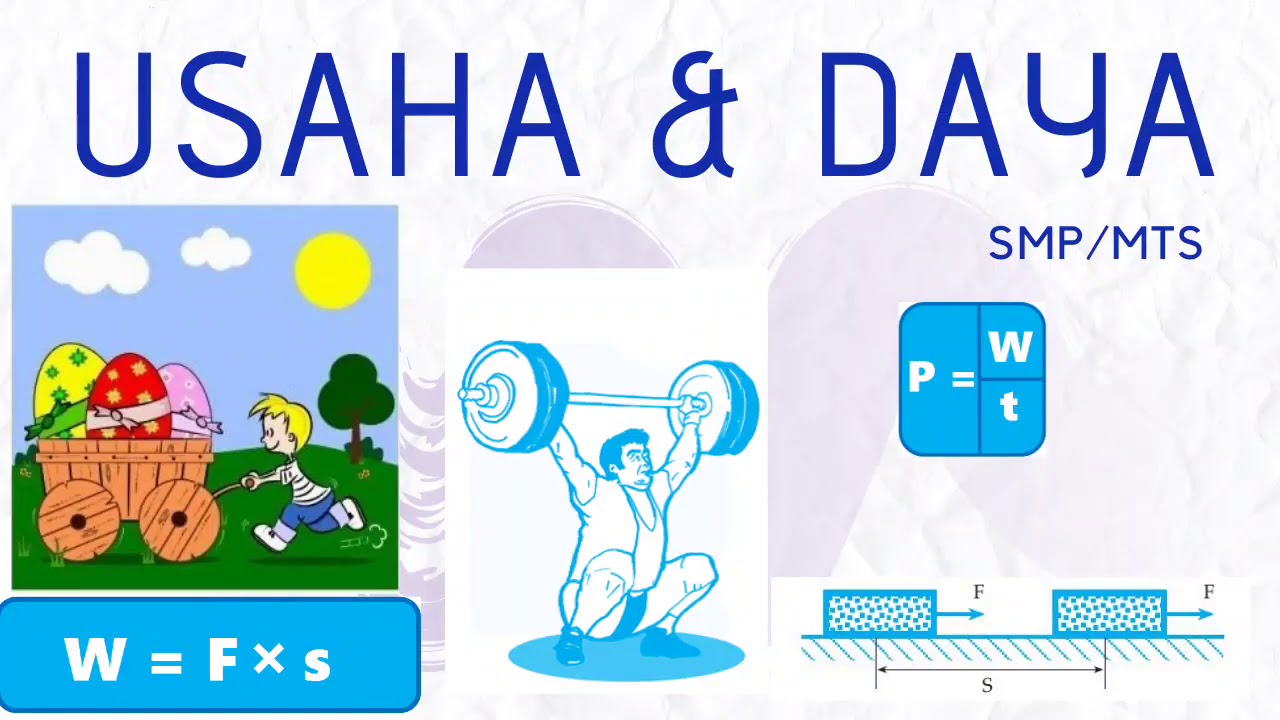
Introductory Work Problem
Summary
TLDRIn this educational video, a group of students discusses the physics of work done by a shopper pushing a cart. The shopper applies a force at a 59° angle, and they calculate the work done on the cart, taking into account the displacement and the force applied. The group clarifies common misconceptions, such as using the wrong angle and misinterpreting the direction of displacement. They also explain how forces like gravity and the normal force contribute no work due to their perpendicular nature to the displacement. The video highlights important concepts of force, work, and angles in physics in an engaging and educational manner.
Takeaways
- 😀 A shopper applies a force of 6.9 newtons at an angle of 59° to the left of the negative y-axis to push a shopping cart.
- 😀 The displacement of the cart is 7.0 meters horizontally to the left, meaning the displacement is negative in the y-axis direction.
- 😀 The work equation is given as W = F × d × cos(θ), where F is the force, d is the displacement, and θ is the angle between the force and displacement.
- 😀 The angle used in the work equation should be the angle between the applied force and the displacement of the cart, not between the force and the vertical.
- 😀 The correct angle for the work equation is 31° (90° - 59°), not 59°.
- 😀 When calculating work, use the magnitude of displacement as a positive value (+7.0 meters) and adjust for the cosine of the angle.
- 😀 The work done by the shopper on the cart is 41 joules, calculated by using the correct angle (31°) in the work equation.
- 😀 Work done by gravity and normal forces is zero because the angle between these forces and the displacement is 90° (cos(90°) = 0).
- 😀 The force of friction's work cannot be determined because the mass of the cart is unknown.
- 😀 Understanding angles and their relationship to forces and displacement is crucial for correctly calculating work in physics problems.
- 😀 Common student mistakes include misinterpreting the angle in the work equation and misusing negative displacement when calculating work.
Q & A
What is the key concept being taught in the video script?
-The key concept is how to calculate the work done by a force on an object, with an emphasis on understanding the relationship between force, displacement, and the angle between them.
What is the formula for work in physics?
-The formula for work is: Work = Force × Displacement × Cosine(θ), where θ is the angle between the direction of the force and the direction of displacement.
What mistake did the students initially make when calculating the work done?
-The students initially used the wrong angle (59 degrees) in their calculation, but the correct angle between the force and displacement was 31 degrees.
How do you determine the angle used in the work equation?
-The angle used in the work equation should be the angle between the direction of the applied force and the direction of displacement. In this case, it was 90 degrees minus 59 degrees, which gives 31 degrees.
Why is the displacement considered positive in the work equation, even if the cart moves to the left?
-The displacement is considered positive because the cosine factor in the work equation determines whether the work is positive or negative, not the sign of the displacement itself.
What does the cosine of the angle between force and displacement determine in the work equation?
-The cosine of the angle between the force and displacement determines the magnitude of the component of the force that contributes to the work done. A smaller angle results in more work being done in the direction of displacement.
Why is no work done by the force of gravity on the cart?
-No work is done by the force of gravity because the angle between the gravitational force (which is vertical) and the horizontal displacement is 90 degrees, and the cosine of 90 degrees is zero.
How is the normal force involved in the calculation of work on the cart?
-The normal force is perpendicular to the direction of displacement, meaning the angle between them is 90 degrees. Since the cosine of 90 degrees is zero, the work done by the normal force is also zero.
What would have happened if the correct value for the displacement was not used in the calculation?
-If the displacement value had been used incorrectly (as negative seven meters instead of positive seven meters), the result could have been an incorrect sign for the work done, even though the magnitude would still be accurate.
Why is it important to draw a free-body diagram in problems involving forces and work?
-Drawing a free-body diagram helps visualize the different forces acting on the object and their directions, allowing for accurate analysis and calculation of the work done by each force.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahora5.0 / 5 (0 votes)