Factor Theorem |G10 Q1 W6
Summary
TLDRThe video explains how to determine if a polynomial is divisible by a binomial, specifically using synthetic division. It walks through three examples: checking if polynomials are divisible by x-2 and x-4, and using synthetic division to confirm if these are factors. The speaker demonstrates how to find remainders and establish whether binomials are factors of given polynomials. The video concludes by encouraging viewers to like, share, and subscribe, ensuring they understood the basic concept of polynomial factorization.
Takeaways
- 📚 The video focuses on polynomial factorization and the relationship between roots and factors.
- 🧮 If 'c' is a zero of a polynomial p(x), then (x - c) is a factor of p(x).
- 🔄 Conversely, if (x - c) is a factor of p(x), then 'c' is a root of the polynomial.
- 🤓 Example 1 demonstrates checking if x - 2 is a factor of the polynomial p(x) = 5x³ + 4x² - 31x + 6 using synthetic division.
- 🧑🏫 Synthetic division is applied by bringing down coefficients, multiplying, and adding to find the remainder.
- ✅ If the remainder is zero, then x - 2 is a factor of the polynomial.
- 🔢 Example 2 explores whether x - 2 is a factor of a different polynomial, x⁴ + x³ - x² - x - 18.
- 📏 Example 2 uses direct substitution and remainder calculation to verify factor status.
- 🚫 Example 3 checks if x - 4 is a factor of the polynomial x³ - 5x² - 9x - 8, finding that it is not because the remainder is not zero.
- 👍 The video encourages viewers to like, share, and subscribe at the end.
Q & A
What does it mean when a polynomial \( p(x) \) has a zero at \( c \)?
-If \( p(x) \) has a zero at \( c \), it means that \( p(c) = 0 \), and \( x - c \) is a factor of \( p(x) \).
How can we verify if \( x - 2 \) is a factor of \( 5x^3 + 4x^2 - 31x + 6 \)?
-We can use synthetic division to divide \( 5x^3 + 4x^2 - 31x + 6 \) by \( x - 2 \). If the remainder is zero, then \( x - 2 \) is a factor.
What are the steps for performing synthetic division?
-1. Write down the coefficients of the polynomial. 2. Bring down the first coefficient. 3. Multiply it by the root, add it to the next coefficient, and continue the process. 4. If the final remainder is zero, the divisor is a factor.
In example 1, what are the results of the synthetic division of \( 5x^3 + 4x^2 - 31x + 6 \) by \( x - 2 \)?
-After performing synthetic division, the remainder is zero, which means \( x - 2 \) is a factor of the polynomial.
How do you determine if \( x - 2 \) is a factor of the polynomial \( x^4 + x^3 - x^2 - x - 18 \)?
-By substituting \( x = 2 \) into the polynomial, if the result is zero, then \( x - 2 \) is a factor.
What is the result when substituting \( x = 2 \) into \( x^4 + x^3 - x^2 - x - 18 \)?
-Substituting \( x = 2 \) gives a value of zero, confirming that \( x - 2 \) is a factor of the polynomial.
How can you verify if \( x - 4 \) is a factor of \( x^3 - 5x^2 - 9x - 8 \)?
-You can perform synthetic division. If the remainder is zero, then \( x - 4 \) is a factor.
What is the result of the synthetic division of \( x^3 - 5x^2 - 9x - 8 \) by \( x - 4 \)?
-The remainder is not zero, meaning \( x - 4 \) is not a factor of the polynomial.
What does it mean if the remainder in synthetic division is not zero?
-If the remainder is not zero, it means the divisor is not a factor of the polynomial.
What is the importance of synthetic division in determining polynomial factors?
-Synthetic division is a quick method to check if a given linear expression is a factor of a polynomial, based on whether the remainder is zero.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados
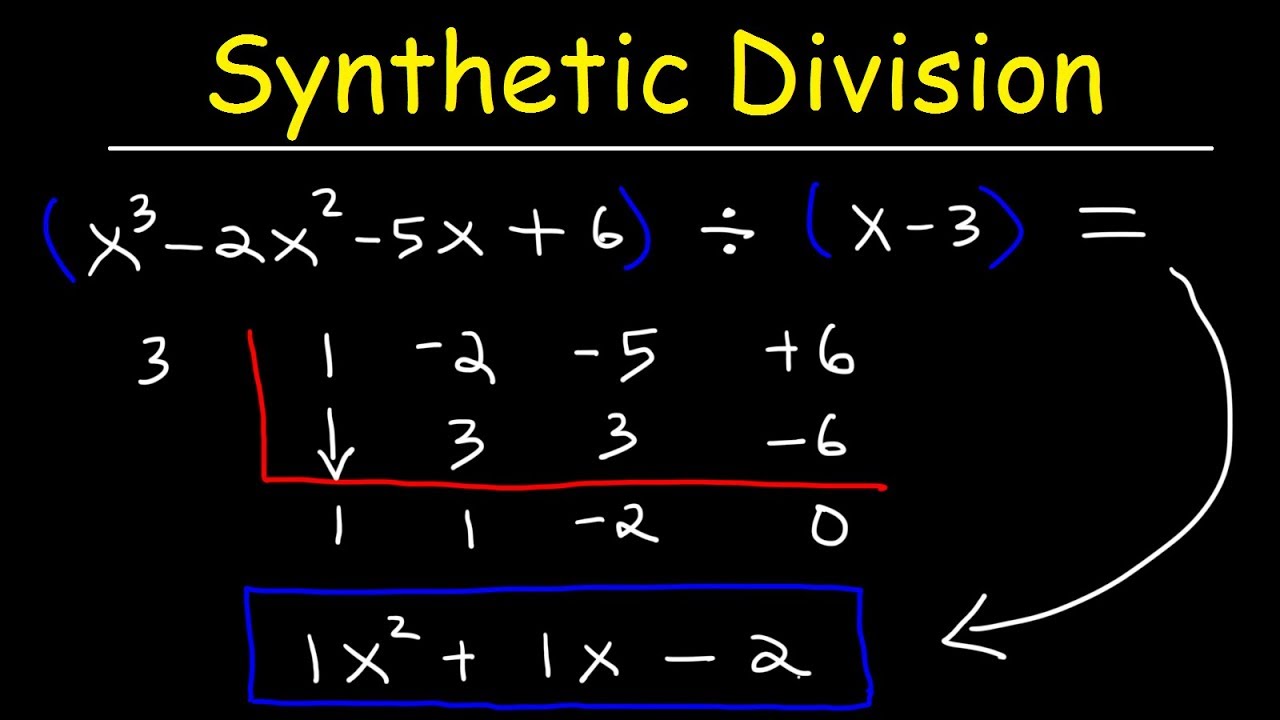
Synthetic Division of Polynomials

RÁPIDO e FÁCIL | BRIOT RUFFINI | divisão de polinômios

Lesson 33 Long Division to Find Roots
Polynomials - Adding, Subtracting, Multiplying and Dividing Algebraic Expressions
UNDERSTAND, DESCRIBE, AND INTERPRET GRAPHS OF POLYNOMIAL FUNCTIONS | GRADE 10 MATH | TAGLISH

Polinomial (Bagian 4) - Teorema Sisa dan Teorema Faktor
5.0 / 5 (0 votes)
