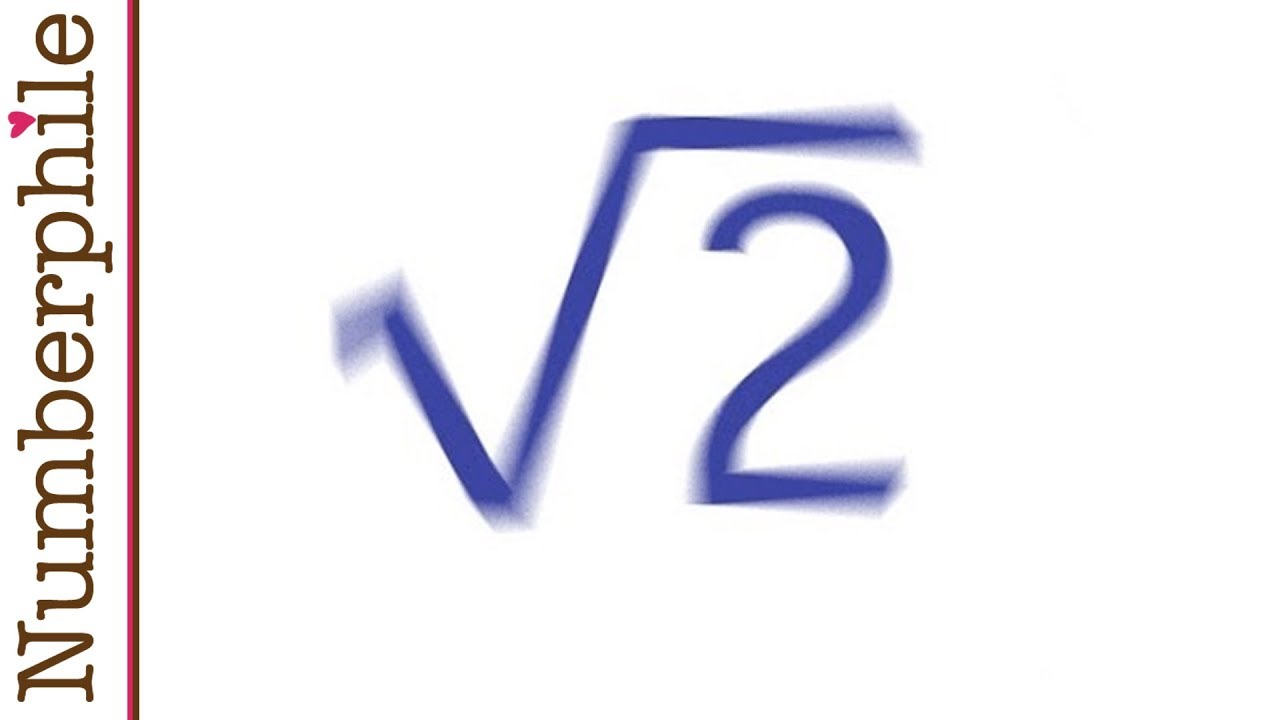DEMONSTRATION : √2 est irrationnel - Seconde

Summary

This video script explores the irrationality of the square root of 2. It begins by defining rational numbers, exemplified by one-third, which can be expressed as a repeating decimal. In contrast, irrational numbers, like pi, have non-repeating, non-terminating decimal expansions. The script then introduces a proof by contradiction to demonstrate that the square root of 2 cannot be expressed as a fraction of two integers, leading to the conclusion that it is indeed an irrational number.

Takeaways

• 📘 The video discusses the irrationality of the square root of 2.
• 🔢 A rational number is defined as one that can be expressed as a fraction of two integers, such as one-third (1/3).
• 📏 Irrational numbers, in contrast, cannot be expressed as a simple fraction; their decimal representation is non-repeating and infinite, like the number pi (π).
• 🤔 The video aims to prove that the square root of 2 is irrational by assuming the opposite and seeking a contradiction.
• 📉 The proof uses a method of contradiction, assuming that the square root of 2 is rational and then deriving an absurdity from this assumption.
• 🔄 The assumption leads to the expression of the square root of 2 as a fraction a/b, where a and b are integers with no common divisors.
• 🔢 When squared, the equation a^2 = 2b^2 is derived, and by manipulating this equation, it's shown that both a and b must be even numbers.
• 🔄 This leads to a contradiction because it implies that a and b have a common divisor (2), which contradicts the initial assumption that they are coprime.
• 📚 The video emphasizes the importance of understanding the properties of numbers, such as the fact that the square of an odd number is always odd.
• 🏁 The conclusion is that the square root of 2 is irrational, as the initial assumption that it could be expressed as a fraction leads to a contradiction.

Q & A

What is the definition of an irrational number as explained in the video?

An irrational number is a number that cannot be expressed as a simple fraction, meaning it cannot be written in the form of a ratio of two integers. When written in decimal form, it has a non-repeating, non-terminating pattern.

Can you provide an example of a rational number given in the video?

Yes, one-third (1/3) is given as an example of a rational number because when divided, it results in a repeating decimal pattern of '0.3333...' indefinitely.

What is the approximate value of the irrational number pi mentioned in the video?

The approximate value of pi given in the video is 3.14, but it is emphasized that the actual value of pi continues indefinitely without a pattern.

What method is used in the video to prove that the square root of 2 is irrational?

The video uses a proof by contradiction, assuming that the square root of 2 is rational and then showing that this assumption leads to a contradiction, thus proving that the square root of 2 is irrational.

What does it mean for two numbers to be 'co-prime' as discussed in the video?

Two numbers are 'co-prime' or 'relatively prime' if they have no common divisors other than 1, meaning they cannot be simplified further.

Why does the video suggest that a and b in the expression of the square root of 2 as a rational number must be co-prime?

The video suggests that a and b must be co-prime because any fraction can be simplified to its simplest form, which is when the numerator and denominator are co-prime.

What property of squares is used in the video to deduce that a must be even if a^2 = 2b^2?

The video uses the property that an even number is a multiple of 2. Since a^2 equals 2 times an integer (2b^2), it implies that a^2 is even, and therefore a must also be even.

How does the video demonstrate that both a and b cannot be even, leading to a contradiction?

The video shows that if a and b are both even, then they would have a common divisor (2), which contradicts the initial assumption that a and b are co-prime.

What is the conclusion of the proof in the video regarding the rationality of the square root of 2?

The conclusion is that the square root of 2 is not rational, as the assumption that it is rational leads to a contradiction, proving it must be irrational.

Outlines

00:00

📐 Introduction to Irrational Numbers

This paragraph introduces the concept of irrational numbers through the example of the square root of 2. It contrasts rational numbers, which can be expressed as fractions of integers (like one-third, which can be written as a repeating decimal), with irrational numbers, which cannot be expressed as fractions. The square root of 2 is proposed as an example of an irrational number, and the historical example of pi is also mentioned. The explanation sets up for a proof by contradiction, suggesting that if the square root of 2 were rational, it could be expressed as a fraction of two integers. However, the proof will aim to show that this leads to a contradiction, thereby proving the irrationality of the square root of 2.

05:02

🔍 Proof by Contradiction of the Irrationality of the Square Root of 2

The paragraph delves into a proof by contradiction to demonstrate the irrationality of the square root of 2. It begins by assuming that the square root of 2 is rational, which implies it can be expressed as a fraction of two integers, a and b, which are in their simplest form (co-prime). The proof then squares both sides of the equation to derive that 'a' squared equals 2 times 'b' squared. Since 'a' squared is even (as it equals 2 times an integer), 'a' itself must be even, leading to the conclusion that 'a' can be written as 2 times some integer 'k'. Substituting back, the equation becomes 4 times 'k' squared equals 2 times 'b' squared, which simplifies to 'b' squared equals 2 times 'k' squared. This implies 'b' is also even, leading to a contradiction because it suggests both 'a' and 'b' could be further simplified, which contradicts the initial assumption that 'a' and 'b' are in their simplest form with no common divisors. This contradiction proves that the initial assumption that the square root of 2 is rational is false, thus establishing its irrationality.

Keywords

💡Irrational Number

An irrational number is a real number that cannot be expressed as a ratio of two integers, meaning it cannot be written in the form of a fraction. In the video, the concept is central as it is used to explain the nature of the square root of 2. The video provides an example of pi as a commonly known irrational number, which cannot be expressed as a repeating or terminating decimal and continues indefinitely without a pattern.

💡Rational Number

A rational number is any number that can be expressed as the quotient or fraction p/q of two integers, with the denominator q not equal to zero. The video script uses the example of one-third (1/3) to illustrate a rational number, which can be written as a repeating decimal 0.333..., indicating a pattern that continues indefinitely.

💡Square Root of 2

The square root of 2 is a number that, when multiplied by itself, gives the result of 2. The video's main focus is to demonstrate that the square root of 2 is an irrational number. This is done through a proof by contradiction, assuming the opposite and showing that it leads to an absurdity.

💡Proof by Contradiction

Proof by contradiction is a common mathematical technique where one assumes the opposite of what they want to prove and then shows that this assumption leads to a contradiction. In the video, this method is used to demonstrate the irrationality of the square root of 2, by assuming it is rational and deriving a contradiction.

💡Prime Numbers

Prime numbers are natural numbers greater than 1 that have no positive divisors other than 1 and themselves. The video mentions that in the assumed rational form of the square root of 2 (a/b), both a and b could be prime numbers to ensure the fraction is in its simplest form, without common divisors.

💡Reduced Fraction

A reduced fraction is a fraction in which the numerator and denominator have no common factors other than 1, making it the simplest form of the fraction. The video script explains that if a and b are assumed to be in simplest form, they should not have any common divisors, which is a key point in the proof.

💡Even Numbers

Even numbers are integers that are exactly divisible by 2. In the video, the concept is used to show that if a number squared is even, then the number itself must also be even, leading to a contradiction in the proof that the square root of 2 is rational.

💡Odd Numbers

Odd numbers are integers that are not divisible by 2. The video uses the property that the square of an odd number is always odd to further the proof by contradiction, showing that both a and b cannot be odd if their ratio is supposed to represent the square root of 2.

💡Decimal Expansion

The decimal expansion of a number is the representation of the number in base 10, showing the digits after the decimal point. The video discusses how rational numbers have a decimal expansion that either terminates or repeats, while irrational numbers have a non-repeating, non-terminating decimal expansion.

💡Multiples

Multiples are numbers that can be obtained by multiplying a number by an integer. The video uses the concept of multiples to show that if a square number is a multiple of 2, then the original number must also be a multiple of 2, which is used in the proof to establish that both a and b are even, leading to a contradiction.

Highlights

Definition of irrational numbers and rational numbers.

Example of a rational number: one-third, explained as a repeating decimal.

Introduction of irrational numbers with the example of the number pi.

Explanation of the vague definition of irrational numbers and the need for a clearer definition.

Rational numbers are expressed as a fraction of two integers, a and b.

Irrational numbers cannot be expressed as a fraction of two integers.

The method of proof by contradiction to demonstrate the irrationality of the square root of 2.

Assumption that the square root of 2 is rational leads to a contradiction.

If the square root of 2 is rational, it can be expressed as a/b where a and b are coprime integers.

Squaring the equation a/b = √2 leads to a new equation a^2 = 2b^2.

Analysis showing that a^2 is an even number implies a is also even.

If a is even, it can be expressed as 2k where k is an integer.

Substituting a with 2k in the equation leads to 4k^2 = 2b^2.

Solving for b^2 shows that b^2 is also an even number, implying b is even.

The contradiction arises when both a and b are shown to be even, contradicting their initial assumption of being coprime.

Conclusion that the square root of 2 is irrational based on the reached contradiction.

End of the demonstration with a summary of the proof.

Transcripts

bonjour dans cette vidéo nous avons

effectué et étudier une démonstration de

l'irrationalité de racines de 2 alors

déjà qu'est ce qu'un nombre irrationnel

et pour répondre à cette question il

faudrait savoir ce qu'est un nombre

rationnel alors un nombre rationnelle

pas comme en français c'est un nombre

que je comprends que je comprends au

sens décimales je vais donner un exemple

très simple de nombreux rationnelle un

tiers est un nombre rationnelle parce

que lorsque je divise un par 3 c'est une

suite 2,3 après la virgule 0,3 à 3 3 à 3

et ça se poursuit comme ça indéfiniment

alors je ne peux pas l'écrire sous sa

forme décimales un tiers mais je le

comprends sous sa forme décimales dans

le sens où je sais que il se poursuit

comme ça avec une infinité de 3,1 nombre

irrationnel et bien c'est pas ça un

nombre irrationnel

quand on tente de l'écrire sous sa forme

décimales on trouve une suite de

décimales qui se suivent mais sans suite

logique ça ne se répète pas on peut pas

dire parce que là j'ai un cadre derrière

j'aurai un set ça c'est pas vrai on en

connaît un de nombre irrationnel depuis

longtemps c'est le nombre pi dont on

donne habituellement une valeur approché

3,14

mais on c'est derrière que j'ai encore

1,1 1,5 1,9 et ça se poursuit comme ça

de façon indéfinie et bien ce nombre là

est un nombre irrationnel mais enfin

cette définition elle est un peu vague

on pourrait apporter une définition un

peu plus claire de ce qu'est un nombre

rationnelle parce que du coup si on sait

ce qu'est un nombre rationnelle et bien

on sait ce que c'est qu'un nombre

irrationnel et comme ça on pourra

prouver que racine de 2 et irrationnel

alors un homme rationnel s'écrit sous

cette forme à sur b avec a et b qui sont

des entier on se souvient tout à l'heure

de notre un tiers 1 et 3 sont des entier

un tiers est bien un nombre rationnelle

et bien un nombre rationnelle peut

toujours s'écrire sous la forme assure b

a et b entier positifs négatifs mais en

tout cas ce sont des entier

donc un nombre irrationnel c'est un

nombre qui ne peut pas s'écrire sous

cette forme

il vaudrait donc démontrer que notre

racines de deux ne peut pas s'écrire

sous la forme à sur b a et b entier et

bien là tout naturellement le principe

de la démonstration va être d'utiliser

un raisonnement par l'absurde c'est à

dire qu'on va supposer

au départ le contraire de ce qu'on veut

démontrer et l'objectif sera d'aboutir à

la fin à une contradiction à une

absurdité par rapport au contexte de

l'exercice donc ici si on va supposer

contraire on va supposer que racine de 2

et rationnel on est d'accord que c'est

faux mais c'est là le principe de la

démonstration par l'absurde et en

supposant que racine de 2 et rationnel

on devrait aboutir à la fin quelque

chose de faux si tel est le cas et bien

ça voudra dire que notre hypothèse de

départ est également fausse et donc

racine de deux n'est pas rationnel c'est

à dire racines 2 et irrationnel alors

c'est parti

supposons donc que racine de 2 est un

nombre rationnel alors si on suppose que

racine carrée de 2 et rationnelle cela

signifie que racine de 2 peut s'écrire

sous la forme assure b avec a et b qui

sont des sentiers mais on va aller plus

loin dans l'écriture rationnelle de

racine carrée de deux en fait

a et b on va même pouvoir dire que ce

sont des nombres premiers entre eux

pourquoi lorsqu'on a une fraction je

prends par exemple infractions 16 sur 18

il est toujours possible de simplifier

la fraction pour la rendre irréductible

et pour cela il faut récupérer des

diviseurs commun entre le numérateur et

le dénominateur ici un diviseur commun

ces deux ce qui veut dire que je peux

simplifiée par deux et cela va me donner

8 sur 9

ensuite on regarde si on peut continuer

mais en tous les cas il est toujours

possible d'aller le plus loin possible

pour rendre notre fraction irréductible

et quand notre fraction est irréductible

cela signifie que le numérateur et le

dénominateur non plus de diviseur commun

je peux plus les simplifier et donc

s'ils n'ont plus de diviser

commun ça veut dire qu'ils sont premiers

entre eux donc on peut supposer que

l'écriture de racine carrée de deux sous

la forme assure b est avec a et b des

entiers premier entre eux on imagine

qu'en réalité on a notre racines de 2

qui a été écrit sous sa forme la plus

simple possible

est ce si on en aura besoin dans la

suite de la démonstration

alors du coup on va poursuivre en

mettant tout ça au carré si racines de

deux égal à sur b cela signifie que

racine de 2 ou qui a ré est égal à ce

assure b au carré racines de deux au

carré bien ça fait 2 assure b le tout au

carré ça fait à carey sur becquart et

donc on a deux qui est égal à akkar et

sur becquart et je l'écris dans l'autre

sens soit à cadrer sur becquart est égal

à 2 c'est la même chose tout simplement

maintenant pour multipliées de part et

d'autre part b carré et de cette façon

là j'obtiens une nouvelle égalité qui

est à carrer égal 2 beccari alors en

voyant ceux ci eh bien on peut en

déduire que notre carré à gauche est un

nombre pair pourquoi ça parce que à

carey est égal à 2 x 1 entier autrement

dit à carrer ces 2 x quelque chose donc

à caresses et un multiple de 2 donc si

c'est un multiple de 2 on est bien

d'accord que à carrer père et bien ceci

va entraîner que à et perd alors ça

c'est moins des moins évident pour le

comprendre il faudrait se souvenir d'une

propriété qui nous dit que six as et un

père alors un carré et un perce d'une

propriété qui fait partie également des

démonstrations à connaître et qui a été

vu un petit peu plus tôt dans la leçon

du coup et bien si a été un père cela

signifierait que à carey serait un père

donc c'est pas possible puisque à

carhaix et père bon bah du coup y'a pas

le choix à et perd donc on en arrive au

fait que à et père et 6 ha et perd on re

applique la règle de tout à l'heure sur

les multiples cela signifie que à peut

s'écrire sous la forme de x 1 entier

cet anti on va l'appeler cas et on va

dire que ça peut s'écrire deux cas donc

à égal deux cas

en revenant un autre égalité de tout à

l'heure à carrer galles 2b carré eh bien

on peut remplacer maintenant à part deux

cas on obtient comme ça une nouvelle

égalité qui sera 2 k o car est égal de

becquart et en écrivant un petit peu

autrement de chaos carrés ces deux cas

fois de cas soit 4 carrés dont 4 cas

carré égal 2 b au carré on divise de

part et d'autre part deux et on obtient

deux cas carré et galbées carré qu'on

écrit dans l'autre sens soit becquart

est égale deux cas carré et on réutilise

une troisième fois la règle du multiple

on à becca carré en ab carré pardon qui

est égal à 2 x 1 entier autrement dit b

carré est un nombre pair

eon rae applique la règle de tout à

l'heure et bien comme b carré et perd

cela entraîne que b est également un

nombre pair et là on arrive à quoi et

bien là on arrive à une absurdité

il ya un problème et oui on vient de

prouver que à et perd et que b et perd

également mais souvenons nous de ce

qu'on avait dit au départ on avait dit

que a et b son premier d'entre eux on

avait dit que a et b correspondent à la

fraction simplifier au maximum

mais s'ils sont tous les deux pères ça

voudrait dire que je peux encore

simplifier cette fraction ça c'est pas

possible puisque on a posé au départ que

a et b ne peuvent plus être divisé a et

b non pas diviseurs commun et pourtant

là on vient démontrer que a et b ont un

diviseur commun ces deux eh bien on

aboutit on aboutit à une absurdité

cela signifie donc que l'hypothèse de

départ est fausse soit racines de deux

n'est pas rationnelle et donc racine de

2 est irrationnel cqfd

cette séquence est terminée

[Musique]