Venn Diagram and Sets || Mathematics in the Modern World
Summary
TLDRIn this educational video, Ram from Matoklasan explores the concept of sets and their graphical representation through Venn diagrams, introduced by John Venn in 1881. He explains the universal set and how to represent sets within it using circles or geometrical figures. The video delves into subsets and proper subsets, using Venn diagrams to illustrate their relationships. Ram provides examples to clarify these concepts and introduces the power set, explaining its calculation and application. The video concludes with a tip on determining the number of subsets in a set and encourages viewers to subscribe for more informative content.
Takeaways
- 📚 Sets can be visually represented using Venn diagrams, named after John Venn who introduced them in 1881.
- 🎯 The universal set 'U' in a Venn diagram contains all objects under consideration and is depicted by a rectangle.
- 🔵 Circles or other shapes within the rectangle represent individual sets, and points within these shapes symbolize set elements.
- ➡ Venn diagrams are used to illustrate relationships between sets, such as the set of vowels in the English alphabet.
- 🔶 A subset is a set where every element of set A is also in set B, denoted by A ⊆ B.
- 🔷 A proper subset occurs when A is a subset of B, but A is not equal to B, denoted by A ⊂ B.
- 🔄 The concepts of subset and proper subset are analogous to 'less than or equal to' and 'less than', respectively.
- 🔑 Every set is a subset of itself, and the empty set is a subset of any set, which is a fundamental theorem in set theory.
- 💡 The power set of a set contains all possible subsets, including the empty set and the set itself.
- 🔢 The number of subsets in a set with 'n' elements is calculated as 2^n, which includes all combinations from the empty set to the set itself.
Q & A
What is a Venn diagram?
-A Venn diagram is a graphical representation of sets, named after English mathematician John Venn. It uses circles or other geometrical figures to represent sets and often a rectangle to represent the universal set, which contains all objects under consideration.
What is the universal set in a Venn diagram?
-The universal set in a Venn diagram is represented by a rectangle and contains all the objects under consideration. It varies depending on the objects of interest, such as a deck of cards where all 52 cards would be inside the rectangle.
How do you represent the set of vowels in the English alphabet using a Venn diagram?
-To represent the set of vowels in the English alphabet using a Venn diagram, you would draw a circle labeled 'V' inside the universal set rectangle. Inside the circle, you would place the elements 'a', 'e', 'i', 'o', and 'u'.
What is a subset in set theory?
-A set A is considered a subset of set B (denoted as A ⊆ B) if every element of set A is also contained in set B.
What is the difference between a subset and a proper subset?
-A proper subset (denoted as A ⊂ B) is a subset where all elements of A are in B, but A is not equal to B. A subset can be equal to the set it is compared to, whereas a proper subset cannot.
How can you represent a subset relationship in a Venn diagram?
-In a Venn diagram, a subset relationship can be represented by drawing a smaller circle inside a larger one, indicating that all elements of the smaller set are also elements of the larger set.
What is the cardinality of a set, and how does it relate to subsets?
-The cardinality of a set is the number of elements it contains. In relation to subsets, if a set has 'n' elements, its power set has 2^n elements, which includes all possible combinations of those elements, including the empty set and the set itself.
What is a power set, and how is it represented?
-A power set is the set of all possible subsets of another set, including the empty set and the set itself. It is denoted by P(S), where S is the original set. The power set can be represented by listing all the subsets of S.
How many subsets does a set with 'n' elements have?
-A set with 'n' elements has 2^n subsets, including the empty set and the set itself. This is derived from the fact that each element can either be included or excluded from a subset, resulting in 2 choices per element.
What is the power set of the empty set?
-The power set of the empty set contains only the empty set itself, as the empty set is the only subset of itself. Therefore, the power set of the empty set is {∅}.
What is the power set of a set containing an empty set?
-The power set of a set containing an empty set includes the empty set and the set itself. Therefore, the power set is {∅, {∅}}.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados

Union and Intersection in Venn Diagrams? | Don't Memorise

Set Operations || Mathematics in the Modern World

Representações de Conjuntos - AULA 1 - Curso de Conjuntos - Professora Angela

Algebra 3 - Venn Diagrams, Unions, and Intersections

Venn Diagrams
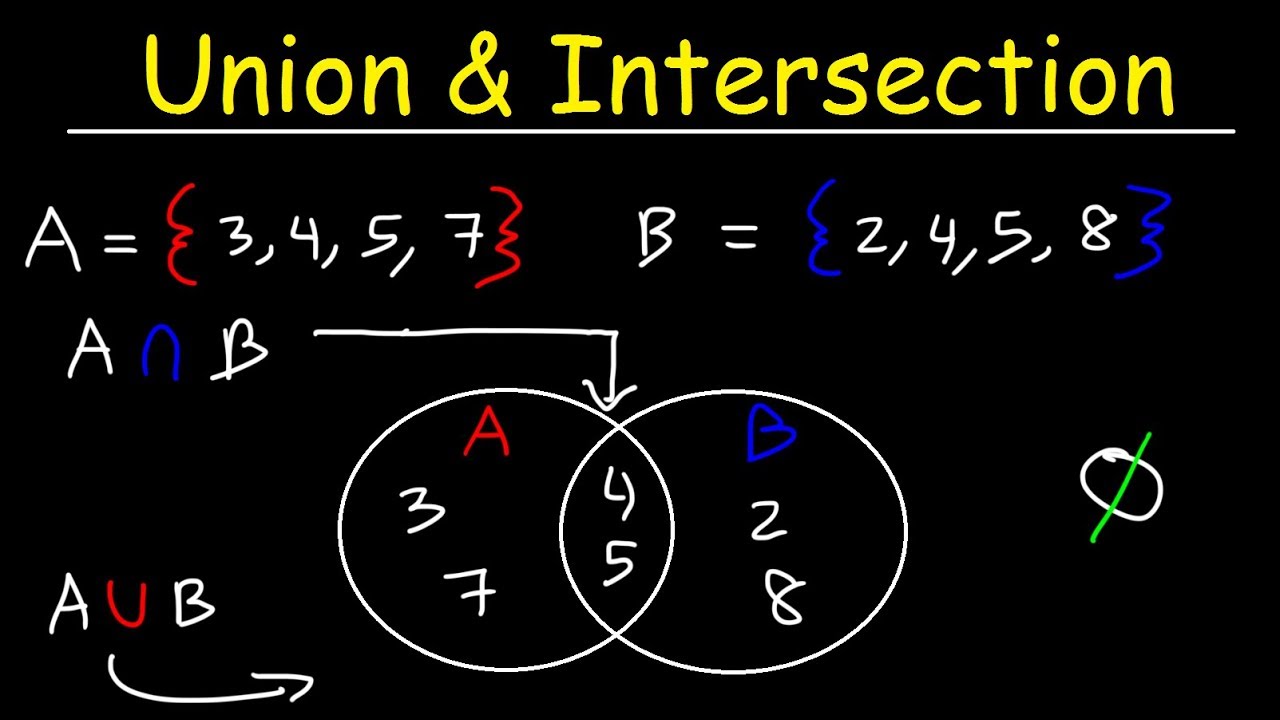
Intersection of Sets, Union of Sets and Venn Diagrams
5.0 / 5 (0 votes)
