Factoring Sums and Differences of Perfect Cubes

Summary

This educational video delves into the method of factoring expressions involving sums and differences of cubes. It introduces the formula a^3 + b^3 = (a + b)(a^2 - ab + b^2) for sums and a^3 - b^3 = (a - b)(a^2 + ab + b^2) for differences, using a and b as cube roots of the terms. Through examples like factoring x^3 + 8 and x^3 - 216, the video simplifies complex algebraic expressions, making the process accessible and emphasizing the importance of recognizing perfect cubes for effective factoring.

Takeaways

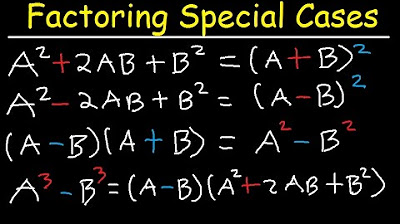
• 🔢 The formula for factoring a sum of cubes is ( a^3 + b^3 = (a + b)(a^2 - ab + b^2) ).
• 🔑 In the sum of cubes formula, a and b are the cube roots of the terms being factored.
• 📚 For example, to factor x^3 + 8, identify a = x and b = 2 (since 2^3 = 8) and apply the formula.
• ✅ Practice by factoring expressions like x^3 + 125 and 8x^3 + 27 using the sum of cubes formula.
• 🔄 The formula for factoring a difference of cubes is ( a^3 - b^3 = (a - b)(a^2 + ab + b^2) ).
• 📉 For a difference of cubes, the sign between a and b in the factored form is negative.
• 📘 Examples of difference of cubes include factoring x^3 - 216 and 64y^3 - 125.
• 🔄 The sign in the factored form of a sum or difference of cubes flips from the first term to the last.
• 💡 The script provides a generalized formula for both sum and difference of cubes, highlighting the sign changes.
• 📌 The script emphasizes the importance of recognizing perfect cubes and applying the formulas correctly.

Q & A

What is the main focus of the video?

The main focus of the video is to teach factoring sums and differences of cubes.

What is the formula for factoring a sum of cubes?

The formula for factoring a sum of cubes is \( a^3 + b^3 = (a + b)(a^2 - ab + b^2) \).

How do you determine the values of 'a' and 'b' in the sum of cubes formula?

In the sum of cubes formula, 'a' is the cube root of the first term and 'b' is the cube root of the second term.

What is an example of factoring a sum of cubes given in the video?

An example given in the video is factoring \( x^3 + 8 \), where 'a' is \( x \) and 'b' is \( 2 \), resulting in \( (x + 2)(x^2 - 2x + 4) \).

What is the formula for factoring a difference of cubes?

The formula for factoring a difference of cubes is \( a^3 - b^3 = (a - b)(a^2 + ab + b^2) \).

How do you factor the expression \( x^3 + 125 \) using the sum of cubes formula?

For the expression \( x^3 + 125 \), 'a' is \( x \) and 'b' is \( 5 \), leading to the factored form \( (x + 5)(x^2 - 5x + 25) \).

What is the process for factoring the expression \( 8x^3 + 27 \)?

For \( 8x^3 + 27 \), 'a' is \( 2x \) and 'b' is \( 3 \), resulting in the factored form \( (2x + 3)(4x^2 - 6x + 9) \).

How does the video handle the factoring of an expression that is not a perfect cube?

The video suggests replacing non-perfect cubes with the closest perfect cube and then proceeding with the factoring process.

What is the generalized formula for both sum and difference of cubes?

The generalized formula for both sum and difference of cubes is \( a^3 \pm b^3 = (a \pm b)(a^2 \mp ab + b^2) \).

Can you provide an example of factoring a difference of cubes from the video?

An example from the video is factoring \( x^3 - 216 \), where 'a' is \( x \) and 'b' is \( 6 \), resulting in \( (x - 6)(x^2 + 6x + 36) \).

How does the video explain the sign change in the generalized formula for factoring cubes?

The video explains that in the generalized formula, the sign before 'ab' changes from plus to minus for the difference of cubes and remains the same for the sum of cubes.