GEO.1.2

Summary

This educational video script delves into the concept of the segment addition postulate, a fundamental principle in geometry that allows for the calculation of the length of a line segment by adding the lengths of its constituent parts. The script explains the concept using a number line and congruent segments, introducing the congruent symbol to denote segments of equal length. It proceeds to illustrate the postulate with examples, demonstrating how to find the length of a segment by adding or subtracting the lengths of related segments. The script also covers the concept of the midpoint of a segment, which divides it into two congruent parts, and the segment bisector, a line that bisects a segment at its midpoint. Practical examples are used to solve for unknown segment lengths and midpoints, reinforcing the understanding of these geometric principles.

Takeaways

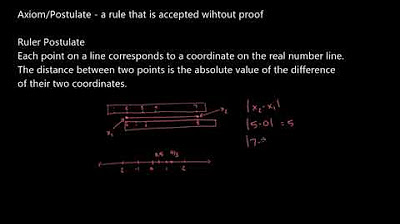
• 📏 A segment is a part of a line with a measurable length that starts and ends at two points.
• 🔢 The distance between two points is represented as the length of the segment between them, denoted as AB or |AB|.
• 📐 Congruent segments are segments of equal length, written as AB ≅ CD when AB equals CD.
• 🔄 The segment addition postulate states that if two segments are collinear, the length of the whole segment is the sum of the lengths of the parts.
• 📘 Examples demonstrate how to find the length of a segment by adding or subtracting the lengths of other segments.
• 🔢 Solving algebraic expressions involving segments involves combining like terms and balancing equations to find the unknown variable.
• 📌 The midpoint of a segment divides it into two congruent segments, meaning the segments on either side of the midpoint are equal in length.
• 📐 A segment bisector is a line that intersects a segment at its midpoint, effectively bisecting the segment into two congruent parts.
• 🔑 To find the midpoint, set the lengths of the segments on either side of the midpoint equal to each other and solve for the variable.
• 📘 Solving problems involving the midpoint often involves setting the expressions for the segments equal to each other and then solving for the unknown.

Q & A

What is a segment in geometry?

A segment in geometry is a part of a line that has a definite length, defined by two endpoints, and it ends at those points.

How is the distance between two points represented?

The distance between two points, say A and B, is represented as the length AB, or sometimes written as AB.

What does it mean for two segments to be congruent?

Two segments are congruent if they have the same length, which can be represented as AB ≅ CD if segments AB and CD are of equal length.

What is the segment addition postulate?

The segment addition postulate states that if two segments are collinear (lie on the same line), the length of the whole segment is the sum of the lengths of the parts.

How do you find the length of a segment when given the lengths of two other segments that form it?

You find the length of the whole segment by adding the lengths of the two segments that form it, as per the segment addition postulate.

What is the midpoint of a segment?

The midpoint of a segment is the point that divides the segment into two congruent segments, effectively bisecting it.

How do you determine if a point is the midpoint of a segment?

A point is the midpoint of a segment if the segments on either side of it are congruent, meaning they have equal lengths.

What is a segment bisector?

A segment bisector is a line or ray that intersects a segment at its midpoint, effectively bisecting the segment into two congruent parts.

How do you solve for the length of a segment when given the midpoint and the lengths of the segments it creates?

If a point is the midpoint of a segment, you set the lengths of the segments it creates equal to each other and solve for the unknown length.

How do you use the segment addition postulate to solve algebraic expressions involving segments?

You use the segment addition postulate to set up equations by adding or subtracting the lengths of segments, and then solve for the unknown variable.