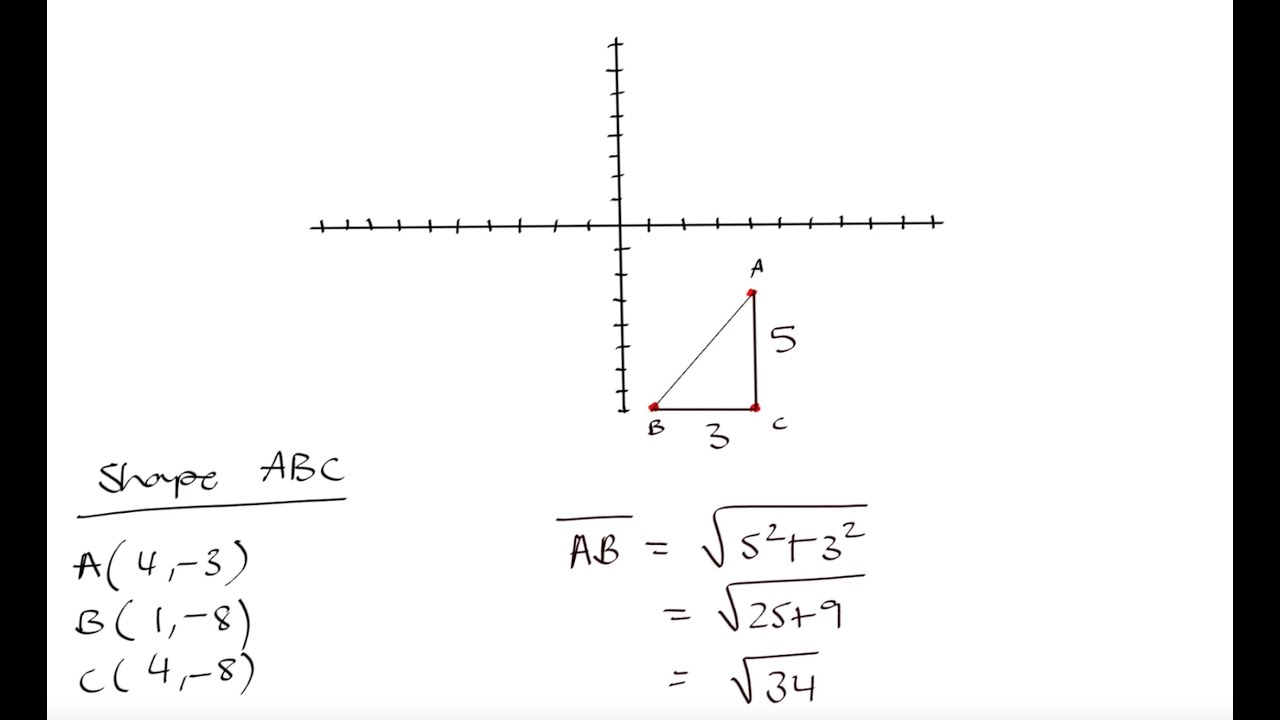Como calcular la DISTANCIA entre dos puntos en el PLANO CARTESIANO. Usando el Teorema de Pitagoras.

Summary

In this video, the process of calculating the length of a line segment in the Cartesian plane using the Pythagorean Theorem is explained step by step. The presenter demonstrates how to form a right triangle from two points on the plane, using the grid to measure the lengths of the legs (catheti) of the triangle. By applying the Pythagorean Theorem, which states that the square of the hypotenuse is equal to the sum of the squares of the other two sides, the presenter calculates the distance between the points. The tutorial concludes with the final length calculation and an invitation for viewers to subscribe.

Takeaways

• 😀 The script explains how to calculate the length of a line segment in the Cartesian plane using the Pythagorean theorem.
• 😀 To calculate the distance between two points, we construct a right triangle using the given line segment as the hypotenuse.
• 😀 The Pythagorean theorem only applies to right triangles, which is why a right angle must be formed using the line segment.
• 😀 The script uses an example to calculate the distance between the points (-2,3) and (2,-2).
• 😀 A right triangle is formed by drawing two perpendicular lines from each point to form the legs of the triangle.
• 😀 To calculate the length of the legs (catheti) of the triangle, we count the grid units on the Cartesian plane.
• 😀 In the given example, the first leg measures 5 units, and the second leg measures 4 units.
• 😀 Once the lengths of the legs are known, the Pythagorean theorem can be applied to find the length of the hypotenuse.
• 😀 The Pythagorean theorem states that the square of the hypotenuse is equal to the sum of the squares of the legs.
• 😀 Using the theorem, the hypotenuse is calculated as the square root of (25 + 16), resulting in a value of approximately 6.4 units.

Q & A

What is the main objective of this video?

The main objective of the video is to teach how to calculate the length of a line on a Cartesian plane using the Pythagorean theorem.

How does the Pythagorean theorem relate to calculating distances on the Cartesian plane?

The Pythagorean theorem helps calculate the distance between two points on the Cartesian plane by constructing a right triangle and applying the theorem to the triangle's sides.

What is required before using the Pythagorean theorem in this context?

Before using the Pythagorean theorem, it is necessary to create a right triangle by drawing two perpendicular lines from the points whose distance is being calculated.

What are the coordinates of the two points used in this example?

The two points in the example are (-2, 3) and (2, -2).

How is the right triangle constructed in this example?

The right triangle is constructed by drawing two perpendicular lines from the given points. The lines form a right angle where they meet, creating the right triangle.

Why are grid units used in this example?

Grid units are used to measure the length of the triangle's sides, making it easier to calculate the distances between points in the Cartesian plane.

What are the lengths of the legs (catheti) of the right triangle in this example?

The length of one leg is 5 units, and the length of the other leg is 4 units.

How do you calculate the hypotenuse of the right triangle in this example?

To calculate the hypotenuse, the Pythagorean theorem is applied: the square of the hypotenuse equals the sum of the squares of the legs. In this case, 5^2 + 4^2 = 25 + 16 = 41, and the hypotenuse is the square root of 41, which is approximately 6.4.

What formula is used to find the distance between two points on the Cartesian plane?

The distance formula, derived from the Pythagorean theorem, is used: distance = √[(x2 - x1)^2 + (y2 - y1)^2].

When is this method of using the Pythagorean theorem applicable?

This method is applicable when the points on the Cartesian plane have integer coordinates. If the coordinates are fractional or decimal, a different formula must be used to calculate the distance.