Introduction to Rational Function I Señor Pablo TV
Summary
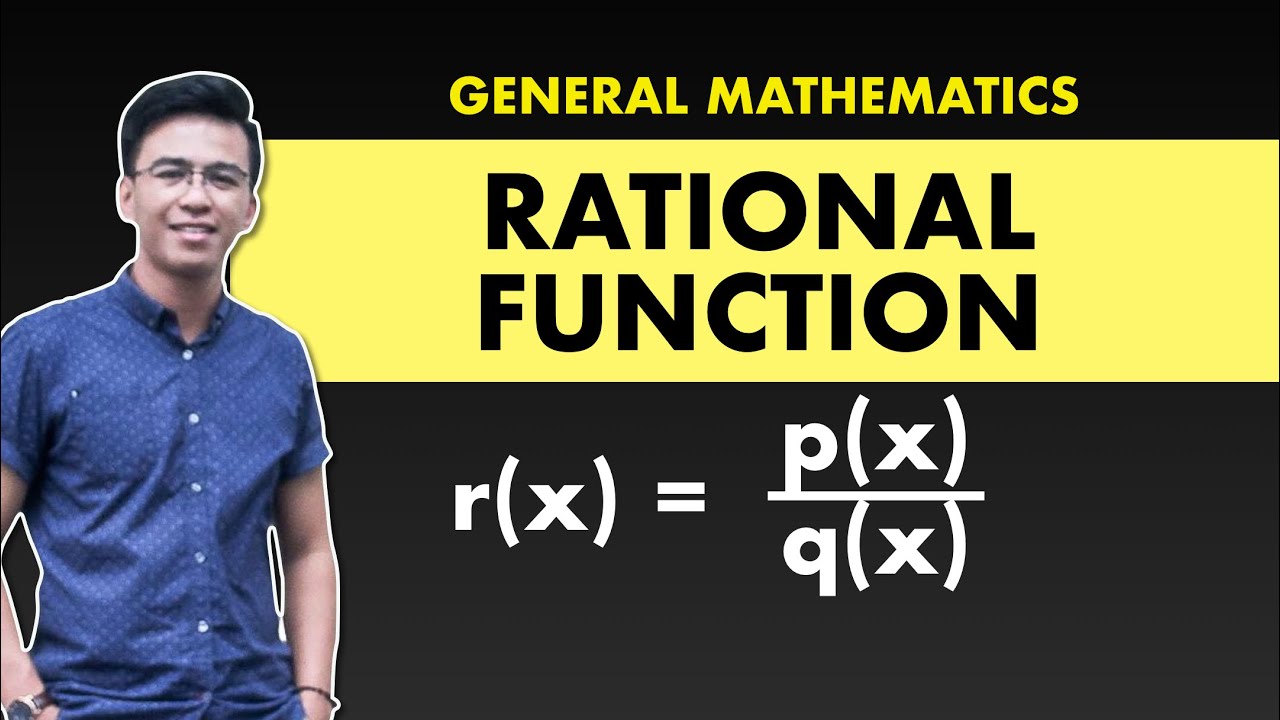
TLDRThis lesson introduces the concept of a rational function, which is a ratio of two polynomials, denoted as f(x) = p(x) / q(x), where p(x) and q(x) are polynomials and q(x) must not be zero. The video clarifies that a rational function becomes undefined when the denominator equals zero. Examples are given to distinguish between rational functions and polynomials, emphasizing the importance of the denominator in defining a rational function. The lesson concludes with a reminder to subscribe to the channel for more educational content.
Takeaways
- 📚 A rational function is represented as f(x) = p(x) / q(x), where p(x) and q(x) are polynomials.
- ⚠️ The denominator q(x) must not be equal to zero for the function to be considered rational.
- 🚫 The presence of a non-polynomial term in the denominator, such as x^(1/2), makes the function not a rational function.
- 🔍 The condition q(x) = 0 is problematic as it makes the rational function undefined.
- 📉 An example of an undefined rational function is x + 1 over 0, as the denominator is zero.
- 📈 The script provides an example of a rational expression: x^2 + 3x + 2 over 0, which is undefined due to the zero denominator.
- 🔢 The script contrasts rational functions with polynomial functions, indicating the importance of the denominator being a polynomial.
- 🤔 The script asks if x^2 + 4 over x + 3 is a rational function, implying it is conditional based on the value of x.
- 💯 The script confirms that x + 1 over x + 3 is a rational function, as the denominator is a polynomial and not zero.
- 📚 The importance of understanding the definition and conditions of rational functions is emphasized for mathematical comprehension.
- 👋 The script concludes with a reminder to subscribe to the channel for more educational content.
Q & A
What is a rational function?
-A rational function is a mathematical expression denoted by f(x) = p(x) / q(x), where p(x) and q(x) are polynomials, and q(x) must not be equal to zero.
What is the condition for a function to be considered a rational function?
-A function is a rational function if it can be expressed as the ratio of two polynomials, with the denominator not equal to zero.
What happens if the denominator of a rational function is zero?
-If the denominator of a rational function is zero, the function is undefined at that point.
Can a rational function have a non-polynomial expression in the numerator or denominator?
-No, both the numerator and the denominator of a rational function must be polynomials.
Is the expression x + 1 over zero a rational function?
-No, the expression x + 1 over zero is not a rational function because the denominator is zero, which makes the function undefined.
What is the condition for the expression x squared plus 3x plus 2 over 0 to be considered a rational expression?
-The expression x squared plus 3x plus 2 over 0 is not a rational expression because the denominator is zero, which violates the condition for a rational function.
Is the expression x raised to one half plus three over x squared a rational function?
-No, the expression x raised to one half plus three over x squared is not a rational function because the numerator contains a non-polynomial term (x to the power of 1/2).
Is the function x squared plus four over x plus three a rational function?
-Yes, the function x squared plus four over x plus three is a rational function because it is the ratio of two polynomials and the denominator is not zero.
Is the expression x plus one over x plus three a rational function?
-Yes, the expression x plus one over x plus three is a rational function, as both the numerator and the denominator are polynomials and the denominator is not zero.
What should you do if you encounter a rational function with a zero denominator?
-If a rational function has a zero denominator, you should recognize that the function is undefined at that specific value of x.
How can you determine if a given expression is a polynomial function?
-An expression is a polynomial function if it is a sum of terms with non-negative integer exponents of the variable, without any division by a variable.
What does the script suggest to do after learning about rational functions?
-The script suggests subscribing to the channel for more lessons and information.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführen5.0 / 5 (0 votes)