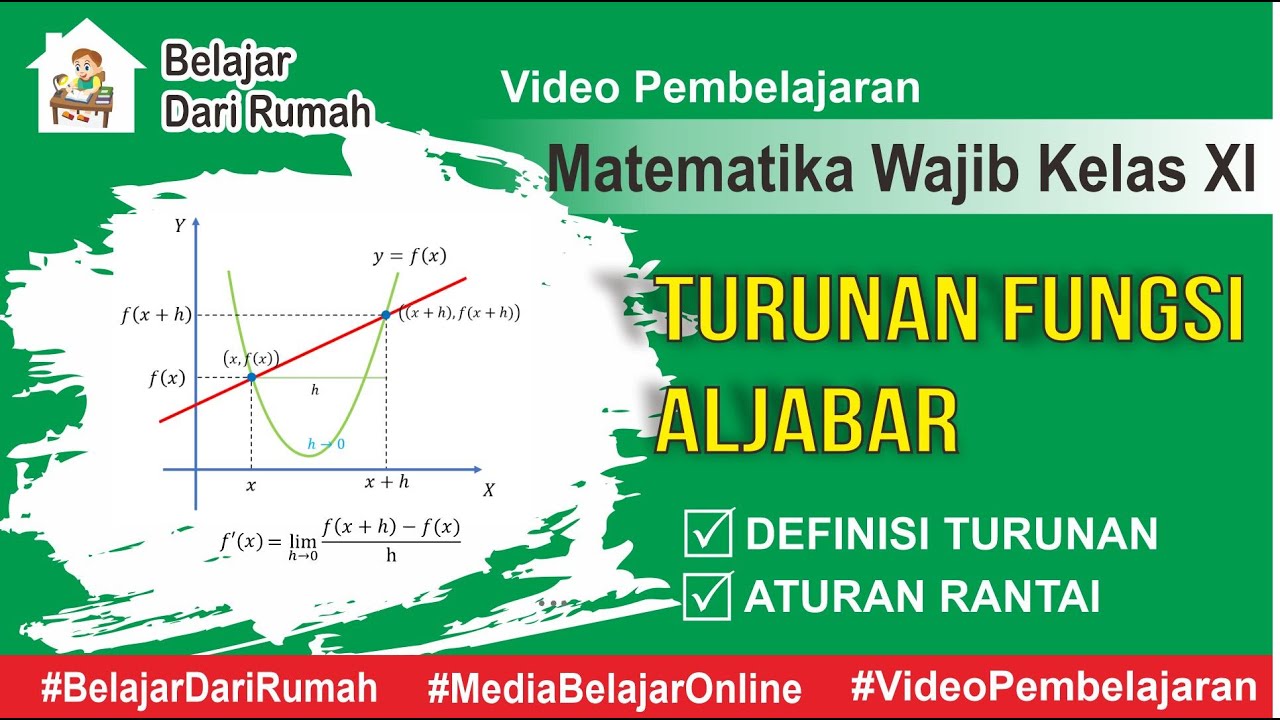
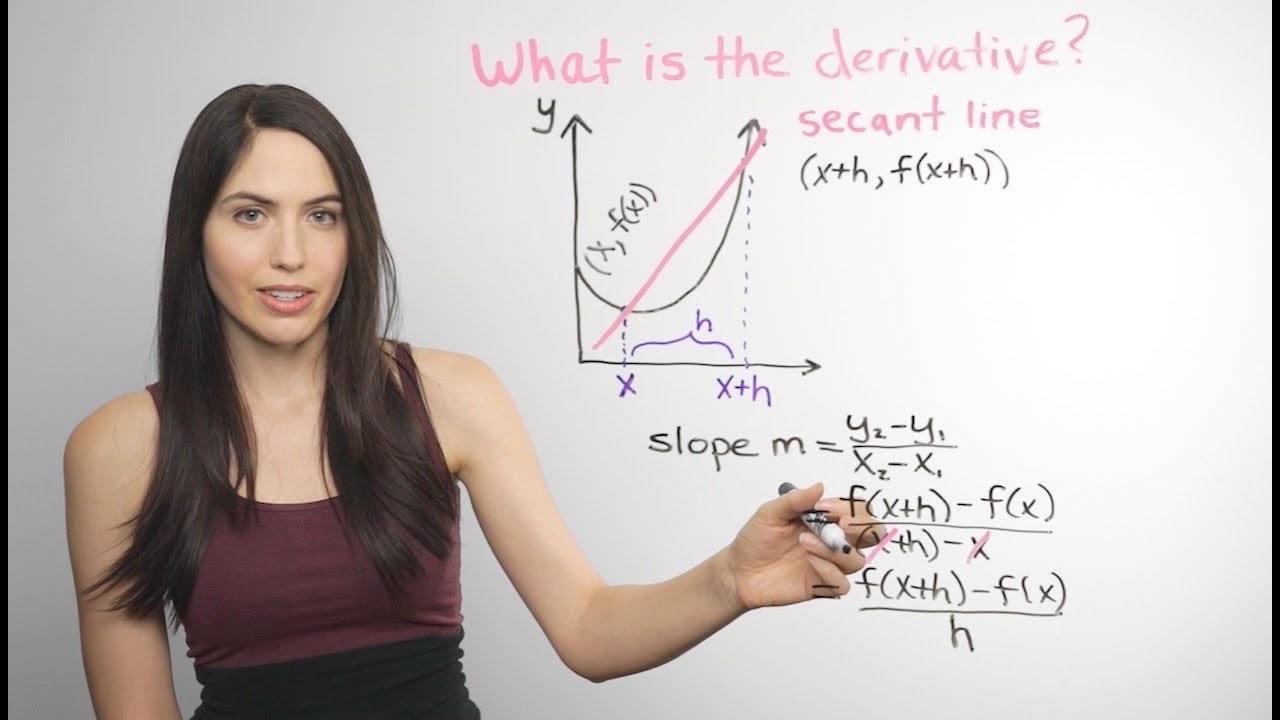Definition of the Derivative

Summary

This video explains how to find the derivative of a function using the definition of the derivative, focusing on the limit process. It demonstrates the application of the formula through several examples, including linear functions, polynomials, and rational functions. By replacing variables and simplifying expressions, viewers learn to calculate derivatives step by step. The video covers various functions such as f(x) = 5x - 4, f(x) = x², and f(x) = 1/x, ultimately illustrating that the derivative f'(x) provides crucial insights into the behavior of functions.

Takeaways

• 😀 Understanding mental states is crucial for overall health.
• 😌 Emotions can significantly influence physical well-being.
• 🧠 Awareness of one's mental state leads to better coping strategies.
• 🏋️‍♂️ Regular physical activity can improve mood and mental clarity.
• 💤 Quality sleep is essential for maintaining mental and physical health.
• 🧘‍♀️ Mindfulness practices can reduce stress and enhance mental resilience.
• 🍏 Nutrition plays a vital role in supporting mental health.
• 👥 Social connections and support are important for emotional well-being.
• 🌱 Personal development and self-care strategies can enhance mental states.
• 🩺 Professional help is available and effective for managing mental health issues.

Q & A

What is the definition of the derivative as mentioned in the video?

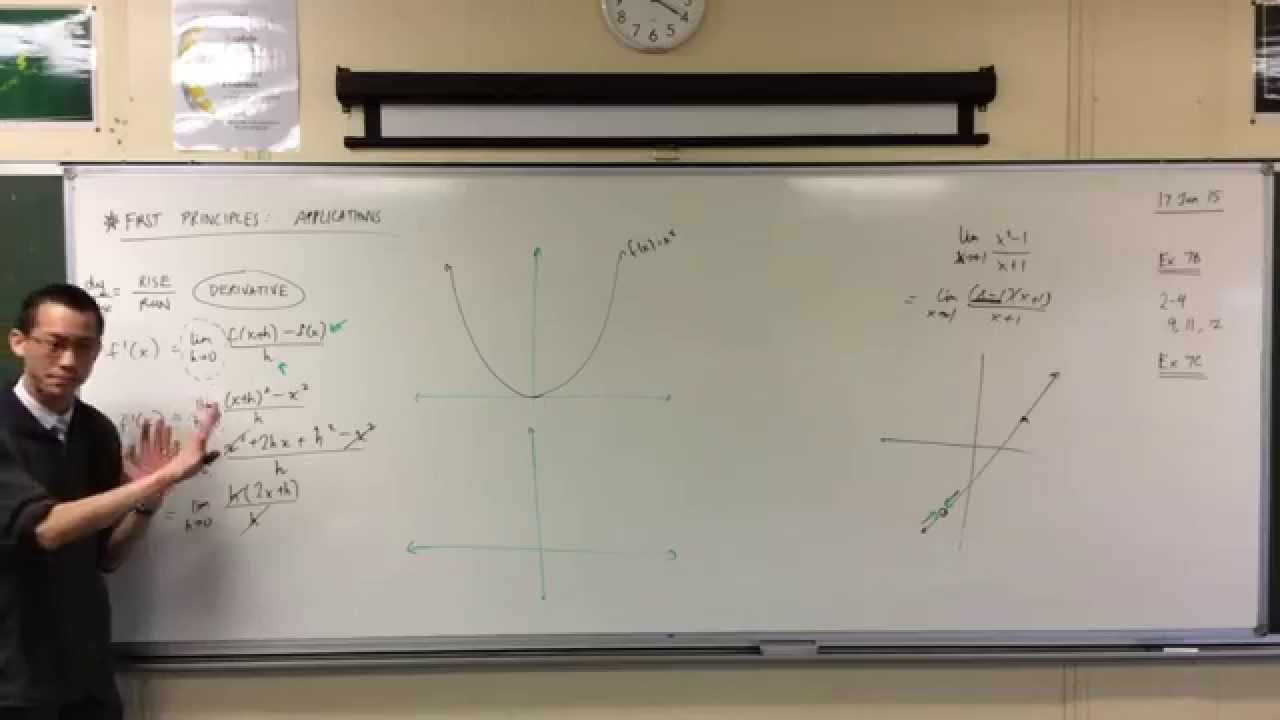
The derivative of a function, denoted as f prime of x, is defined as the limit as h approaches zero of the expression (f of x plus h minus f of x) divided by h.

How do you find f of x plus h for the function f of x = 5x - 4?

For f of x = 5x - 4, f of x plus h is found by replacing x with x plus h, resulting in f of x plus h = 5(x + h) - 4, which simplifies to 5x + 5h - 4.

What simplifications occur when finding the derivative of f of x = 5x - 4?

When calculating the derivative, the terms 5x and -5x cancel each other out, as do -4 and +4, leaving the limit as h approaches zero of 5h divided by h, which simplifies to 5.

What is the derivative of f of x = x squared?

The derivative of f of x = x squared is f prime of x = 2x, found using the limit definition and simplifying the expression.

How is the limit expressed when dealing with complex fractions?

In the case of complex fractions, one should multiply both the numerator and denominator by the common denominator to eliminate the fractions before applying the limit.

What technique is used to simplify the expression when finding the derivative of a function involving square roots?

When dealing with square roots, you multiply the numerator and the denominator by the conjugate of the numerator to simplify the expression.

What is the first derivative of f of x = 1/x?

The first derivative of f of x = 1/x is f prime of x = -1/x squared, derived by using the limit definition and simplifying the complex fraction.

What is the final result for the derivative of f of x = sqrt(x)?

The first derivative of f of x = sqrt(x) is f prime of x = 1/(2sqrt(x)), calculated by applying the limit definition and using direct substitution.

How do you approach finding the derivative of a polynomial function like f of x = x squared - 5x + 9?

For the polynomial f of x = x squared - 5x + 9, you calculate f of x plus h, expand it, apply the limit definition, simplify, and find that the derivative f prime of x = 2x - 5.

What is the derivative of f of x = 8/sqrt(x)?

The first derivative of f of x = 8/sqrt(x) is found to be f prime of x = -4/(x * sqrt(x)), derived through simplification and applying the limit process.