Pengertian Fungsi - Matematika Kelas XI Kurikulum Merdeka
Summary
TLDRThis educational video script delves into the concepts of 'relation' and 'function' in the context of high school mathematics. It explains the definition of a relation as a rule connecting members of one set to another, using diagrams such as arrows, ordered pairs, and Cartesian diagrams for illustration. The script then clarifies that a function is a specific type of relation where each member of one set is connected to exactly one member of another set. The video provides examples, including a real-life analogy of a blender making orange juice, to distinguish between relations and functions, emphasizing the uniqueness and specificity of functions in mathematical terms.
Takeaways
- 📚 The video is a lesson on the concept of 'relation' and 'function' in mathematics, specifically for 11th-grade students in the odd semester.
- 🔗 The lesson starts by explaining the concept of 'relation', which is a rule that connects elements from one set to another, and can be represented using diagrams, ordered pairs, or Cartesian diagrams.
- 📈 The video uses diagrams of arrows, ordered pairs, and Cartesian diagrams to illustrate the concept of relations between two sets.
- 🔑 The script emphasizes the difference between a 'relation' and a 'function', stating that a function is a specific type of relation where each element of one set is connected to exactly one element of another set.
- 📝 The function is usually represented in the form of 'f(x) = y', where 'f' is the function, 'x' is the input variable, and 'y' is the output variable.
- 💡 An example given in the video to explain functions is using a blender to make orange juice, where the oranges are the input and the juice is the output.
- 👉 The video script provides a clear definition of a function and explains that for a relation to be a function, it must meet certain criteria: it must be specific and not arbitrary, with each input having exactly one output.
- 📉 The script uses three diagrams to illustrate the difference between relations and functions, analyzing whether each diagram represents a function based on the criteria discussed.
- ✅ The first diagram with elements A, B, C from set X and 1, 2, 3 from set Y, where each element has a unique pair, is identified as both a relation and a function.
- ❌ The second diagram, where one element from set X does not have a pair in set Y, is identified as a relation but not a function because it does not meet the criteria of having a pair for each element.
- ❗ The third diagram, where an element from set X has more than one pair in set Y, is also identified as a relation but not a function, as it violates the 'one-to-one' pairing rule of functions.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is the concept of relations and functions in mathematics, particularly in the context of high school education.
What is a relation in mathematics?
-A relation in mathematics is a rule that connects members of one set to another set. It can be represented in three ways: through an arrow diagram, an ordered pair set, or a Cartesian diagram.
How is a relation typically represented in an arrow diagram?
-In an arrow diagram, a relation is represented by arrows pointing from elements of one set to elements of another set, indicating the connections between the two sets.
What is an ordered pair set in the context of relations?
-An ordered pair set is a way to represent a relation by listing pairs of elements from two sets, where the first element of each pair is from the first set and the second is from the second set.
How is a relation depicted in a Cartesian diagram?
-In a Cartesian diagram, a relation is depicted by plotting points on the coordinate plane, where the x-axis represents the first set and the y-axis represents the second set, and each point represents a pair in the relation.
What is the difference between a relation and a function?
-While both are types of relations, a function is a specific type of relation where each element of the first set is connected to exactly one element of the second set, following a set rule and not randomly.
How is a function typically represented in mathematical notation?
-A function is typically represented as f(x) = y, where 'f' denotes the function, 'x' is the input variable, and 'y' is the output variable.
Can you give an example of a function from everyday life mentioned in the script?
-An example from everyday life mentioned in the script is a blender. If you put oranges (input) into a blender, it produces orange juice (output), which is a function because each input (orange) results in a specific output (orange juice).
What are the criteria that must be met for a relation to be considered a function?
-For a relation to be considered a function, it must meet the criteria that each element in the first set has exactly one corresponding element in the second set, ensuring a one-to-one correspondence.
How can you determine if a relation in a Cartesian diagram is a function or not?
-You can determine if a relation in a Cartesian diagram is a function by checking if each point on the x-axis (first set) corresponds to exactly one point on the y-axis (second set), without any repetition or missing elements.
What is the significance of the terms 'setia' (faithful), 'jomblo' (single), and 'selingkuh' (cheating) used in the script to describe functions?
-These terms are used metaphorically to describe the properties of functions: 'setia' means that each input has only one output (faithfulness), 'jomblo' indicates that there should be no element in the first set without a corresponding output (not single), and 'selingkuh' implies that an element should not have more than one corresponding output (not cheating).
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
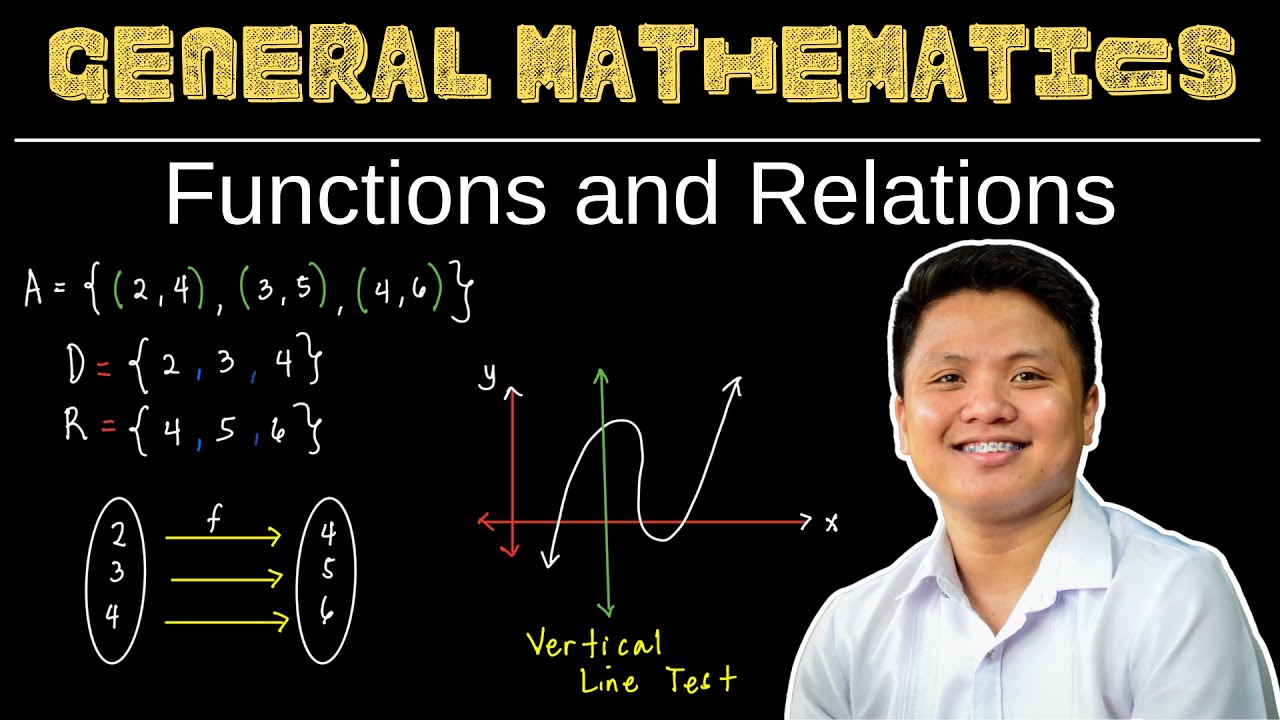
Relations and Functions | General Mathematics | Grade 11
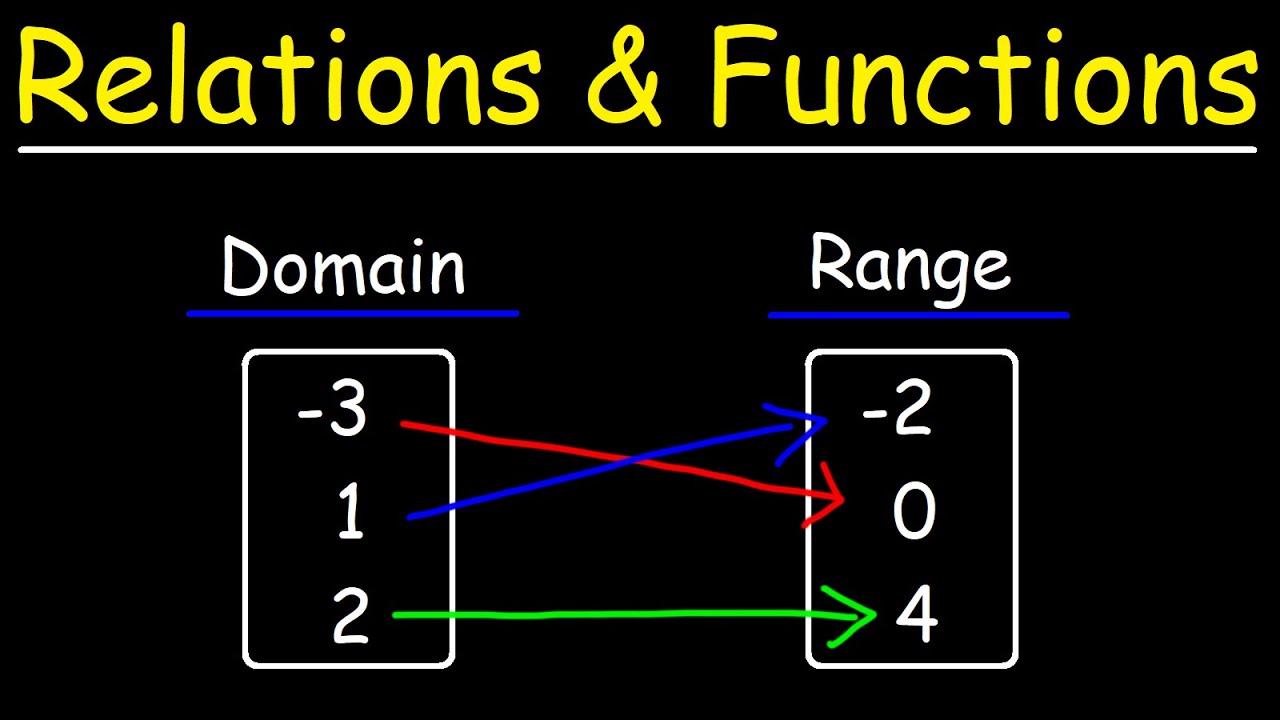
Relations and Functions | Algebra

Turunan (Diferensial) Aturan berantai

Pensamiento Matemático II PROGRESION 10

RELASI DAN FUNGSI | DOMAIN, KODOMAIN, RANGE - Matematika Kelas 8 SMP
FUNCTIONS | SHS GRADE 11 GENERAL MATHEMATICS QUARTER 1 MODULE 1 LESSON 1
5.0 / 5 (0 votes)
