TRIGONOMETRI #3 (perbandingan trigonometri di berbagai kuadran)
Summary
TLDRThis video tutorial covers trigonometric ratios in all four quadrants, providing a comprehensive understanding of how sine, cosine, tangent, secant, cosecant, and cotangent behave in each quadrant. It explains the rules for determining the signs of these functions depending on the quadrant, using reference angles and key identities to simplify calculations. The video includes detailed examples to demonstrate how to find values for various angles, helping viewers master trigonometric functions and their relationships in different quadrants. The content aims to make learning trigonometry easier and more accessible.
Takeaways
- 😀 Quadrants are crucial for understanding trigonometric functions. There are four quadrants, each with specific rules about which functions are positive.
- 😀 In Quadrant 1 (0° to 90°), all trigonometric functions (sin, cos, tan, sec, csc, cot) are positive.
- 😀 In Quadrant 2 (90° to 180°), only sine (sin) and cosecant (csc) are positive, while the others (cos, tan, sec, cot) are negative.
- 😀 In Quadrant 3 (180° to 270°), tangent (tan) and cotangent (cot) are positive, with the others being negative.
- 😀 In Quadrant 4 (270° to 360°), cosine (cos) and secant (sec) are positive, while sine (sin), tangent (tan), and cotangent (cot) are negative.
- 😀 Important trigonometric identities include Sin(90° - α) = Cos(α), Cos(90° - α) = Sin(α), and Tan(90° - α) = Cot(α).
- 😀 Trigonometric values for special angles (e.g., 30°, 45°, 60°, 90°) are fundamental for solving problems across quadrants.
- 😀 To solve problems in different quadrants, use reference angles and quadrant rules (e.g., 90° + angle, 180° - angle) to find the correct trigonometric value.
- 😀 The tutorial includes example problems to demonstrate how to apply trigonometric identities and transformations to angles in different quadrants.
- 😀 It is helpful to memorize the trigonometric values for Quadrant 1 since these values form the basis for calculating values in the other quadrants.
Q & A
What is the importance of understanding trigonometric functions in different quadrants?
-Understanding trigonometric functions in different quadrants is crucial because each quadrant has specific sign conventions for the values of sine, cosine, tangent, and their reciprocals. This knowledge allows you to determine the correct values for trigonometric functions based on the angle's location in the unit circle.
How are the four quadrants defined in terms of angle ranges?
-The four quadrants are defined by the following angle ranges: Quadrant 1 (0° to 90°), Quadrant 2 (90° to 180°), Quadrant 3 (180° to 270°), and Quadrant 4 (270° to 360°). These ranges help identify the sign of trigonometric functions in each quadrant.
Which trigonometric functions are positive in Quadrant 1?
-In Quadrant 1, all trigonometric functions (sine, cosine, tangent, secant, cosecant, and cotangent) are positive.
In Quadrant 2, which trigonometric functions are positive?
-In Quadrant 2, only sine and cosecant are positive, while cosine, tangent, secant, and cotangent are negative.
What is the relationship between sine and cosine in the first quadrant?
-In the first quadrant, sine and cosine have specific equalities, such as sin 0° = cos 90°, sin 30° = cos 60°, sin 45° = cos 45°, and so on. This relationship is critical when transforming sine to cosine and vice versa.
How do you determine the value of sin 120° using reference angles?
-To determine sin 120°, you can use the reference angle 30° (since 120° = 90° + 30°). Because sine is positive in Quadrant 2, sin 120° equals cos 30°, which is √3/2.
What are the key formulas used for transforming trigonometric functions between sine and cosine?
-The key formulas are: sin α = cos (90° - α) and cos α = sin (90° - α). These formulas allow you to convert between sine and cosine by adjusting the angle.
In Quadrant 3, which trigonometric function is positive?
-In Quadrant 3, only tangent and cotangent are positive, while sine and cosine are negative.
What is the method to solve for cos 135°?
-To solve for cos 135°, you can use the reference angle 45° (since 135° = 90° + 45°). Because cosine is negative in Quadrant 2, the value of cos 135° is -√2/2.
How do you solve sin 315° using reference angles?
-To solve sin 315°, you can use the reference angle 45° (since 315° = 270° + 45°). Since sine is negative in Quadrant 4, sin 315° is -√2/2.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
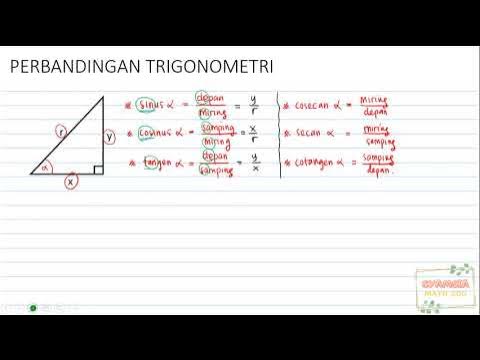
PERBANDINGAN TRIGONOMETRI (PART 1) SINUS, COSINUS, TANGEN, SECAN, COSECAN, COTANGEN

Identitas Trigonometri - Part 1

Trigonometric Ratios (Tagalog Math)

TRIGONOMETRI ( PERBANDINGAN TRIGONOMETRI DLM SEGITIGA SIKU SIKU & SUDUT SUDUT ISTIMEWA )

ILLUSTRATING CIRCULAR FUNCTIONS || PRE-CALCULUS
ENTENDIENDO realmente QUÉ son SEN, COS y TAN ▶ ¿De DONDE PROVIENEN las RAZONES TRIGONOMÉTRICAS? 📐📖
5.0 / 5 (0 votes)
