ENTENDIENDO realmente QUÉ son SEN, COS y TAN ▶ ¿De DONDE PROVIENEN las RAZONES TRIGONOMÉTRICAS? 📐📖
Summary
TLDRThis video introduces the fundamental concept of trigonometric ratios, highlighting their significance in science, physics, and various mathematical equations. It explains how trigonometric ratios are derived from right triangles and the relationships between their sides. Through examples, the video explores the six key trigonometric functions—sine, cosine, tangent, cotangent, secant, and cosecant—and their applications in solving geometric problems, calculating distances indirectly, and understanding wave phenomena. The video concludes by emphasizing the wide-reaching applications of trigonometry in fields such as engineering, astronomy, and music, demonstrating the practical importance of these mathematical tools.
Takeaways
- 😀 Trigonometry is a crucial mathematical tool used to calculate angles and distances indirectly, which has broad applications in science, physics, and engineering.
- 😀 The constant pi (π) is derived from the ratio of the circumference's perimeter to its diameter, approximately equal to 3.14, and this is essential for understanding circles.
- 😀 A right triangle consists of three sides: the hypotenuse (largest side), and the other two sides, called catheti. The ratio of these sides forms the basis for trigonometric functions.
- 😀 By maintaining a constant angle in a right triangle, the ratio of the sides (such as opposite, adjacent, and hypotenuse) remains consistent.
- 😀 The six main trigonometric functions are: sine (sin), cosine (cos), tangent (tan), cotangent (cot), secant (sec), and cosecant (csc), each defined by a specific ratio of triangle sides.
- 😀 Trigonometric functions are defined in relation to the angle of a right triangle, with each function comparing different sides (opposite, adjacent, hypotenuse) to one another.
- 😀 The mnemonic 'SOH-CAH-TOA' helps students remember trigonometric definitions: Sine = Opposite/Hypotenuse, Cosine = Adjacent/Hypotenuse, Tangent = Opposite/Adjacent.
- 😀 Trigonometric ratios are crucial for solving real-world problems such as calculating heights and distances indirectly, such as measuring the height of the Eiffel Tower using angles.
- 😀 Each trigonometric function can be expressed as a reciprocal of another, such as the cosecant being the reciprocal of sine, and secant being the reciprocal of cosine.
- 😀 Trigonometric functions extend beyond acute angles, allowing for calculations with angles larger than 90 degrees, negative angles, and even extending into applications like Fourier series and solving differential equations.
Q & A
What are the six trigonometric ratios discussed in the video?
-The six trigonometric ratios are sine, cosine, tangent, cotangent, secant, and cosecant.
How is the number Pi related to the circumference of a circle?
-Pi is the ratio of the circumference of a circle to its diameter. This ratio is always constant and approximately equals 3.14.
What is the significance of the triangle with sides 3, 4, and 5 units in the video?
-The triangle with sides 3, 4, and 5 is used to demonstrate how the ratios between the sides of a right triangle remain consistent when the angles are fixed.
What is the importance of keeping the angle constant in a right triangle when calculating trigonometric ratios?
-When the angle remains constant, the trigonometric ratio between the sides of the triangle also remains constant, regardless of how the side lengths change.
What mnemonic is used to remember the basic trigonometric ratios, and what does it stand for?
-The mnemonic 'SOH-CAH-TOA' is used to remember the basic trigonometric ratios: Sine = Opposite/Hypotenuse, Cosine = Adjacent/Hypotenuse, Tangent = Opposite/Adjacent.
How are the reciprocal trigonometric ratios related to the basic trigonometric ratios?
-The reciprocal trigonometric ratios are related as follows: cosecant is the reciprocal of sine, secant is the reciprocal of cosine, and cotangent is the reciprocal of tangent.
What is the role of trigonometry in physics and other scientific fields?
-Trigonometry plays a key role in physics and science, allowing the calculation of angles, distances, and understanding of wave phenomena, including electromagnetic waves and sound waves.
Why is the value of pi significant in the calculation of the perimeter of a circle?
-Pi is significant because it defines the relationship between the circumference and the diameter of a circle, making it possible to calculate the perimeter using the formula C = 2πr.
How do trigonometric ratios help in indirect measurement of distances?
-Trigonometric ratios allow us to calculate unknown distances indirectly by using known angles and side lengths in right triangles, as shown in the example of calculating the height of the Eiffel Tower.
What is the general definition of a trigonometric ratio in a right triangle?
-A trigonometric ratio is the value obtained by comparing two sides of a right triangle with respect to one of its acute angles, such as the ratio of the opposite side to the hypotenuse for sine.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

IDENTITAS TRIGONOMETRI

Trigonometric Ratios (Tagalog Math)

Konsep Dasar dan Sifat-sifat Logaritma Matematika Peminatan Kelas 10
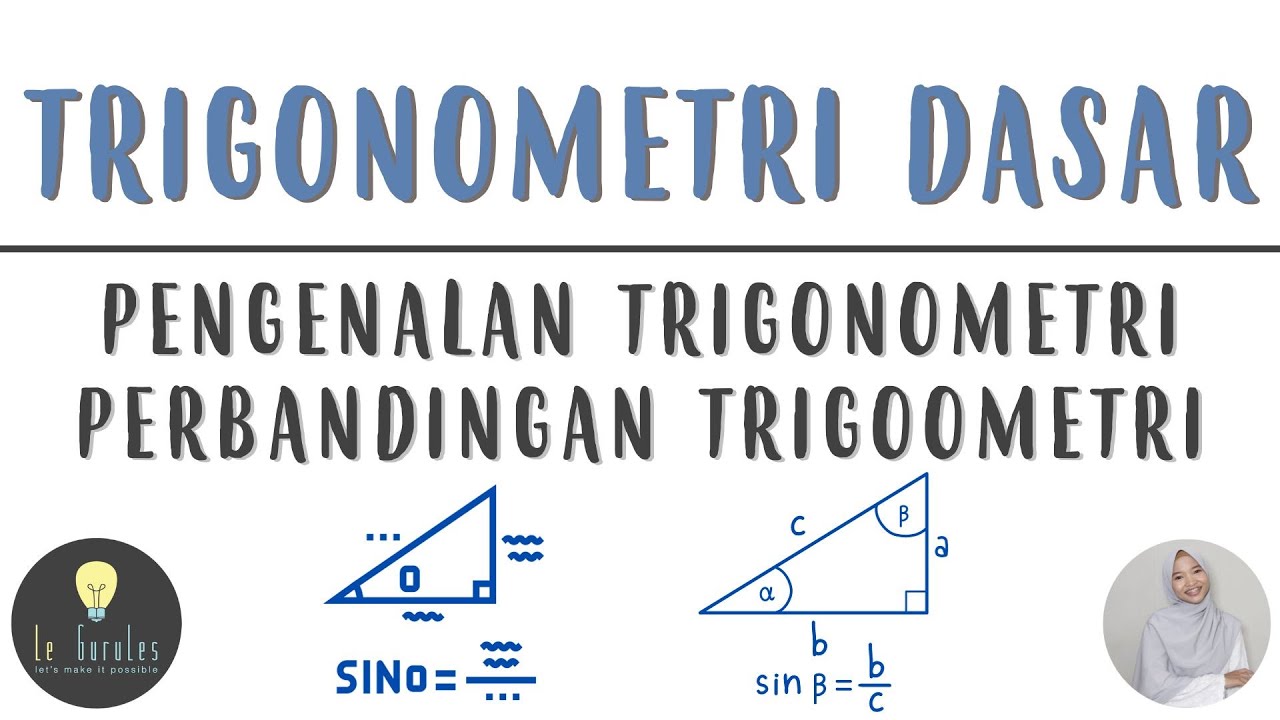
Matematika SMA - Trigonometri (1) - Pengenalan Trigonometri, Perbandingan Trigonometri (A)

10 класс. Алгебра. Тригонометрические уравнения, решаемые разложением левой части на множители

Pengantar Persamaan Diferensial
5.0 / 5 (0 votes)