Bernoulli's Equation for Fluid Flow Video in Physics

Summary

This video provides a clear and intuitive derivation of Bernoulli's equation for fluid flow. It explains the fundamental assumptions of ideal fluids—such as incompressibility, constant density, and steady flow—while introducing the continuity equation, which links fluid velocity and pipe cross-sectional area. The work-energy theorem is then applied to explain how pressure differences lead to changes in the fluid's kinetic and potential energy. Ultimately, the video leads to the derivation of Bernoulli’s equation, offering insights into the relationship between pressure, velocity, and height in a fluid system.

Takeaways

• 😀 The video aims to provide an intuitive understanding of Bernoulli's equation for fluid flow.
• 😀 The fluid is assumed to be ideal: incompressible, with constant density, steady flow, and no viscosity.
• 😀 The volume flow rate, Q, is constant in the system, meaning the product of pipe area and fluid velocity at any point must also be constant (continuity equation).
• 😀 As the pipe narrows, fluid velocity increases, implying a difference in pressure between wider and narrower sections.
• 😀 The pressure difference causes acceleration in the fluid, which in turn requires work to be done on the fluid, altering its energy.
• 😀 The Work-Energy Theorem is applied to relate the work done by pressures to changes in kinetic and potential energy of the fluid.
• 😀 The work done by pressure p1 (behind the fluid) is positive, while the work by p2 (in front) is negative.
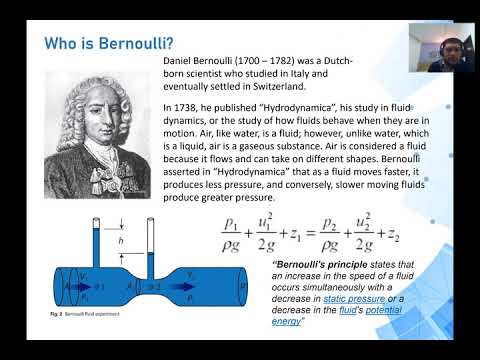
• 😀 The equation combines work terms, changes in kinetic energy, and potential energy, leading to Bernoulli's equation: p1 + 1/2 rho v1^2 + rho g y1 = p2 + 1/2 rho v2^2 + rho g y2.
• 😀 The terms in Bernoulli's equation correspond to pressure, kinetic energy (1/2 rho v^2), and potential energy (rho g y) at different points in the fluid.
• 😀 Bernoulli's equation can be tricky in problems, so key tips include using the continuity equation to solve for unknown velocities and considering only changes in height for gravitational potential energy.

Q & A

What does it mean when a fluid is described as 'ideal' in the context of Bernoulli's equation?

An ideal fluid is incompressible, meaning its density is constant, and it has no viscosity, which means it has no internal friction. This simplifies the analysis of fluid flow, as these properties allow the fluid to maintain a steady flow without energy losses due to friction or changes in density.

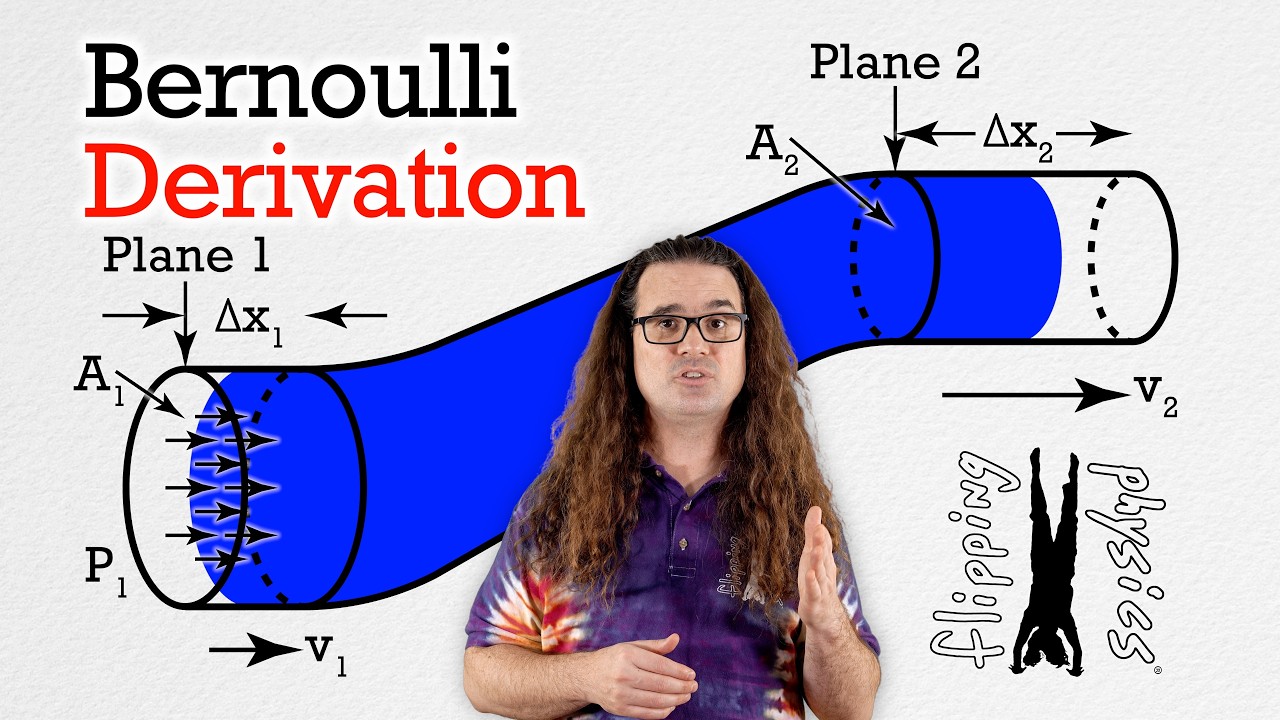
What is the continuity equation, and why is it important in the derivation of Bernoulli's equation?

The continuity equation states that the volume flow rate of an incompressible fluid is constant along a pipe, meaning the product of the cross-sectional area and the fluid velocity (A × V) must remain the same at all points. It is crucial for understanding how fluid velocity changes when the pipe's area changes, which leads to pressure differences as the fluid accelerates.

What happens when the cross-sectional area of a pipe decreases in terms of fluid velocity?

When the cross-sectional area of the pipe decreases, the velocity of the fluid must increase in order to maintain a constant volume flow rate, as dictated by the continuity equation.

How does the change in fluid velocity relate to pressure differences in a pipe?

A change in fluid velocity (such as when the pipe narrows) indicates acceleration, which requires a force. This force is created by pressure differences in the fluid: the pressure behind the fluid (P1) must be greater than the pressure in front of it (P2) to push the fluid forward.

What is the work-energy theorem, and how is it applied in this context?

The work-energy theorem states that the work done on a system is equal to its change in kinetic and potential energy. In the context of fluid flow, the work done by pressure differences (P1 and P2) results in changes in the kinetic energy (due to changes in speed) and potential energy (due to changes in height) of the fluid.

What does the term 'rho' represent in the derivation of Bernoulli's equation?

'Rho' (ρ) represents the density of the fluid. It is a constant for incompressible fluids and is used to express mass in terms of volume in the energy equations for kinetic and potential energy.

How do changes in the height of a fluid affect its potential energy?

The gravitational potential energy of the fluid is related to its height (y). Changes in height between two points in the fluid flow contribute to the change in potential energy, expressed as ρg(y2 - y1), where g is the gravitational acceleration.

What happens to the volume of fluid as it moves through a pipe with constant flow rate?

The volume of the fluid remains constant as it flows through the pipe because the fluid is incompressible. This is consistent with the continuity equation, which ensures that the mass and volume of the fluid are conserved at every point in the flow.

What is the final form of Bernoulli's equation for fluid flow?

The final form of Bernoulli's equation is: P1 + (1/2)ρv1² + ρgy1 = P2 + (1/2)ρv2² + ρgy2, where P1 and P2 are pressures at two points, v1 and v2 are fluid velocities, and y1 and y2 are heights of the fluid at those points.

How can Bernoulli's equation be used to solve fluid flow problems?

Bernoulli's equation can be used to solve problems involving fluid flow by relating the pressures, velocities, and heights at two points along the flow. For example, if one of the speeds (v1 or v2) is unknown, the continuity equation can be used to find it. In cases where the height difference is not given, it's often useful to define the height at one point as zero to simplify the calculations.